Recurrence-Based Representations of the Logistic Map
Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
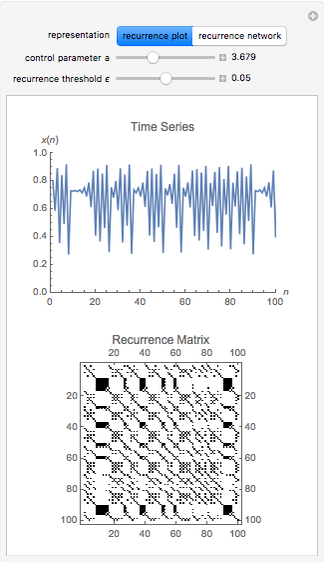
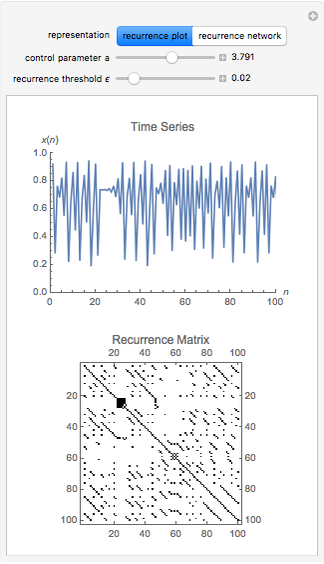
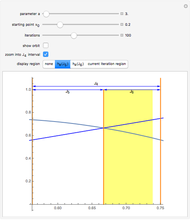
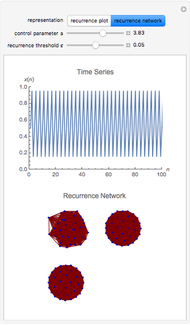
Recurrence plots and recurrence networks are complementary representations of the recurrence properties of a time series. Every time step constitutes a node of the recurrence network. A recurrence in phase space is either represented by a link in the recurrence network or as a black dot in the recurrence plot. Two time steps are recurrent if and only if their mutual distance is smaller than a threshold 
.
Contributed by: Alraune Zech, Jonathan F. Donges, Norbert Marwan, and Jürgen Kurths (February 2010)
Open content licensed under CC BY-NC-SA
Snapshots
Details
A recurrence network (undirected and unweighted) is represented by a binary adjacency matrix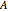 
, where 
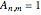if nodes 
and 
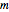are linked, and 
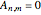otherwise. Two nodes are considered to be linked when their mutual distance is smaller than 
The adjacency matrix is then given by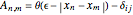 
, where 
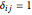if 
and 
otherwise.
The adjacency matrix is related to the recurrence matrix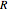 
representing a recurrence plot (RP) by 
.
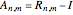The recurrence network view allows us to apply the methods and measures of complex network theory to time series analysis. It is complementary to the traditional recurrence quantification analysis based on line structures in recurrence plots.
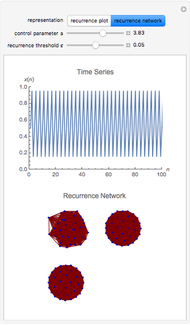
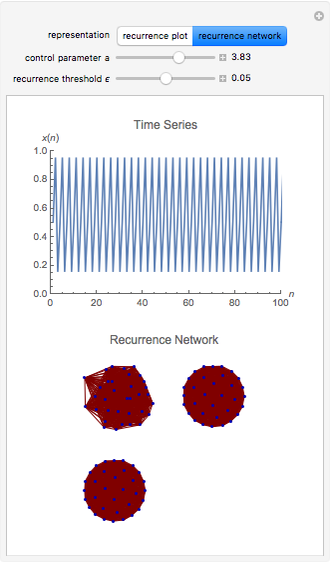
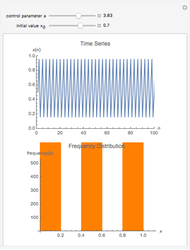
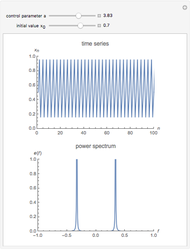
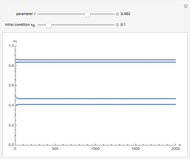
Snapshot 1 (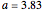
): in the periodic regime, with period length 
, the phase space consists of three (nearly) fully connected network components
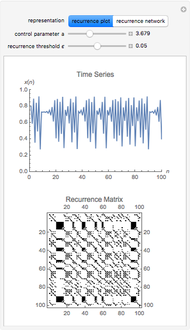
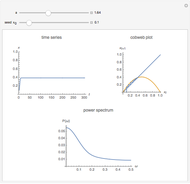
Snapshot 2 (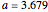
): at the band-merging point, the RP reveals extended clusters of recurrence points, which correspond to many laminar phases; moreover, several diagonal lines appear, showing short epochs of similar evolution of states
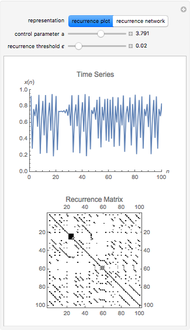
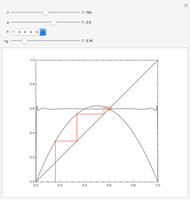
Snapshot 3 (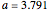
): the RP for laminar states also consists of extended clusters, but possesses more diagonal lines
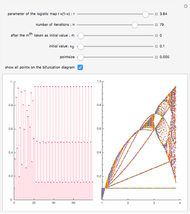
Snapshot 4 (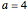
): for the outer crisis a giant component still exists, but the nodes are less tightly clustered than in the previous snapshots
This Demonstration was created during an internship at the Potsdam Institute for Climate Impact Research, Germany. It is based on the articles:
[1] N. Marwan, J. F. Donges, Y. Zou, R. V. Donner, and J. Kurths, "Complex Network Approach for Recurrence Analysis of Time Series," Physics Letters A, 373(46), 2009 pp. 4246–4254.
[2] R. V. Donner, Y. Zou, J. F. Donges, N. Marwan, and J. Kurths, "Recurrence Networks - A Novel Paradigm for Nonlinear Time Series Analysis," arXiv, 2009.
[3] R. V. Donner, Y. Zou, J. F. Donges, N. Marwan, and J. Kurths, "Ambiguities in Recurrence-Based Complex Network Representations of Time Series," Physical Review E (R), 2010.
[4] N. Marwan, N. Wessel, U. Meyerfeldt, A. Schirdewan, and J. Kurths, "Recurrence-Plot-Based Measures of Complexity and Their Application to Heart-Rate-Variability Data," Physical Review E, 66(2), 2002.
[5] N. Marwan, M. C. Romano, M. Thiel, and J. Kurths, "Recurrence Plots for the Analysis of Complex Systems," Physics Reports, 438(5–6), 2007 pp. 237–329.
Further information can be found at Recurrence Plots and Cross Recurrence Plots.
Permanent Citation
Recurrence Network Measures for the Logistic Map
Alraune Zech, Jonathan F. Donges, Norbert Marwan, and Jürgen Kurths
Frequency Distribution of the Logistic Map
Alraune Zech, Jonathan F. Donges, Norbert Marwan, and Jürgen Kurths
Power Spectrum of the Logistic Map
Alraune Zech, Jonathan F. Donges, Norbert Marwan, and Jürgen Kurths
Three Views of the Logistic Map
Hiroki Sayama
Trajectories of the Logistic Map
Michael Trott
Iterates, Cycles, and Bifurcations of the Logistic Map
Bernard Vuilleumier
Bifurcations of the Logistic Map
Rob Morris
Computational Complexity of the Logistic Map
Christoph Spandl
An Interval Eventually Bounding Trajectories of the Logistic Map
Lesinigo Matteo
A Special Parameter Value for the Logistic Map
Lesinigo Matteo
