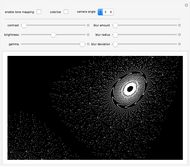
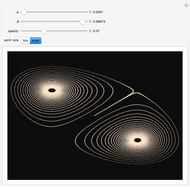
Attraction and Repulsion in Dynamical Systems

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
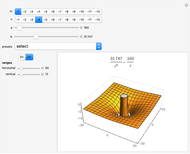
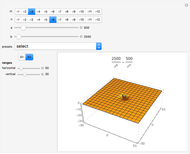
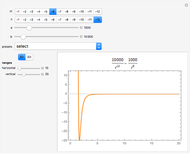
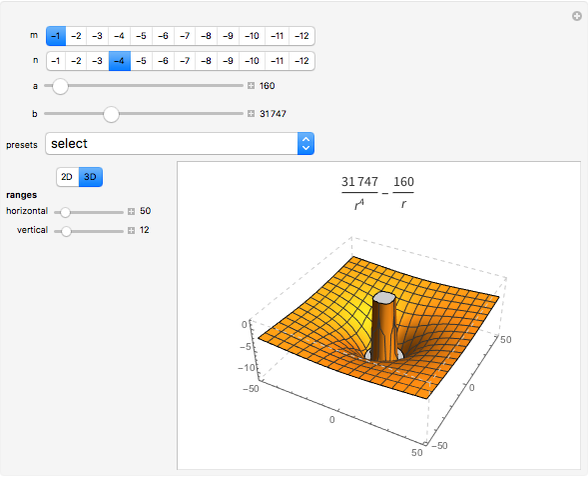
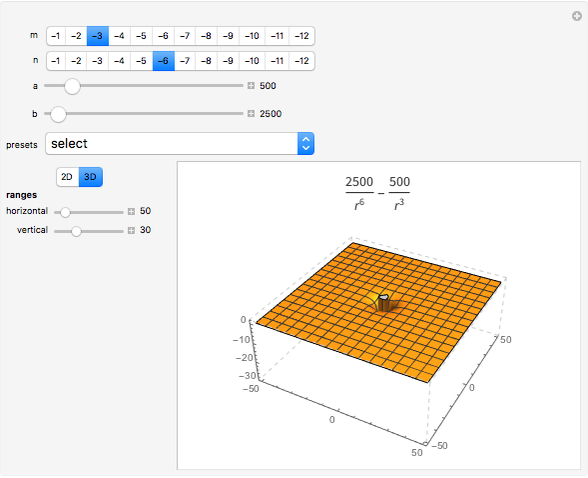
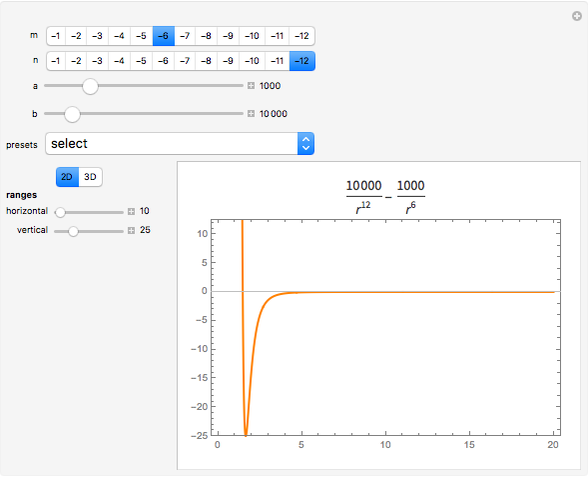
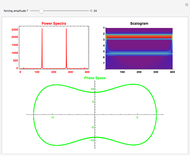
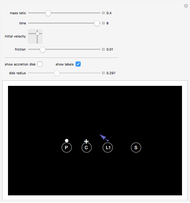
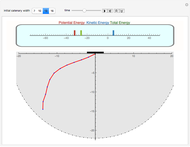
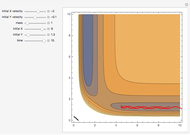
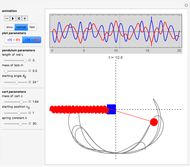
This Demonstration simulates attraction and repulsion in dynamical systems, described by a potential function 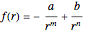 , with
, with  being the relevant distance parameter. When applied to
being the relevant distance parameter. When applied to  -body systems, this simple formula can lead to rich and diverse dynamics for different values of
-body systems, this simple formula can lead to rich and diverse dynamics for different values of  ,
,  ,
,  , and
, and  .
.
Contributed by: Stefan Ganev (May 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Typically, the attraction term dominates at larger distances, while the repulsion is dominant at smaller distances. For  and
and 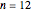 this formula refers to the Lennard–Jones 6-12 potential, used in molecular dynamics models. For
this formula refers to the Lennard–Jones 6-12 potential, used in molecular dynamics models. For 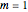 it could be viewed as gravitational potential plus an added repulsive component at close distances. In general, this kind of function could be used in simulations related to a variety of problem domains. In [1] such potential functions are applied to model swarming behavior or variations of chase-and-evade algorithms.
it could be viewed as gravitational potential plus an added repulsive component at close distances. In general, this kind of function could be used in simulations related to a variety of problem domains. In [1] such potential functions are applied to model swarming behavior or variations of chase-and-evade algorithms.
Reference
[1] D. M. Bourg and G. Seemann, AI for Game Developers, Sebastopol, CA: O'Reilly Media, 2004 pp. 80–89.
Permanent Citation