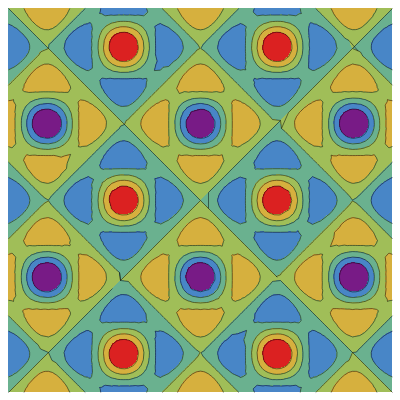
Mathematica MathWorld Wolfram Science More »

Chladni Figures


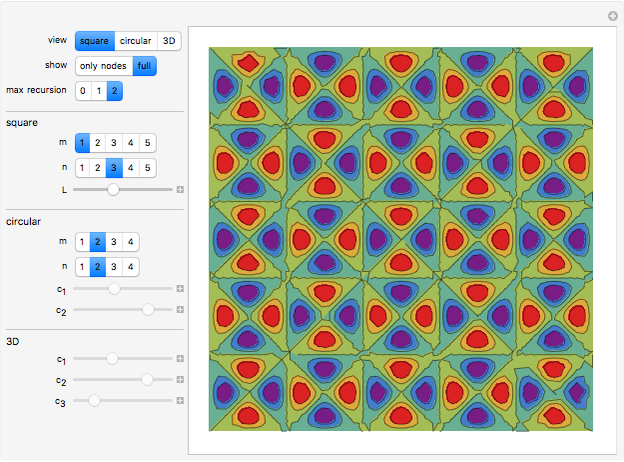
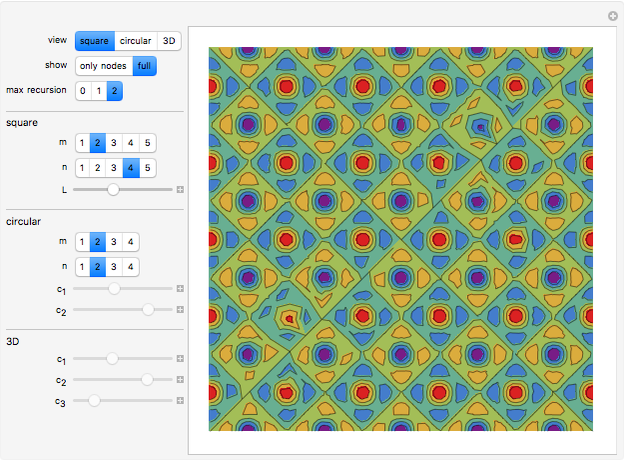
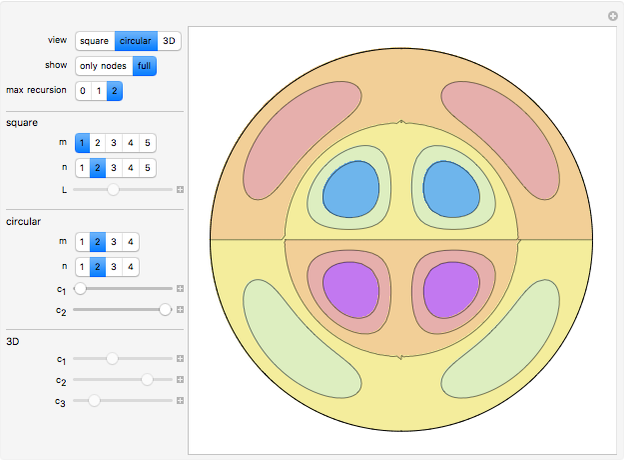
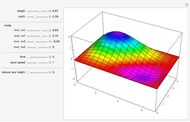
	Chladni figures are wavy lines produced by scattered salt or sand on an elastic material of a given shape, possibly constrained at the edges or at a point in the center, and forced to vibrate with a violin bow. The modes of vibration can be identified because these small particles end up in the places of zero vibration, which happens at those parts of the surface that are stationary, namely at the nodes of vibration, where harmonics cancel. In the case of 3D images, the surfaces result from having three orthogonal harmonics that are periodic outside the domain shown.


	Contributed by: Enrique Zeleny
	Show Initialization Code

For the 2D solution, the following condition holds

for integer values

and

. The solution is uninteresting for

, and for

, notice that the plots are the same.

In the case of 3D solutions,

with

and for the circular membrane

where

is the

zero of the Bessel function of order

is the Bessel function of the first kind and the radius is equal to 1.

Contributed by: Enrique Zeleny

Report an issue »

	Source page:
	Email	Name (optional)
	Occupation (optional)	Organization (optional)
	I use Mathematica often occasionally never
	Country (optional) Remember me
	Note: Please do not include anything you consider confidential or proprietary. Your message and contact information may be shared with the author of any specific Demonstration for which you give feedback, but will not otherwise be published or distributed. Privacy Policy »

RSS

Atom