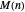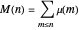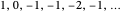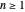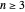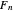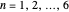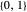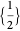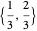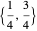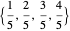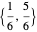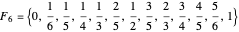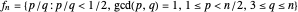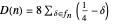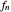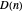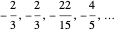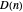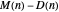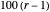Correlating the Mertens Function with the Farey Sequence

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
The Möbius function 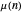 is defined to be
is defined to be 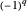 if
if 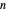 is the product of
is the product of  distinct primes, and zero otherwise, with
distinct primes, and zero otherwise, with 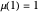 [1]. The values of the Möbius function are
[1]. The values of the Möbius function are 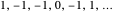 for positive integers
for positive integers 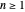 .
.
Contributed by: Jenda Vondra (October 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
For efficiency, we compute 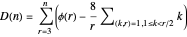 where
where 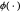 is the Euler totient function [5], and precompute the inside sum [7].
is the Euler totient function [5], and precompute the inside sum [7].
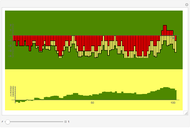
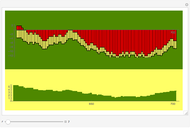
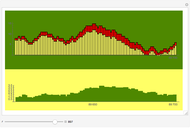
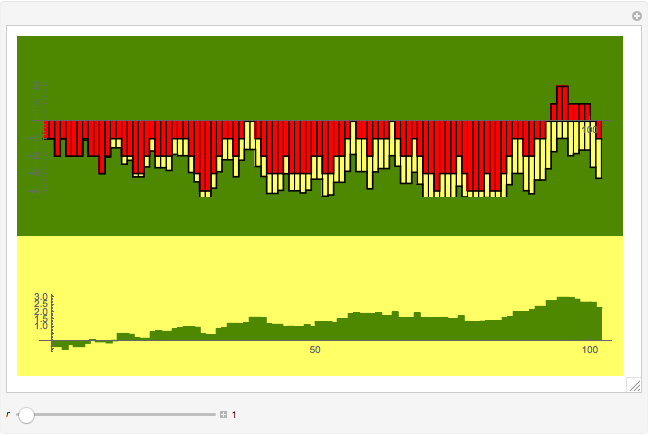
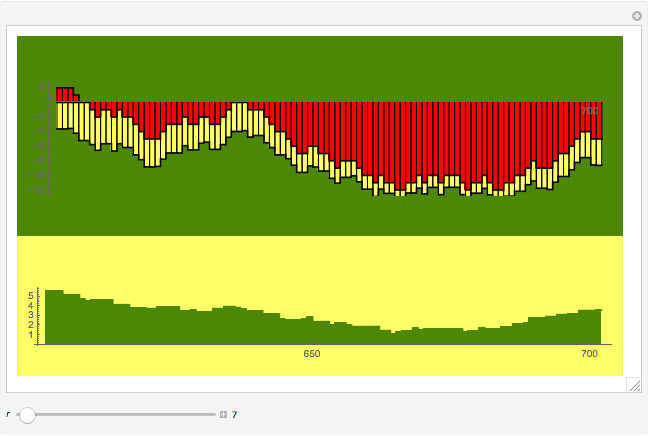
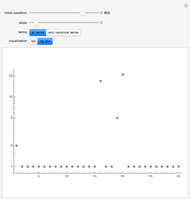
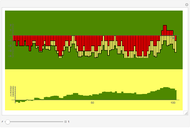
In all, 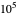 values of the Mertens function
values of the Mertens function 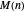 are plotted as red bars in intervals of 100 per visual frame [6].
are plotted as red bars in intervals of 100 per visual frame [6].
Corresponding values of the distribution measure 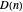 are overlaid in yellow to compare the two function values.
are overlaid in yellow to compare the two function values.
Where one colored bar hides the other fully, use the difference 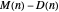 plotted below as green bars to compare values.
plotted below as green bars to compare values.
For 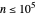 , the heights of the red and yellow bars show correlations and patterns as expected from mappings that exist between the Möbius function
, the heights of the red and yellow bars show correlations and patterns as expected from mappings that exist between the Möbius function 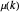 and subsets of the Farey sequence
and subsets of the Farey sequence 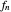 with denominators
with denominators  [4].
[4].
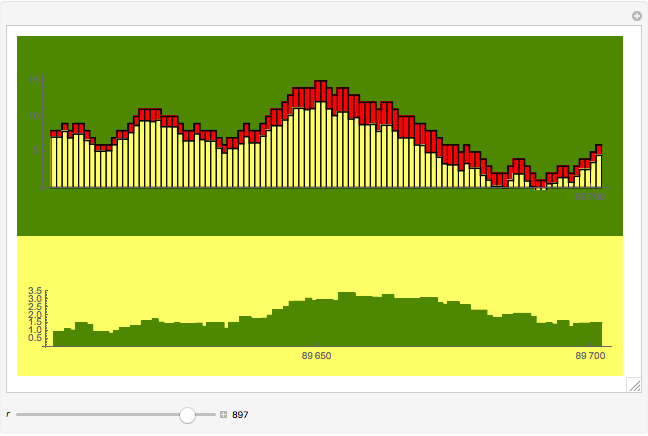
For 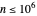 ,
, 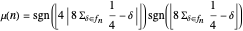 [4], suggesting observable patterns continuing to this range.
[4], suggesting observable patterns continuing to this range.
In [4], it is seen from Ramanujan's sums that 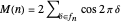 , where
, where  runs over the set
runs over the set 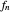 .
.
Let 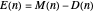 ; then
; then 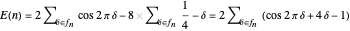 binds the two functions.
binds the two functions.
The distribution of the primes gives the distribution of the Farey sequence via the prime reciprocals.
The distribution of the Möbius function and its summation, the Mertens function, have mysterious random-like properties.
References
[1] Wikipedia. "Möbius Function." (Jun 17, 2013) en.wikipedia.org/wiki/Mobius_function.
[2] Wikipedia. "Mertens Function." (May 3, 2013) en.wikipedia.org/wiki/Mertens_function.
[3] Wikipedia. "Farey Sequence." (Jun 9, 2013) en.wikipedia.org/wiki/Farey_sequence.
[4] J. Vondra. "Experimenting with Sums of Primitive Roots of Unity" from the Wolfram Demonstrations Project. demonstrations.wolfram.com/ExperimentingWithSumsOfPrimitiveRootsOfUnity.
[5] Wikipedia. "Euler's Totient Function." (Sep 18, 2013) en.wikipedia.org/wiki/Euler’s_totient _function.
[6] S. Wolfram. "Mertens Conjecture" from the Wolfram Demonstrations Project. demonstrations.wolfram.com/MertensConjecture.
[7] L. Quet, "Sum of Positive Integers, k, where k <= n/2 and GCD(k,n)=1." (Jan 20, 2002) oeis.org/A066840.
Permanent Citation