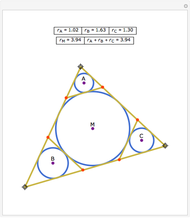
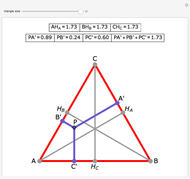
Doug-all Theorem II: Inscribed Triangles

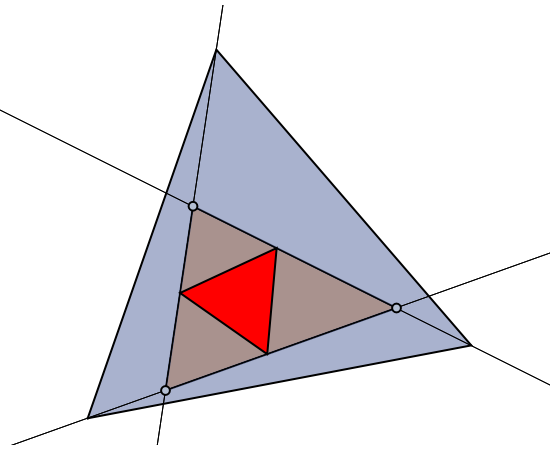
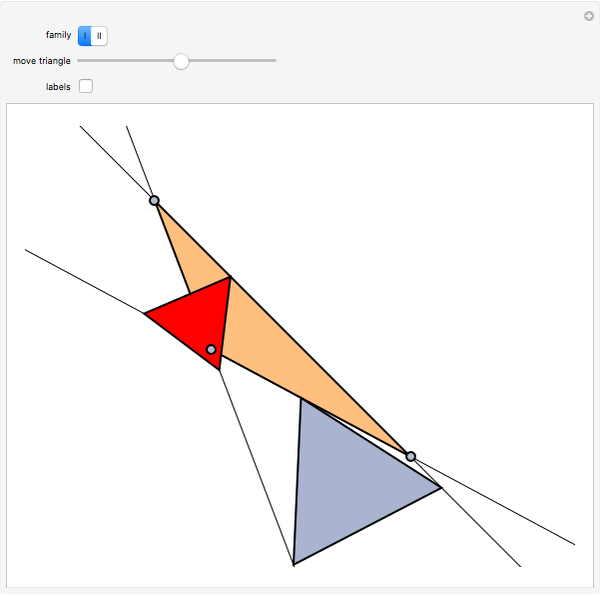
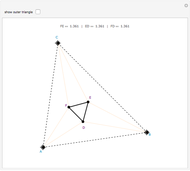
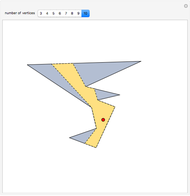
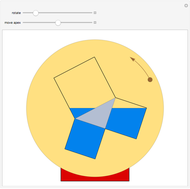
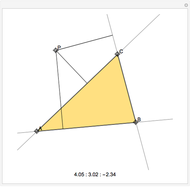
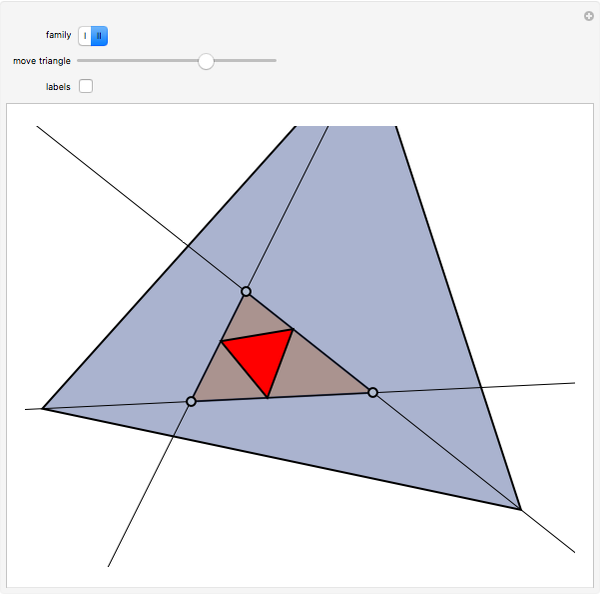
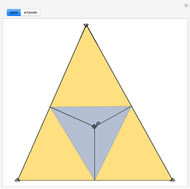
Consider the set of all equilateral triangles inscribed about an arbitrary triangle ; that is, side contains , side contains , and side contains . By "side" we mean the full line, not just the line segment, so that, for instance, point is not necessarily in between and . The Doug-all theorem (its author is not to be confused with the prestigious geometer John Dougall, who died in 1960) claims that there are infinitely many such inscribed triangles, and among them there is a smallest one. 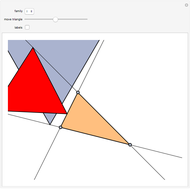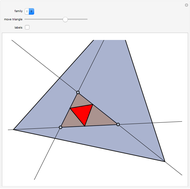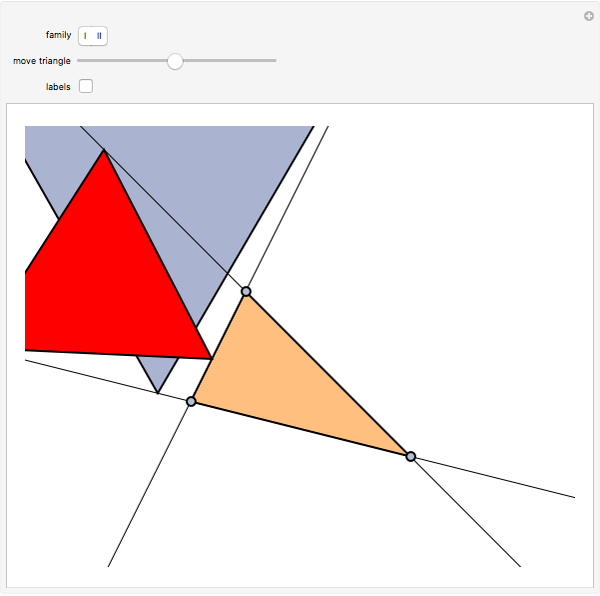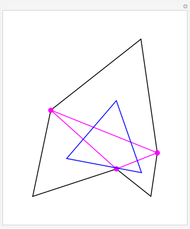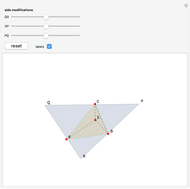There are two families of triangles , one that has triangles completely covering and one never covering . This Demonstration lets you experiment by dragging the vertices of the triangle or moving the inscribed equilateral triangle to verify the existence of the smallest inscribed triangle, which sometimes is outside the triangle!