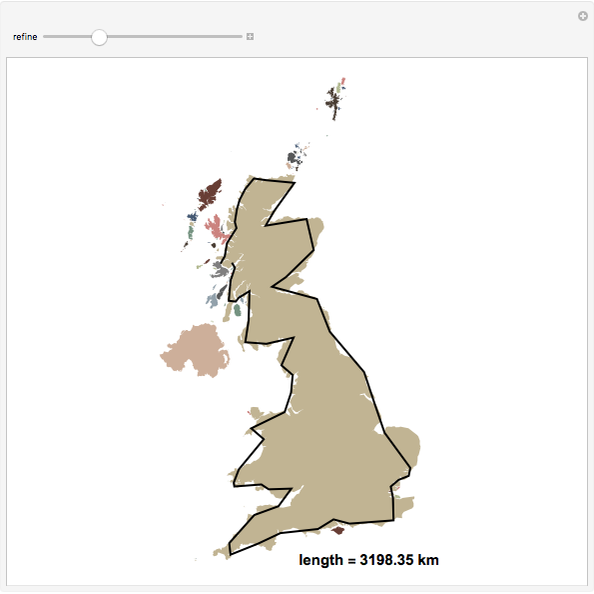
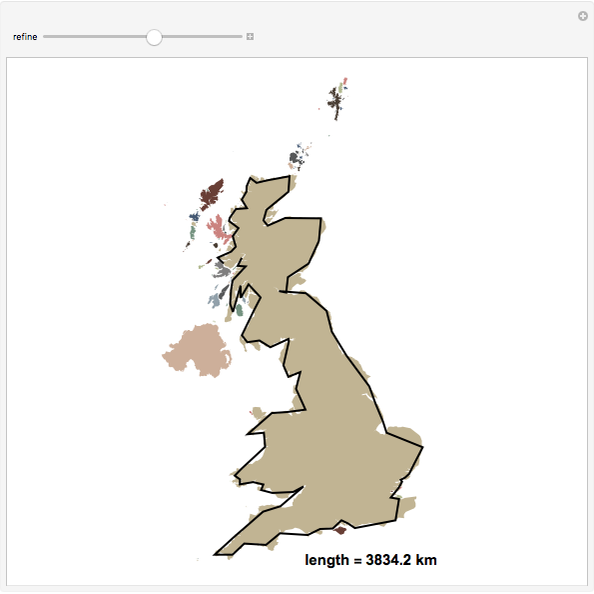
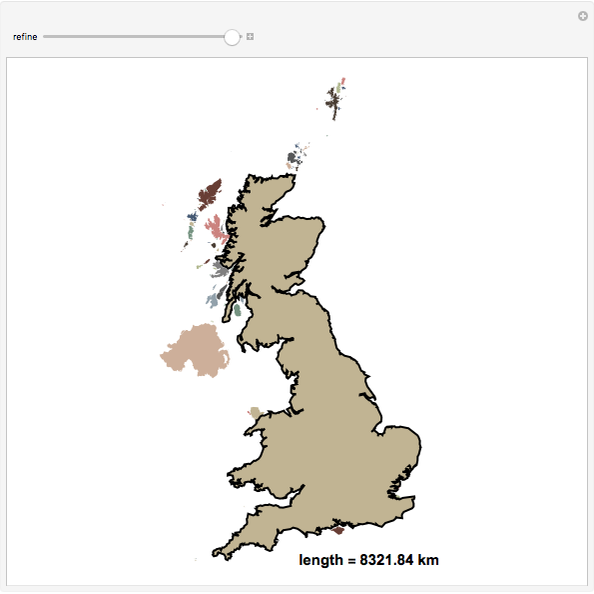
How Long Is the Coast of Britain?

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
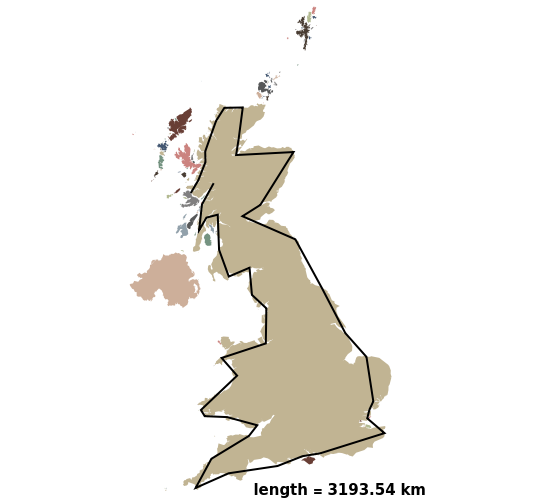
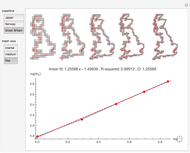
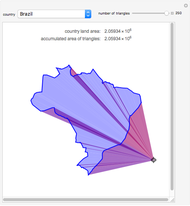
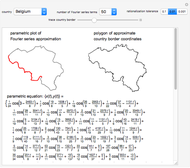
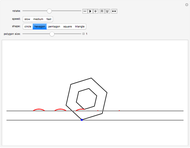
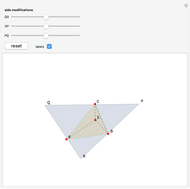
This Demonstration examines the coastline paradox, studied by Benoit Mandelbrot (1924–2010) in his influential 1967 short paper "How Long Is the Coast of Britain?" [3]. The smaller the length of the measuring stick, the longer the length of the coast appears, so that in the limit the coast of the island of Great Britain is of infinite length! Starting from an empirical law obtained previously by Richardson, Mandelbrot introduced the idea of "fractals", concluding that it is meaningless to talk about the length of a coastline and proposing a generalization of the concept of fractal dimension to measure the degree of roughness involved in such measurements.
Contributed by: Jaime Rangel-Mondragon (July 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
References
[1] B. B. Mandelbrot, The Fractal Geometry of Nature, San Francisco: W. H. Freeman and Company, 1982.
[2] Wikipedia. "Benoit Mandelbrot." (Jul 3, 2013) en.wikipedia.org/wiki/Benoit_Mandelbrot.
[3] Wikipedia. "How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension." (Jul 3, 2013) en.wikipedia.org/wiki/How_Long_Is _the _Coast _of _Britain %3 F_Statistical _Self-Similarity_and _Fractional _Dimension.
Permanent Citation
"How Long Is the Coast of Britain?"
http://demonstrations.wolfram.com/HowLongIsTheCoastOfBritain/
Wolfram Demonstrations Project
Published: July 11 2013