First Fermat Point and Isogonic Center of a Triangle
Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
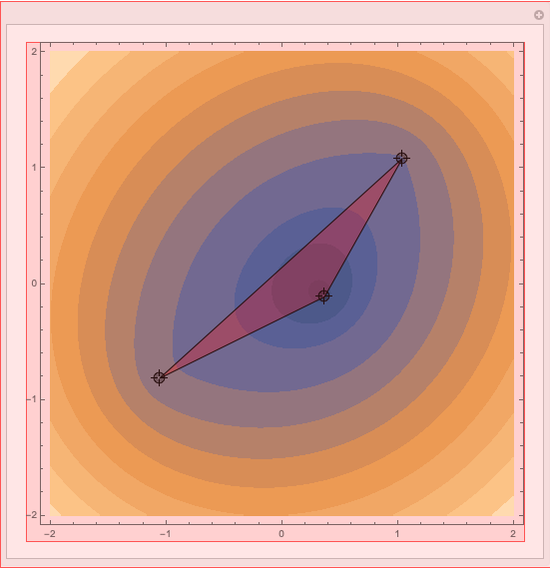
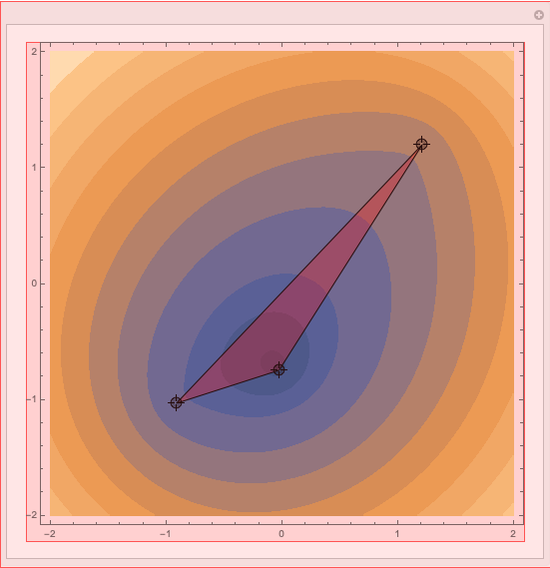
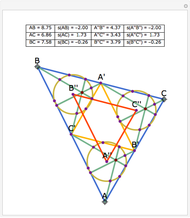
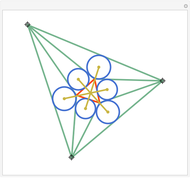
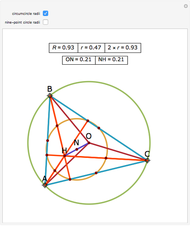
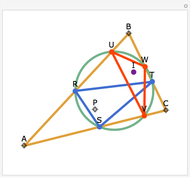
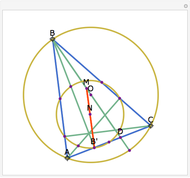
This Demonstration plots the first Fermat point 
(red) and first isogonic center 
(yellow) of a triangle 
. You can drag the triangle vertices. When 
and 
are both inside the triangle, they coincide.
Contributed by: E. Coiras (July 2020)
Open content licensed under CC BY-NC-SA
Snapshots
Details
If all the vertex angles are less than 120º, 
; otherwise 
is the obtuse-angled vertex [2]. The use of a closed formula removes the need for such conditional checks. 
is also the 2D geometric median [3] of the triangle vertices, which as shown here can be calculated by computing two scalar (1D) medians on the corresponding barycentric coordinates [4]. A closed formula for the median of three scalars is also introduced to remove implicit conditional checks.
References
[1] Wikipedia. "Fermat Point." (Jul 13, 2020) en.wikipedia.org/wiki/Fermat_point.
[2] C. Kimberling. "X(13)." Encyclopedia of Triangle Centers. (Jul 13, 2020) faculty.evansville.edu/ck6/encyclopedia/ETC.html.
[3] Wikipedia. "Geometric Median." (Jul 13, 2020) en.wikipedia.org/wiki/Geometric_median.
[4] Wikipedia. "Barycentric Coordinates." (Jul 13, 2020) en.wikipedia.org/wiki/Barycentric_coordinate_system.
Permanent Citation
Sliding to the Fermat Point
Sijia Liang and Bruce Atwood
Circumcircles Intersecting at the First Fermat Point
Jay Warendorff
Fermat Point
Chris Boucher
Spieker Center Triangle Construction
Theodore Gray
A Triangle Formed by the Centers of Three Nine-Point Circles
Jay Warendorff
A First Morley Triangle Concurrence
Jay Warendorff
Point in Triangle
Michael John Twardos
The Center and Radius of the Nine-Point Circle
Jay Warendorff
Pedal Triangles of Isogonal Conjugates
Jay Warendorff
A Collinearity between the Nine-Point Center, the Foot of an Altitude, and a Midpoint
Jay Warendorff