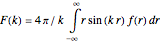Fourier Transform of Radially Symmetric Potential Functions

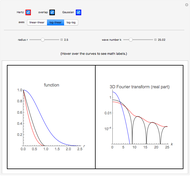
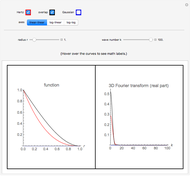
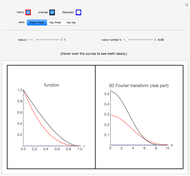
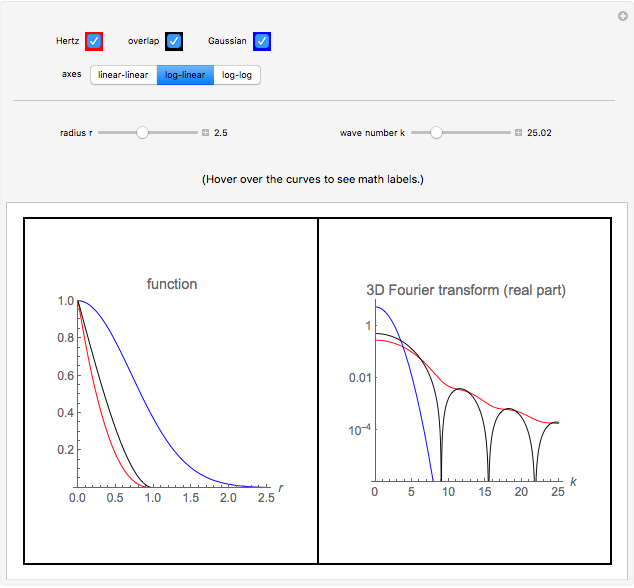
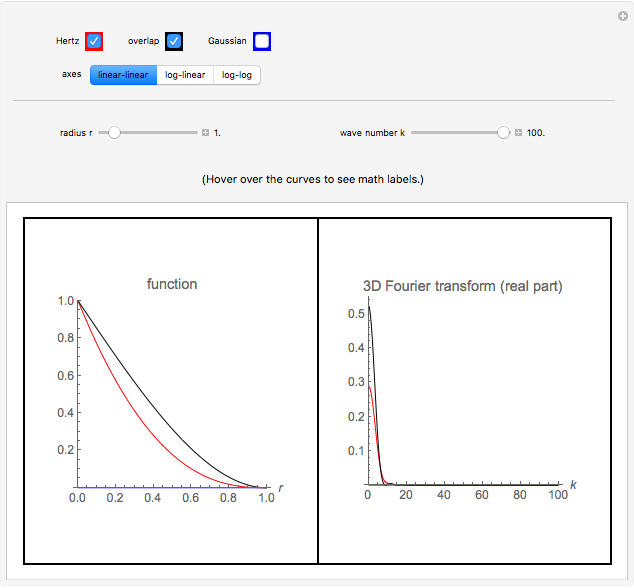
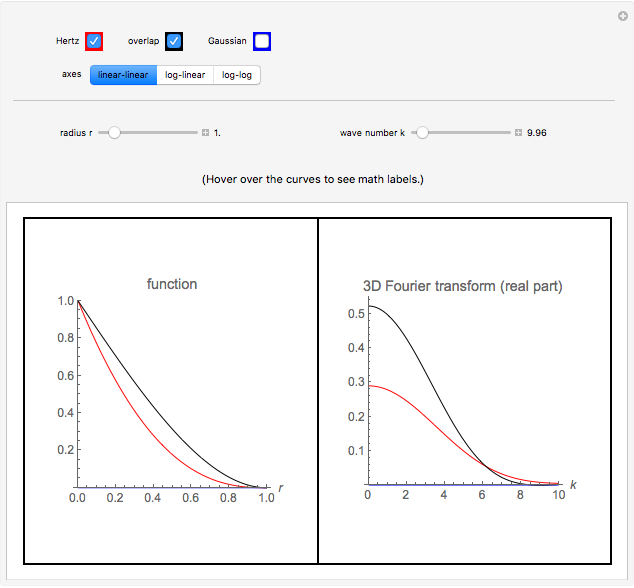
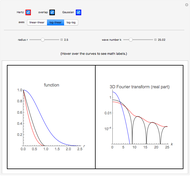
Radially symmetric potential functions that are positive and bounded (i.e., do not diverge at 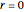) are commonly used to describe the interaction energy between pairs of macromolecules. The centers of mass of two mixed polymers can superimpose when their macromolecules entangle. It turns out that the behavior of these macromolecules (melting, crystallization, and diffusion, for example), is very sensitive to the shape of the potential that describes their interaction. Indeed, the shape of the Fourier transform of this class of potential functions has been shown to be important in classifying the phase behavior of such macromolecules [1].

This Demonstration shows that potential functions that have similar shapes in real space can be very dissimilar in Fourier space. The functions included are the Hertz [2], overlap, and Gaussian potentials. The three-dimensional Fourier transform of a radially symmetrical function is given by

.