Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Geometry of Gregorian Telescope

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

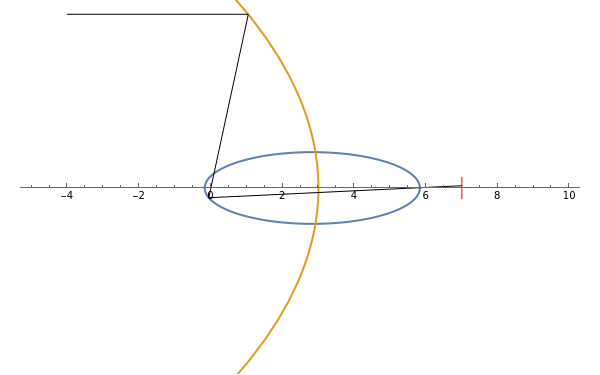
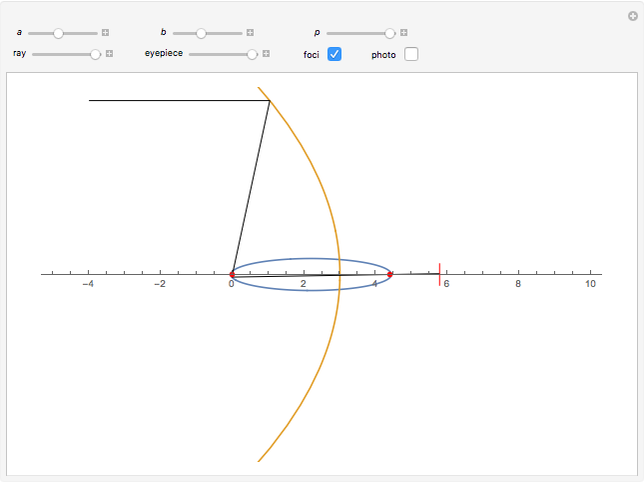
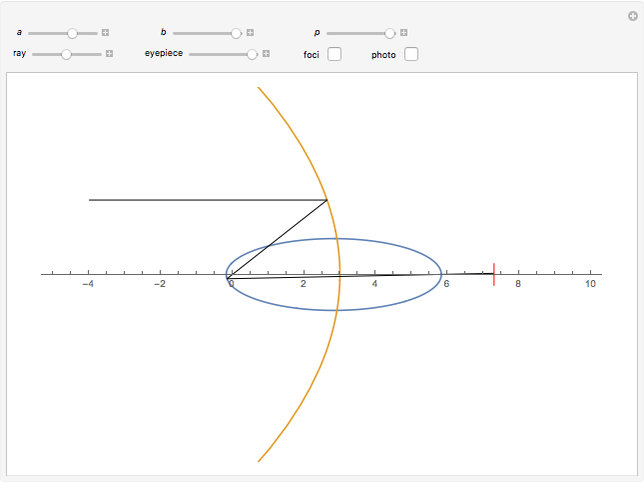
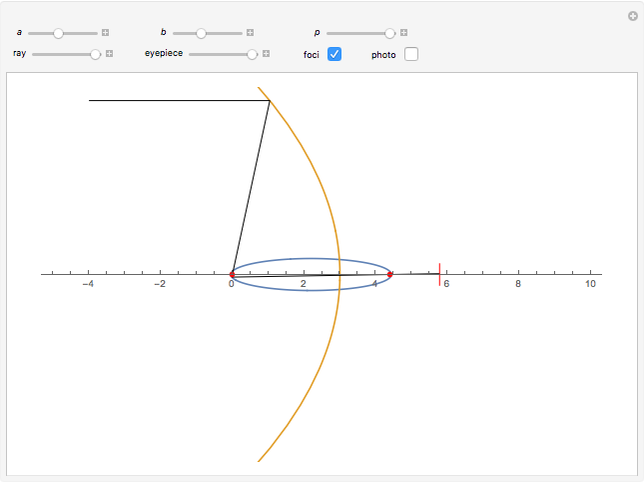
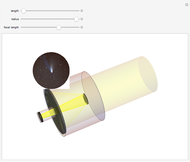
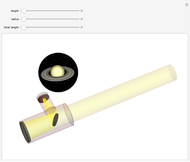
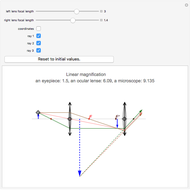
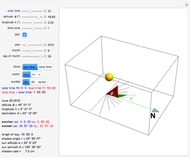
This Demonstration shows the principle of a telescope designed by the Scottish mathematician and astronomer James Gregory in the seventeenth century; it was first built in 1673 by Robert Hooke. The parameters and are the semiaxes of the ellipse, and the equation of the parabola in polar coordinates is The ellipse and parabola have a common focus.

Contributed by: Izidor Hafner (November 2016)
Open content licensed under CC BY-NC-SA

Snapshots

Details

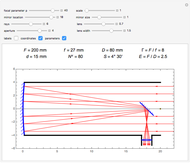
For the construction of a Gregorian telescope see [1].

The photo was taken from [2, p. 94].

References

[1] Wikipedia. "Gregorian Telescope." (Nov 29, 2016) en.wikipedia.org/wiki/Gregorian_telescope.

[2] J. Gregory, Optica Promota, London: Thomson, 1663. books.google.com.mx/books/about/Optica_promota.html?id=W2U_AAAAcAAJ.

[3] King's College London. "James Gregory's Telescope." (Nov 29, 2016) www.kingscollections.org/exhibitions/specialcollections/to-scrutinize-nature/scrutinizing-the-skies/james-gregorys-telescope.

[4] I. Bruce. "James Gregory's Optica Promota." (Nov 29, 2016) www .17centurymaths.com/contents/James%20 Gregory/Prop1OP.pdf.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send