Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Kepler Problem with Classical Spin-Orbit Interaction

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

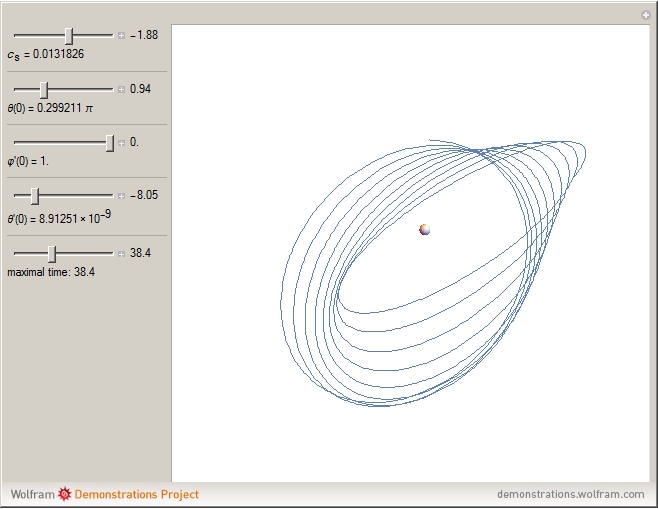
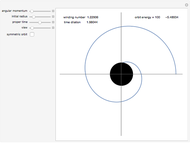
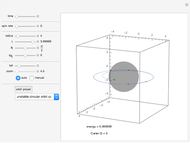
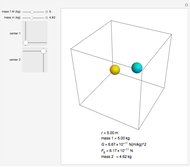
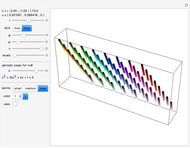
Kepler's laws, derived from Newtonian mechanics, show that planets travel in closed elliptical orbits. The electromagnetic analog (neglecting radiation by accelerated charges) is the basis of Bohr's atomic model. This Demonstration explores the gravitational analog of magnetic phenomena, leading to so-called gravitomagnetic effects in general relativity. Although these are weaker by some 40 orders of magnitude, gravitomagnetism might become significant in an immensely strong gravitational field—for example, in the neighborhood of a black hole or neutron star.

[more]

Specifically, for a spinning mass in a strong gravitational field, general relativity implies the existence of gravitomagnetic dipoles. This creates the possibility for classical spin–orbit interaction as a perturbation to Kepler orbits. This problem is treated within the Newtonian approximation to general relativity.

In this Demonstration, you can vary the strength of the gravitomagnetic dipole , the initial angle , the angular velocities and and the maximum integration time.

[less]

Contributed by: Jarek Duda (January 2017)
Open content licensed under CC BY-NC-SA

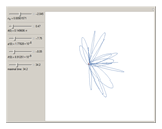
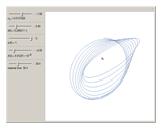
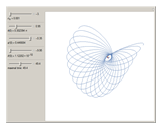
Snapshots

Details

,

where , .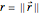

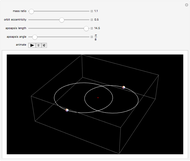
This magnetic field can be the result of intrinsic magnetic dipole moment of electron, or of a magnet, or the spinning of a charged object. For a spinning massive body, one can propose analogous gravitomagnetic [2] corrections to Newtonian gravity, such as the frame-dragging effect [3].

The simplest Kepler problem for orbits around a massive object, including the classical analog of spin–orbit interaction [4], leads to:

,

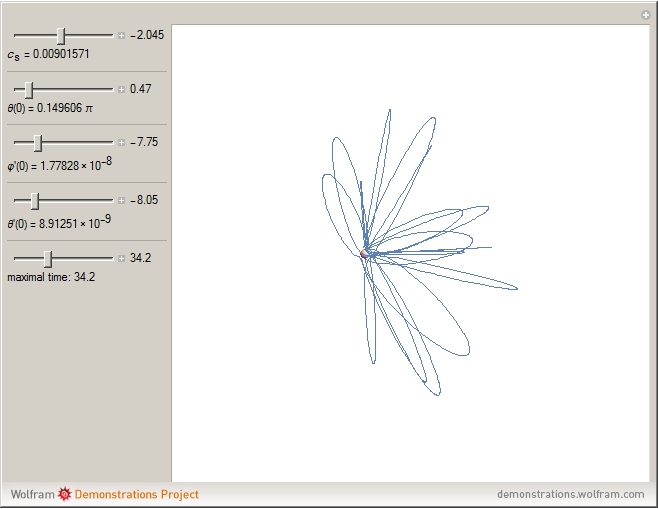
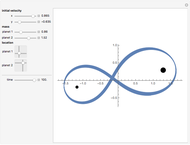
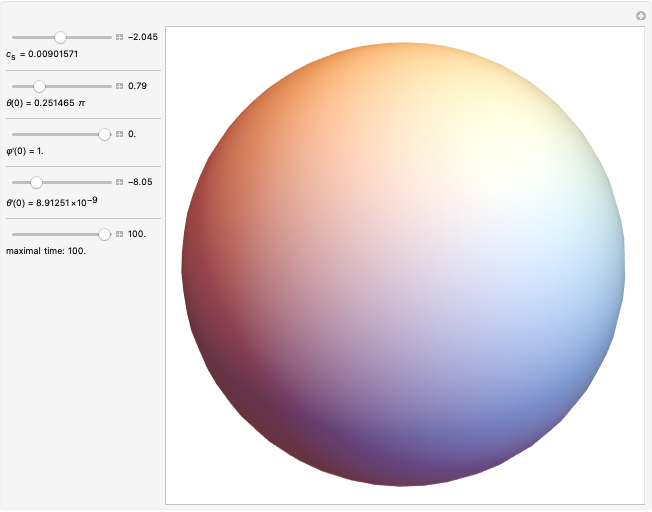
The next slider lets you choose the initial angle between the incoming object and the spin direction, which is fixed here as .

The next two sliders set the initial angular velocity for azimuthal and polar angles . The final slider sets the integration time.

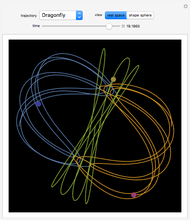
It is an interesting, open question to understand and characterize the closed trajectories of the magnetic dipole in classical electromagnetism [5].

References

[1] Wikipedia. "Magnetic Dipole." (Jan 5, 2017) en.wikipedia.org/wiki/Magnetic_dipole.

[2] Wikipedia. "Gravitoelectromagnetism." (Jan 5, 2017) en.wikipedia.org/wiki/Gravitoelectromagnetism.

[3] Wikipedia. "Frame-Dragging." (Jan 5, 2017) en.wikipedia.org/wiki/Frame-dragging.

[4] Wikipedia. "Spin–Orbit Interaction." (Jan 5, 2017) en.wikipedia.org/wiki/Spin-orbit interaction.

[5] Wikipedia. "Michał Gryziński." (Jan 5, 2017) (redirected from) en.wikipedia.org/wiki/Free-fall_atomic_model.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send