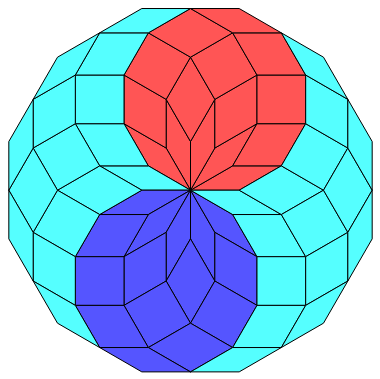
Lindgren's Symmetrical Decompositions of Regular 2n-Gons

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
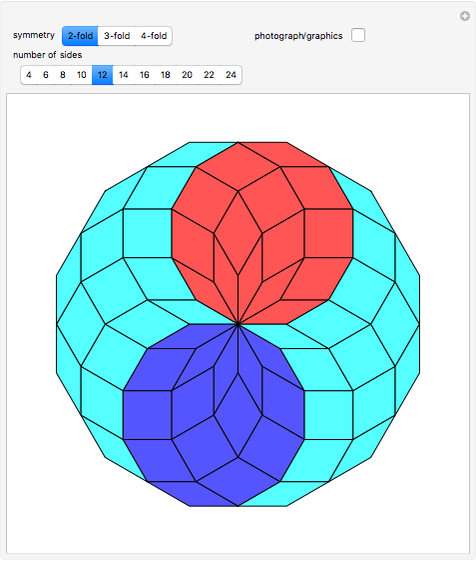
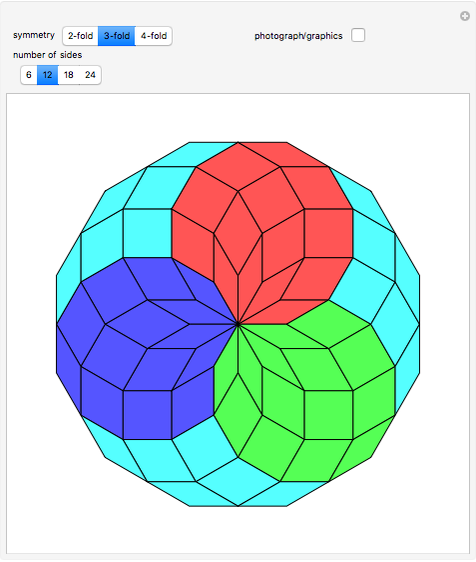
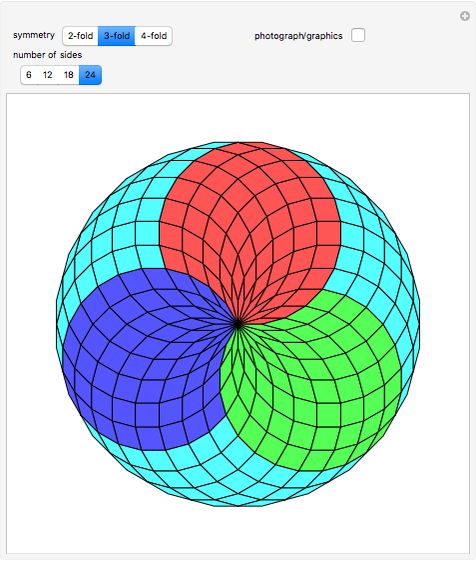
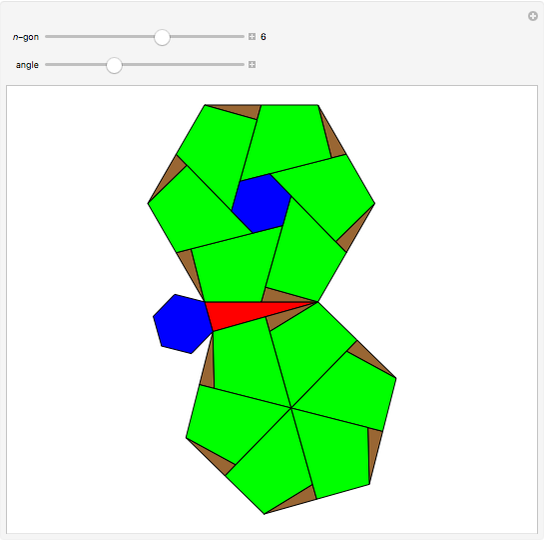
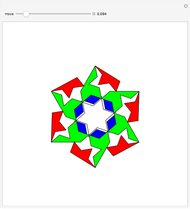
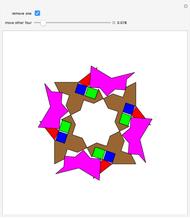
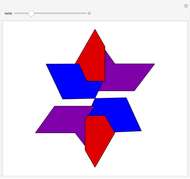
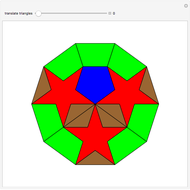
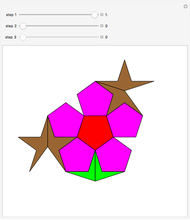
This Demonstration shows Lindgren's symmetrical decompositions of  -gons. These decompositions are also dissections of
-gons. These decompositions are also dissections of  -gons into four smaller
-gons into four smaller  -gons, which you can verify by counting rhombuses.
-gons, which you can verify by counting rhombuses.
Contributed by: Izidor Hafner (July 2016)
Open content licensed under CC BY-NC-SA
Snapshots
Details
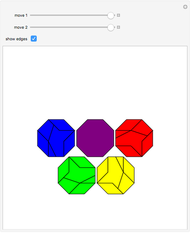
Figure 21.6 in [1] shows three symmetric assemblies of four dodecagons into one, each assembly requiring 12 pieces. Lindgren writes: "These are referred to, but not described, by H. M. Cundy and C. D. Langford, Mathematical Gazette, vol. 44, no. 46 (1960), Note 2875. There have probably been several independent discoveries of them." [2].
For example, Ernest Irving Freese shows the third of the three dissections in Plate 121 of his unpublished 1957 manuscript. These facts together are the most complete attribution for this problem (information from G. N. Frederickson).
The photograph is taken from [3, p. 56], which is taken from [4].
References
[1] H. Lindgren, Geometric Dissections, Princeton, NJ: Van Nostrand, 1964.
[2] H. M. Cundy and C. G. Langford, "On the Dissection of a Regular Polygon into  Equal and Similar Polygons," Mathematical Gazette, 44(46), 1960, Note 2875.
Equal and Similar Polygons," Mathematical Gazette, 44(46), 1960, Note 2875.
[3] D. Wells, The Penguin Dictionary of Curious and Interesting Geometry, London: Penguin Books, 1991.
[4] H. Lindgren, Recreational Problems in Geometric Dissections and How to Solve Them, New York: Dover, !972.
[5] G. N. Frederickson, Dissections: Plane & Fancy, New York: Cambridge University Press, 2003 pp. 10–11.
[6] W. W. Rouse Ball and H. S. M. Coxeter, Mathematical Recreations and Essays, 13th ed., New York: Dover, 1987 p. 142.
[7] Wikipedia. "Dodecagon." (Jun 30, 2016) en.wikipedia.org/wiki/Dodecagon.
Permanent Citation