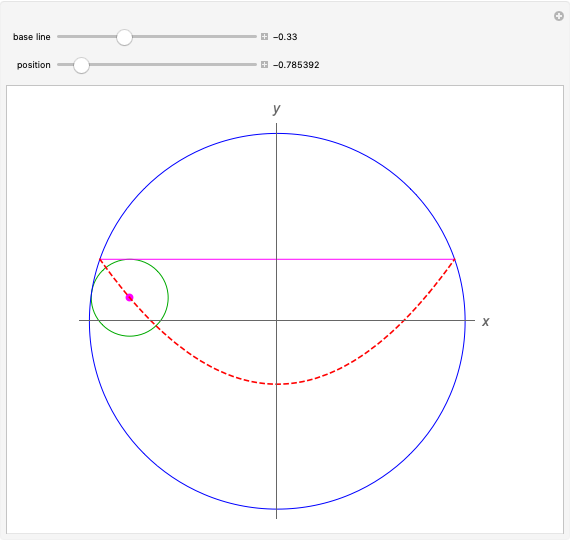
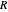Locus of the Center of a Circle Inscribed in a Circular Segment
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
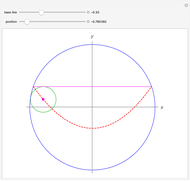
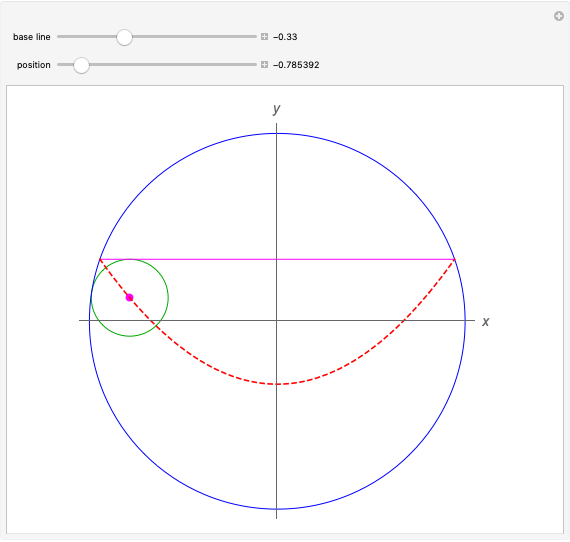
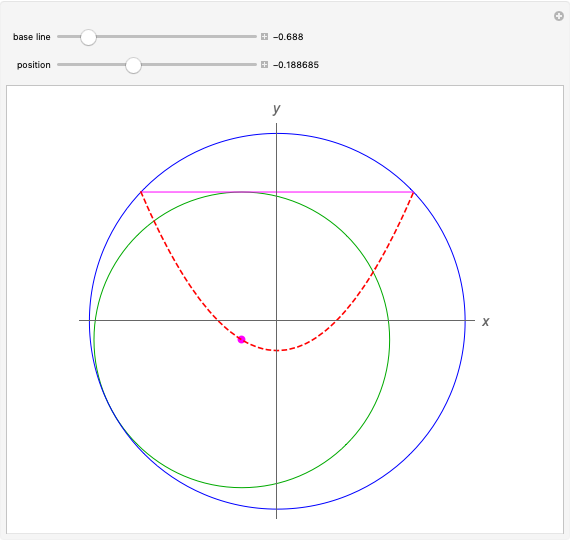
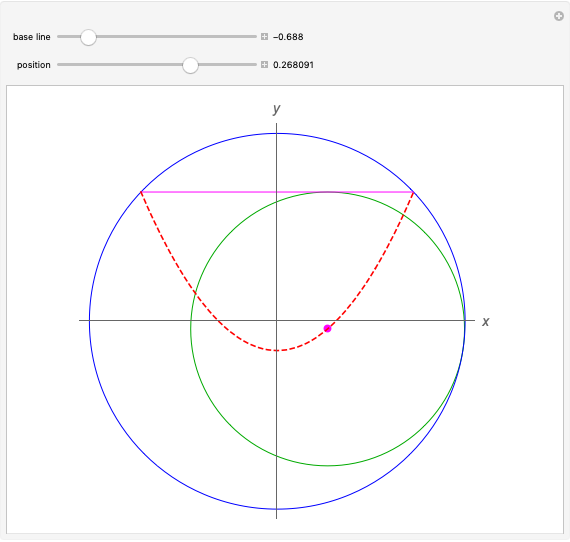
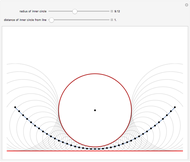
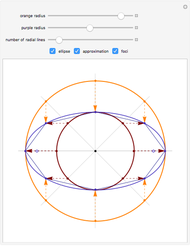
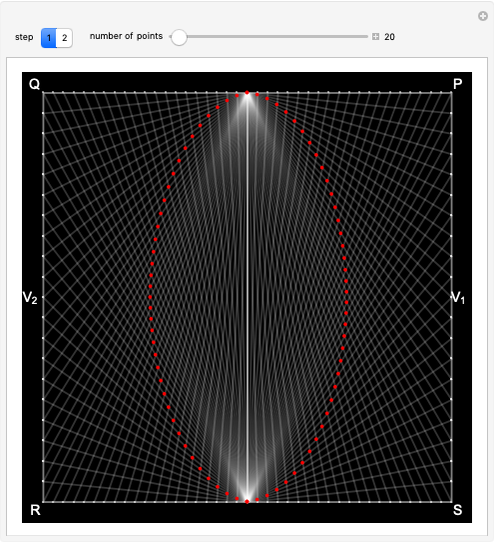
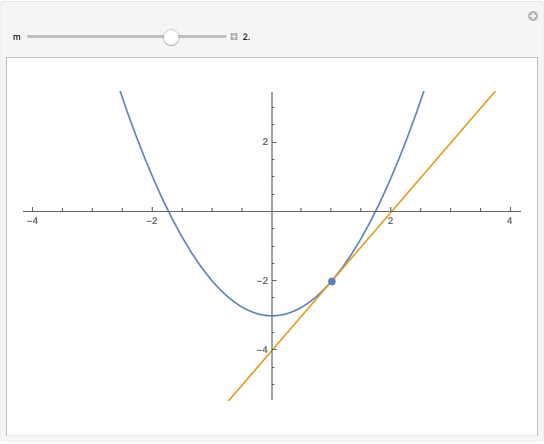
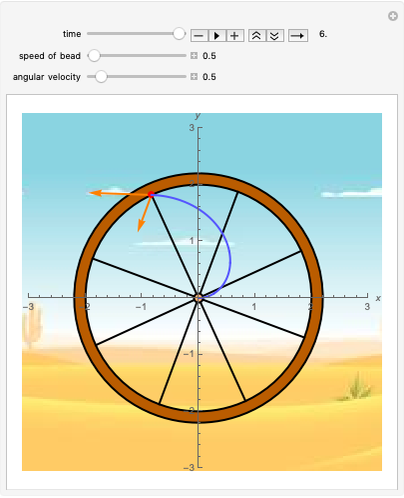
The trajectory of the center of a moving circle inscribed in a circular segment is parabolic.
[more]
Contributed by: Diego Ramos (May 2019)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation