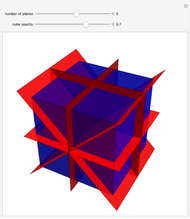
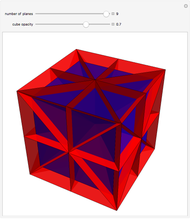
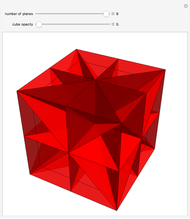
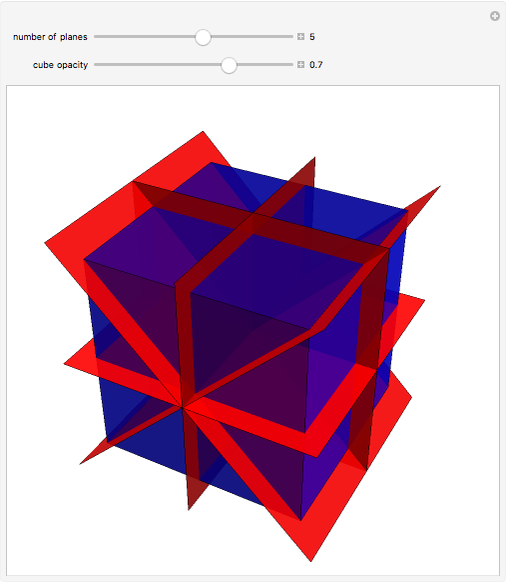
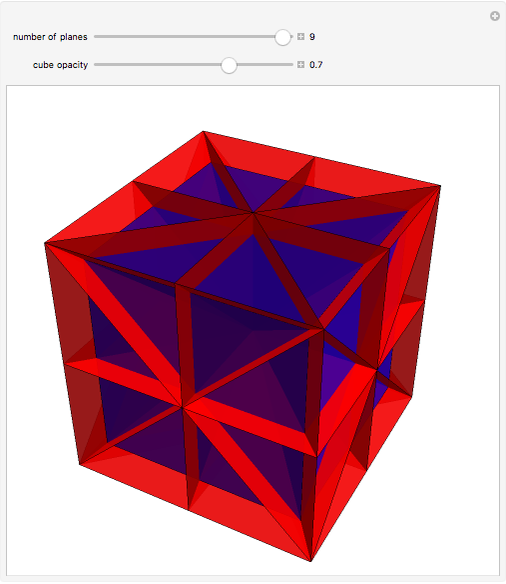
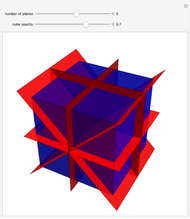
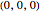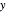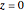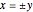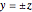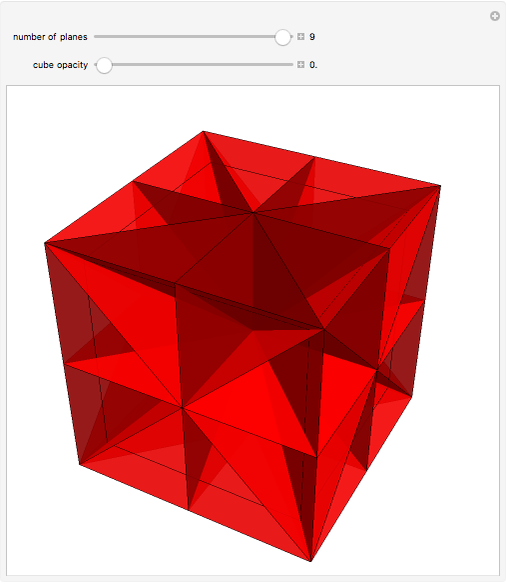
Use the slider to show the nine planes of symmetry (or mirror planes) for the cube. If the center of the cube is the origin and the , , and axes are normal to opposite pairs of faces, the planes have equations , , , , , and 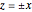. With all nine cuts, each of the six faces of the cube is cut into eight triangles. For each such triangle, join its three vertices to the center of the cube to form a tetrahedron. These 48 tetrahedra partition the cube. (Reduce the opacity to see their interiors.)