Oscillation of a Mass-Spring System as a Model for Lattice Vibration

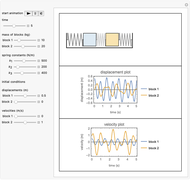
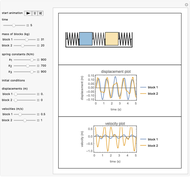
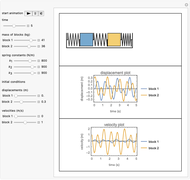
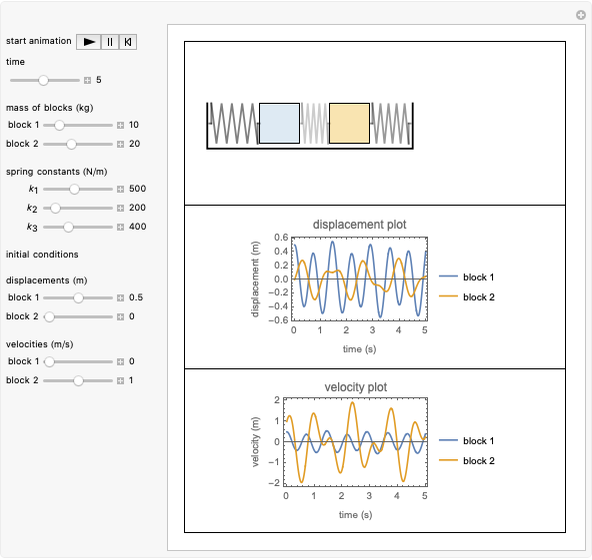
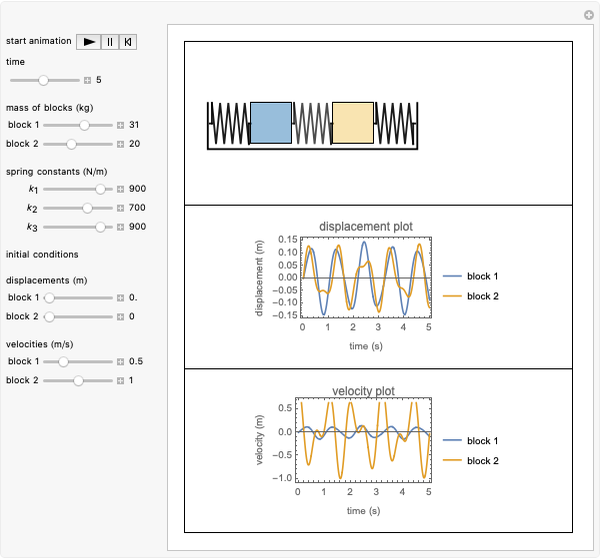
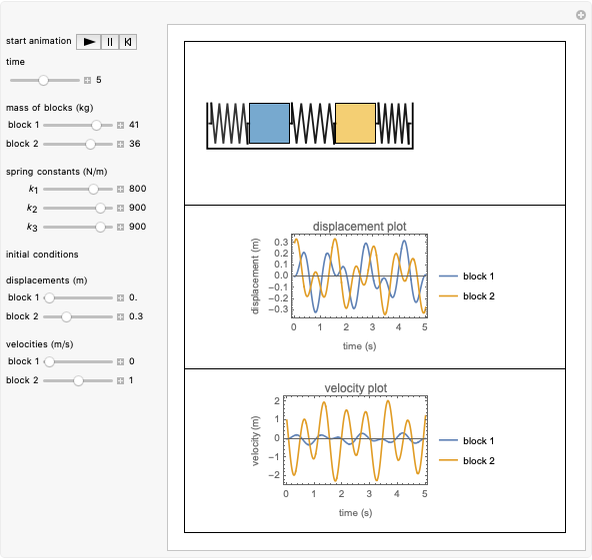
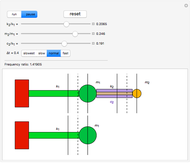
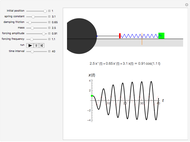
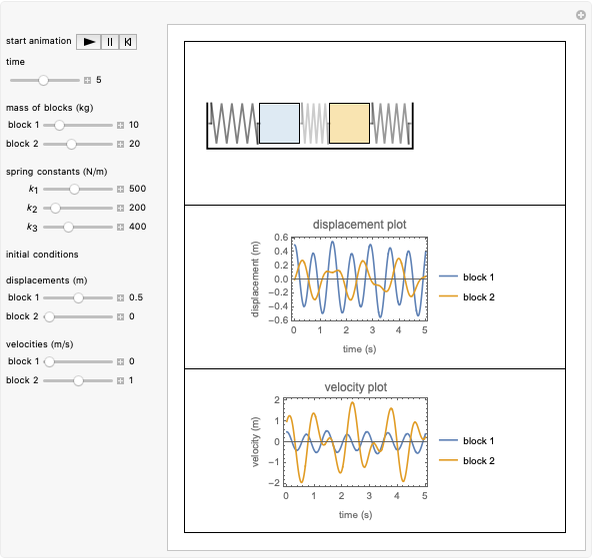
This Demonstration considers a system of two blocks and three springs as a model for lattice vibration of atoms in a one-dimensional crystal. You can vary the block masses, spring constants, initial displacements and initial velocities. The darker the color of the block, the heavier it is. The darker the spring, the stiffer it is. The displacements and velocities of the two blocks are plotted as functions of time.