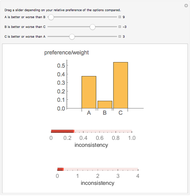
Preference Weights from Pairwise Comparisons

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
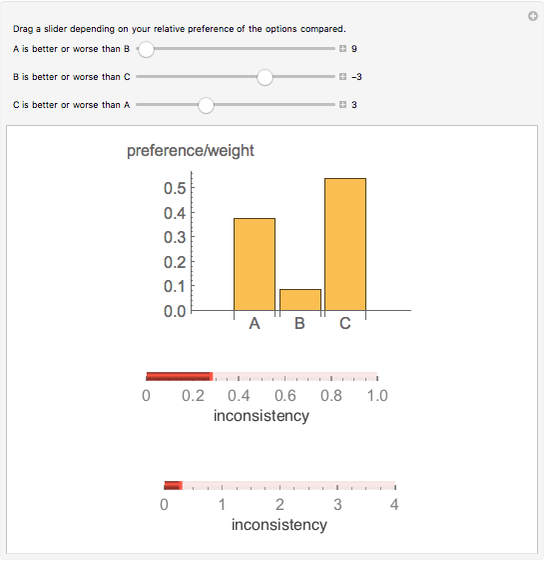
You can compare the three options A, B, and C (which could be alternative projects, products to buy, binary decisions, or power groups).
[more]
Contributed by: Johannes M. Lehner (December 2015)
Johannes Kepler University Linz, Austria
Open content licensed under CC BY-NC-SA
Snapshots
Details
The procedure follows the analytic hierarchy process (AHP) proposed by Saaty [1] and can be applied at different levels of a decision process to weigh the relative power of stakeholder groups (options would be replaced by the names of these groups), decision criteria (such as quality, price, and speed), and alternatives with regard to all of the criteria compared at higher levels of the process. The final decision is derived from weighted preferences with weights from the various levels of the process.
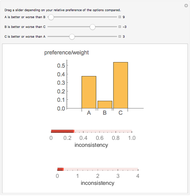
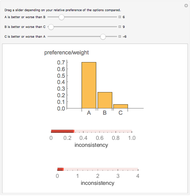
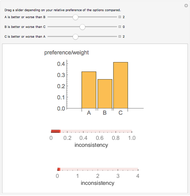
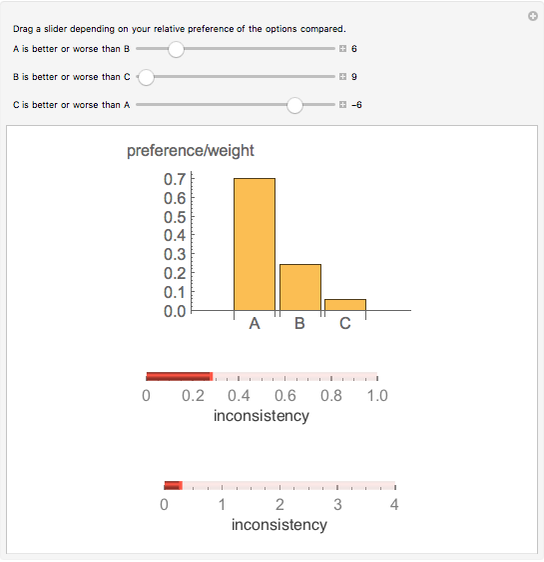
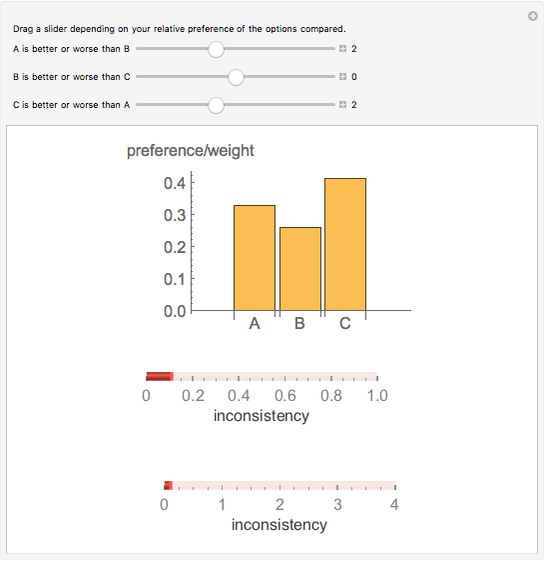
In addition to the preference weights, the procedure also delivers an index of consistency (or inconsistency), shown below the bar chart of preference weights. If pairwise comparisons mutually contradict each other (examples are shown in the snapshots), the inconsistency index is high; otherwise, it is close to zero. The first snapshot shows an example for highly consistent comparisons. The second snapshot shows an example for highly inconsistent comparisons. On the other hand, it is easier to be consistent if preferences are unclear (third snapshot).
This Demonstration is restricted to three options, but the procedure can be easily expanded to any number of alternatives. However, with the number  of alternatives the number of necessary pairwise comparisons is
of alternatives the number of necessary pairwise comparisons is  (e.g. six pairwise comparisons with four options). Usually inconsistency also increases with the number of alternatives to be compared.
(e.g. six pairwise comparisons with four options). Usually inconsistency also increases with the number of alternatives to be compared.
Reference
[1] T. L. Saaty, The Analytic Hierarchy Process, New York: McGraw-Hill, 1980.
Permanent Citation
"Preference Weights from Pairwise Comparisons"
http://demonstrations.wolfram.com/PreferenceWeightsFromPairwiseComparisons/
Wolfram Demonstrations Project
Published: December 30 2015