Quantum Probability as Area on Bloch Sphere

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
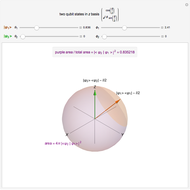
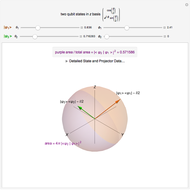
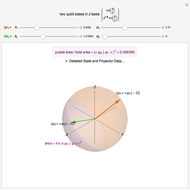
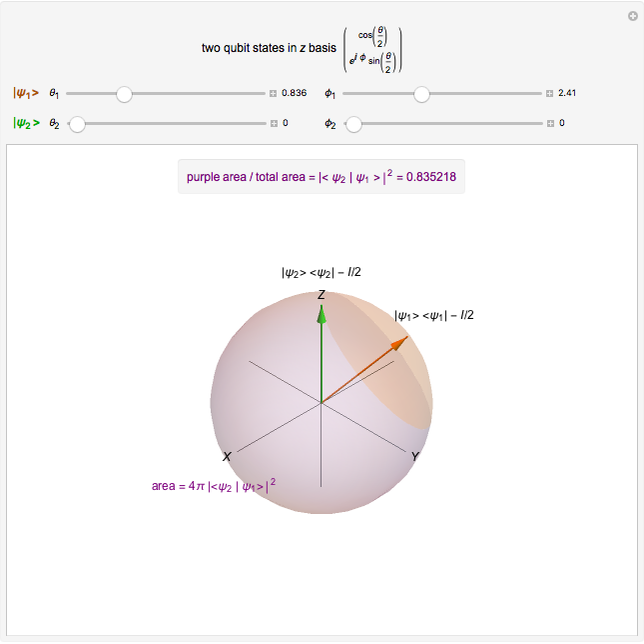
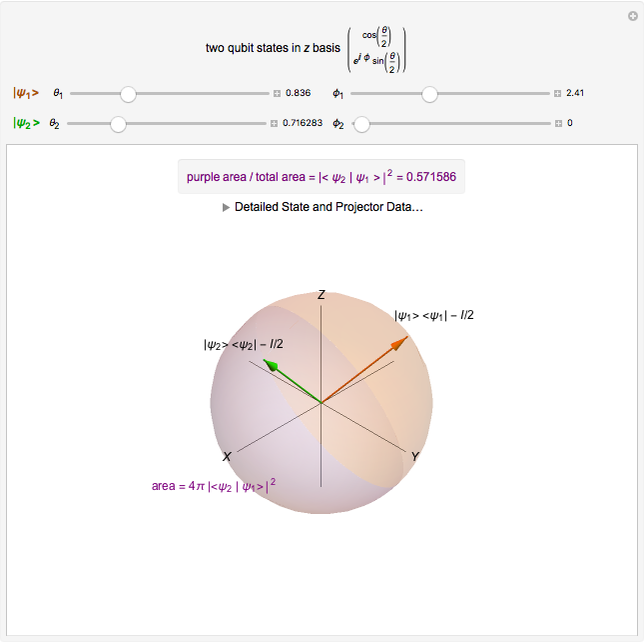
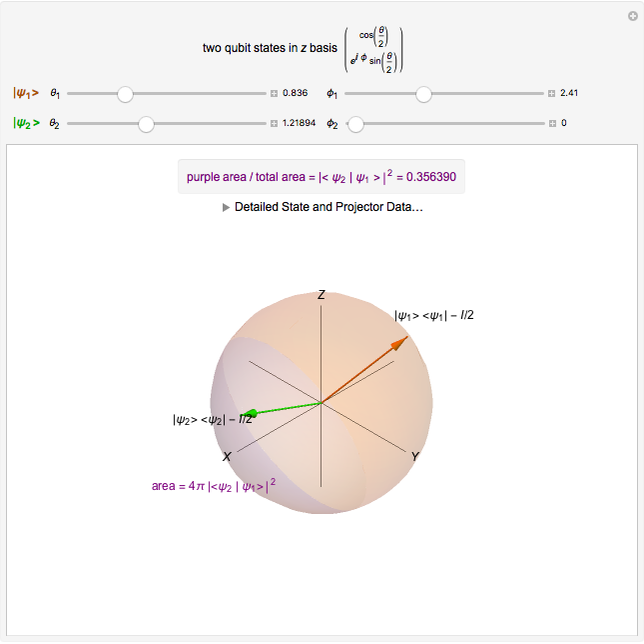
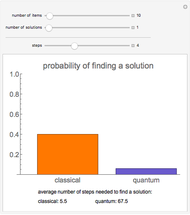
Qubits (quantum bits) are two-state quantum systems. The quantum state of a qubit can be visualized as a point on the Bloch sphere. For a qubit prepared in a state  , the probability of measuring the system to be in a second state,
, the probability of measuring the system to be in a second state,  , can also be visualized on the Bloch sphere. The probability is proportional to the area of the complementary spherical cap obtained by rotating one Bloch vector around the other.
, can also be visualized on the Bloch sphere. The probability is proportional to the area of the complementary spherical cap obtained by rotating one Bloch vector around the other.
Contributed by: John Dell (January 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
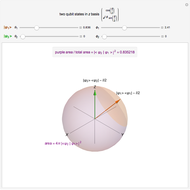
Qubit quantum states can be represented as unit vectors in space. The unit sphere of all such unit vectors is often referred to as the Bloch sphere. This representation is obtained from the 2D complex vector representation by constructing the projection operator corresponding to each state. Expanding the projection operator as a real linear combination of the 2×2 identity operator and the three Pauli spin operators yields a list of four real number coefficients,  , where
, where  . This is the Bloch sphere representation. In this representation, states that are orthogonal in the quantum inner product (i.e. where
. This is the Bloch sphere representation. In this representation, states that are orthogonal in the quantum inner product (i.e. where  ) are represented as opposite vectors on the Bloch sphere. So quantum orthogonality is not the same as geometric orthogonality of Bloch sphere vectors. This Demonstration illustrates that the probability
) are represented as opposite vectors on the Bloch sphere. So quantum orthogonality is not the same as geometric orthogonality of Bloch sphere vectors. This Demonstration illustrates that the probability  , where
, where  is the angle between the vectors on the Bloch sphere, can be visualized in terms of an area on the Bloch sphere determined by the two states. This is a consequence of the symmetry of unitary spaces used in quantum mechanics. A more complicated formula relates the quantum probability to a complementary volume for finite-dimensional state spaces with the canonical Hermitian inner product.
is the angle between the vectors on the Bloch sphere, can be visualized in terms of an area on the Bloch sphere determined by the two states. This is a consequence of the symmetry of unitary spaces used in quantum mechanics. A more complicated formula relates the quantum probability to a complementary volume for finite-dimensional state spaces with the canonical Hermitian inner product.
Permanent Citation