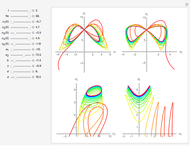
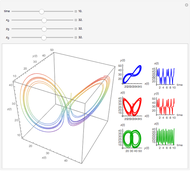
Maxwell-Bloch Equations for a Laser

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
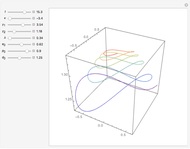
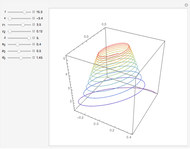
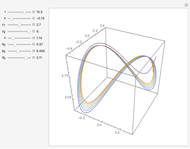
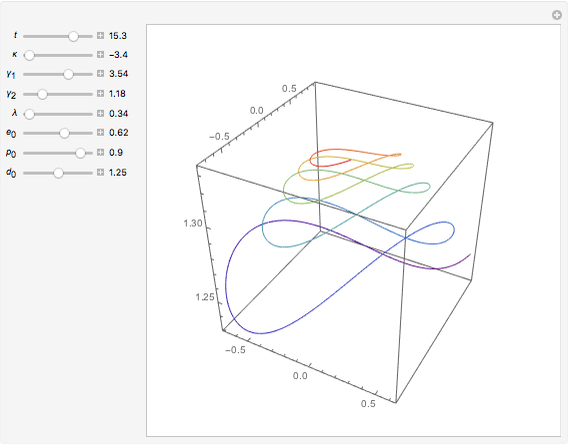
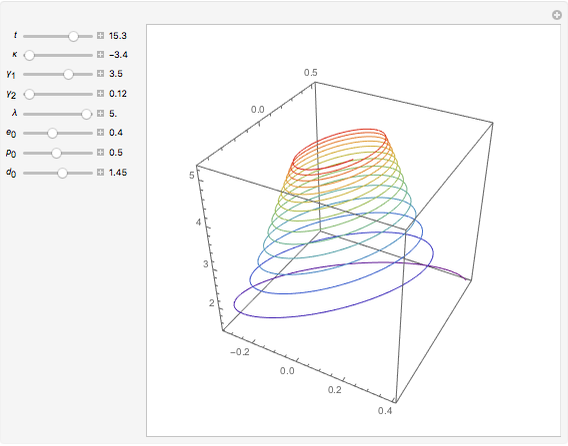
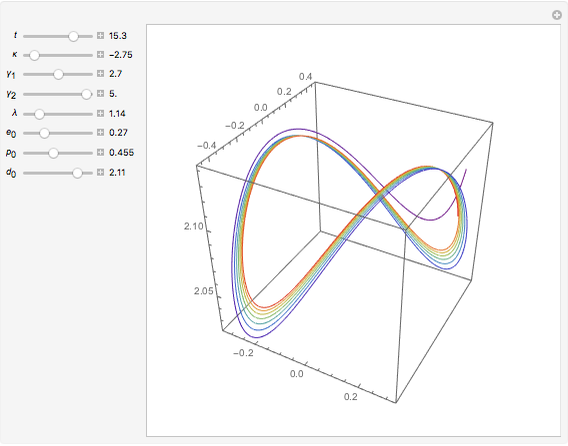
In nonlinear optics, the Maxwell–Bloch equations can be used to describe laser systems. They consist of three first-order equations for the electric field in a single longitudinal cavity mode, which became nonlinear because the system oscillates between at least two discrete energy levels. The system is derived from Maxwell's equations, using semiclassical approximations for quantum variables. The equations are solved for population inversion density  and mean atomic-polarization density
and mean atomic-polarization density  , induced by an electric field
, induced by an electric field  . Here
. Here  is the decay rate in the laser cavity due to beam transmission. The parameters
is the decay rate in the laser cavity due to beam transmission. The parameters  and
and  are decay rates of atomic polarization and population inversion and
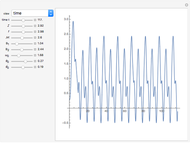
are decay rates of atomic polarization and population inversion and  is a pumping energy parameter. Chaotic behavior and period doubling has been observed experimentally. These equations are related to the Lorenz equations and can exhibit strange attractors.
is a pumping energy parameter. Chaotic behavior and period doubling has been observed experimentally. These equations are related to the Lorenz equations and can exhibit strange attractors.
Contributed by: Enrique Zeleny (December 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The Maxwell–Bloch equations can be written as:
 ,
,
 ,
,
 .
.
References
[1] H. Haken, Light: Laser Light Dynamics, Vol. 2, New York: North-Holland Publishing Company, 1985.
[2] A. Scott (ed.), Encyclopedia of Nonlinear Science, New York: Routledge, 2005 pp. 564–566.
Permanent Citation