Stationary Precession of a Spinning Top

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
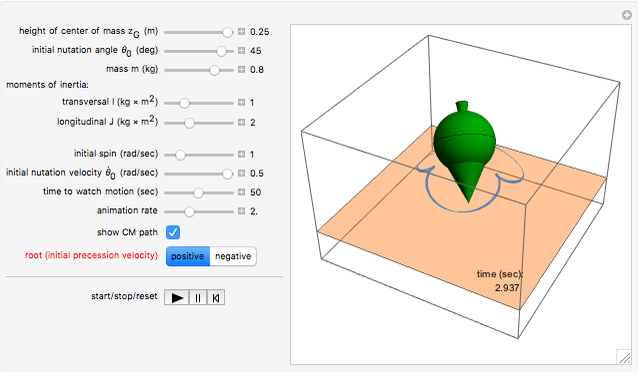
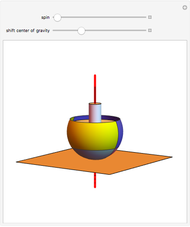
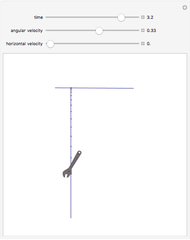
The stationary precession motion of a frictionless symmetric top under the influence of gravity can be described by a nonlinear ODE:
[more]
Contributed by: William C. Guttner (March 2009)
Open content licensed under CC BY-NC-SA
Snapshots
Details
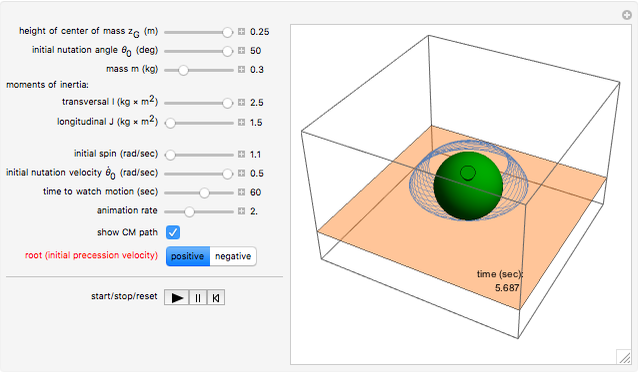
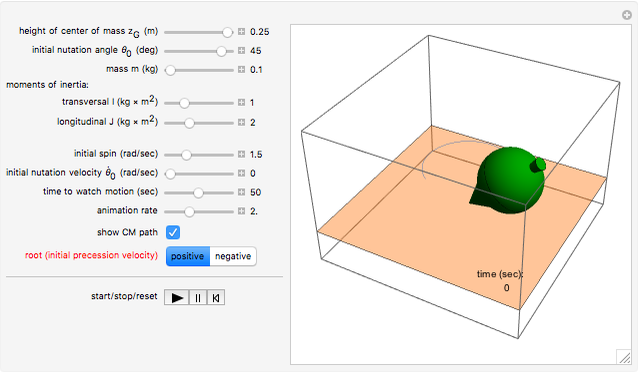
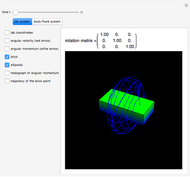
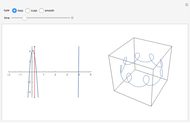
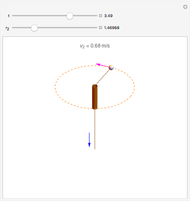
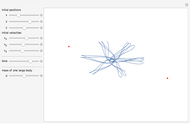
Snapshots 1 and 2: adding an initial nutation velocity, the motion becomes a combination of a stationary precession and a nutation motion
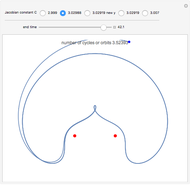
Snapshot 3: increasing the initial spin, the "gyroscopic stability" increases, which reduces the precession velocity: 
Reference: C. P. Pesce, Dinâmica dos Corpos Rígidos, Escola Politécnica da Universidade de São Paulo, 2004.
Permanent Citation