Flip Bifurcation in Dynamical Systems

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
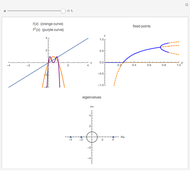
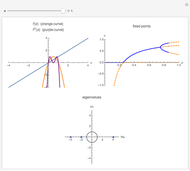
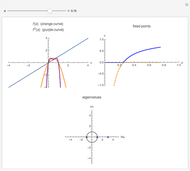
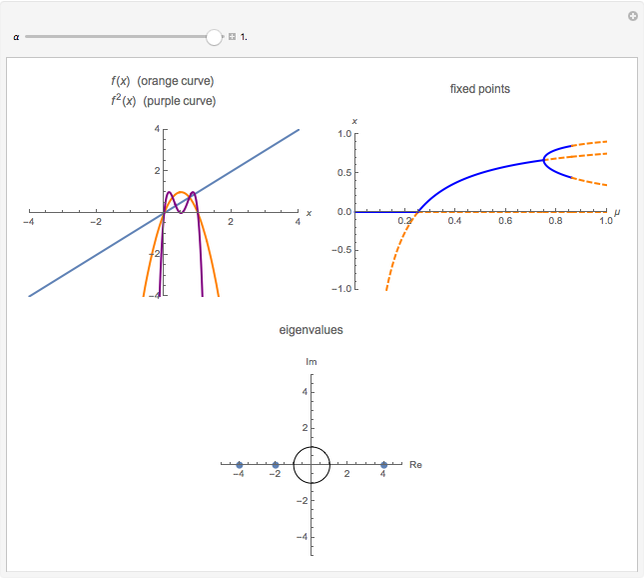
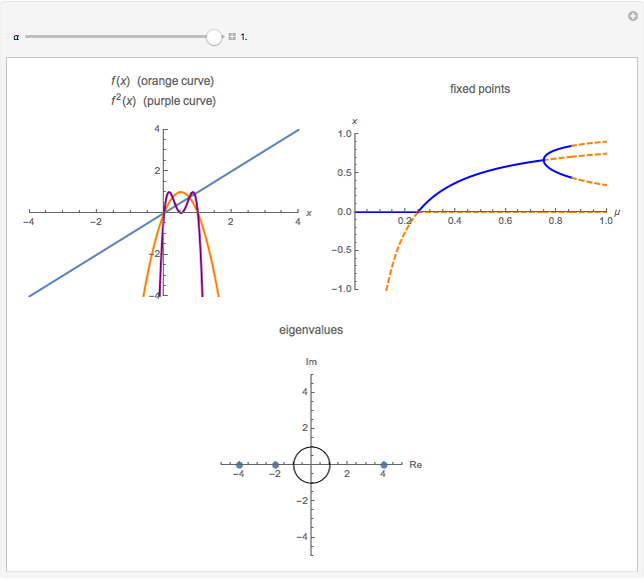
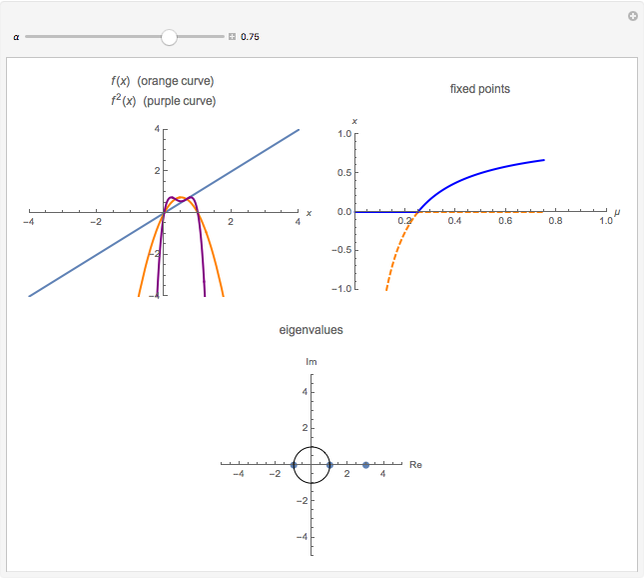
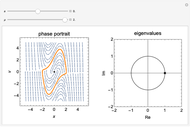
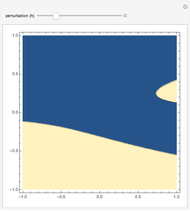
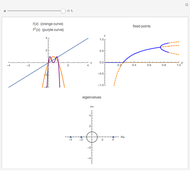
A flip bifurcation occurs when increasing the parameter  causes the graph of the function
causes the graph of the function 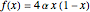 or
or 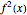 to intersect the line
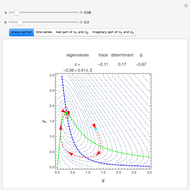
to intersect the line  . See Example 2.32 of [1]. In a flip bifurcation, an eigenvalue leaves the unit circle through the point
. See Example 2.32 of [1]. In a flip bifurcation, an eigenvalue leaves the unit circle through the point 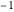 . When this happens, the period two points become stable; thus, this is also known as a period-doubling bifurcation. Varying
. When this happens, the period two points become stable; thus, this is also known as a period-doubling bifurcation. Varying  , the zero solution becomes unstable for
, the zero solution becomes unstable for 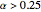 ; the period one blue branch becomes unstable for
; the period one blue branch becomes unstable for 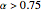 ; the period-doubling bifurcation occurs at
; the period-doubling bifurcation occurs at 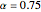 . At the period-doubling bifurcation, the fixed points of
. At the period-doubling bifurcation, the fixed points of 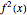 become stable.
become stable.
Contributed by: Edmon Perkins (October 2018)
After work by: Ali Nayfeh and Balakumar Balachandran
Open content licensed under CC BY-NC-SA
Details
Reference
[1] A. H. Nayfeh and B. Balachandran, Applied Nonlinear Dynamics: Analytical, Computational, and Experimental Methods, New York: Wiley, 1995.
Snapshots
Permanent Citation