Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Lines Parallel to the Sides of a Triangle

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

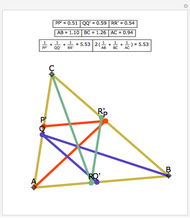
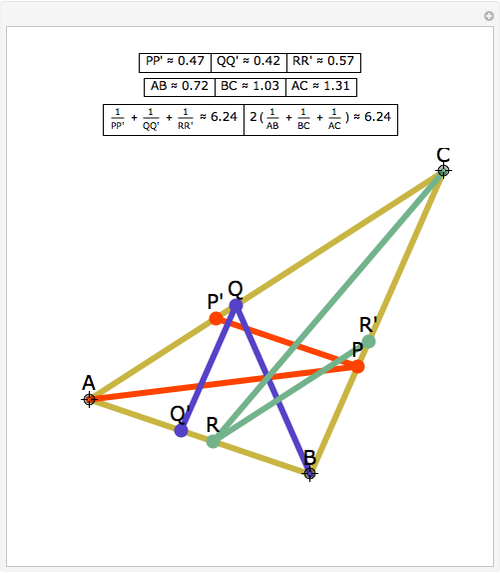
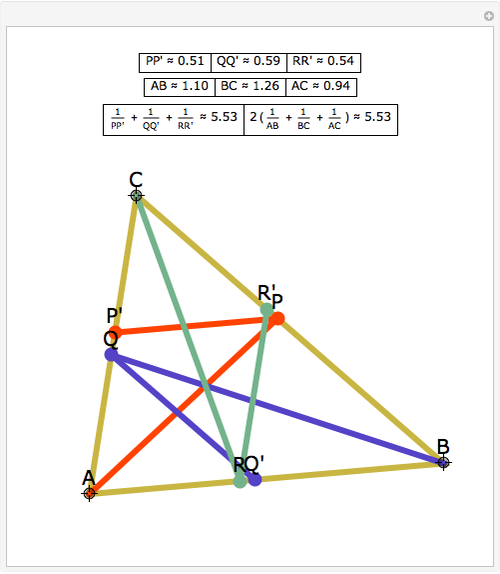
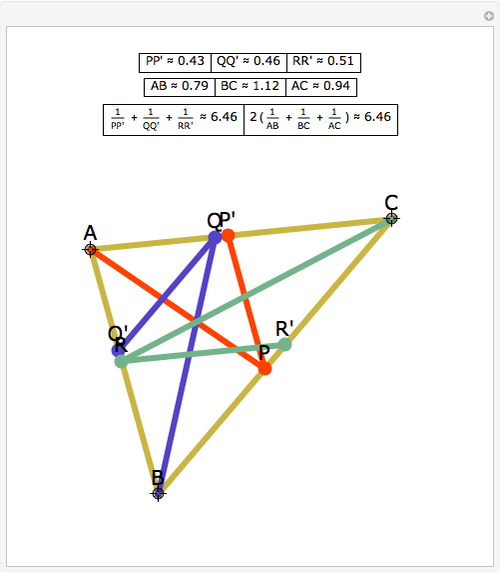
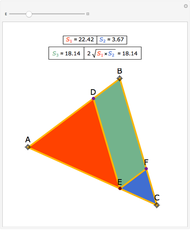
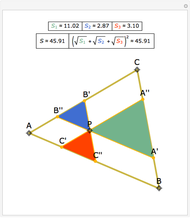
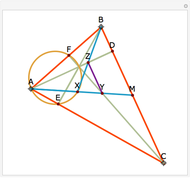
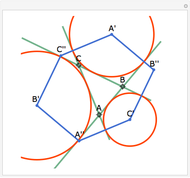
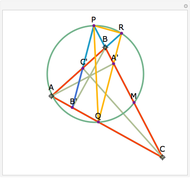
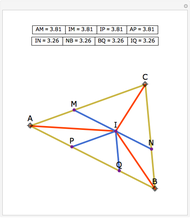
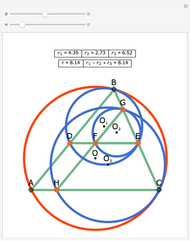
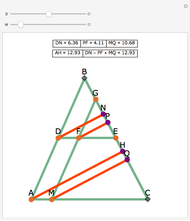
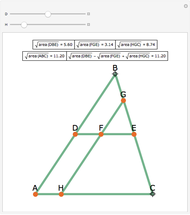
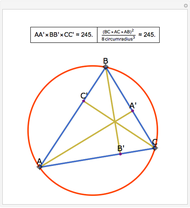
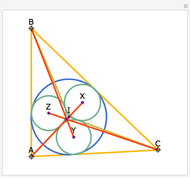
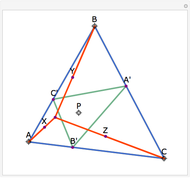
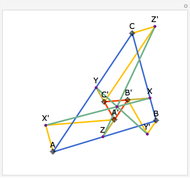
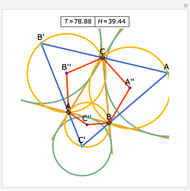
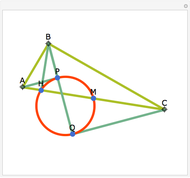
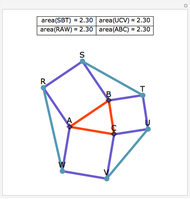
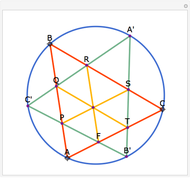
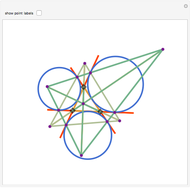
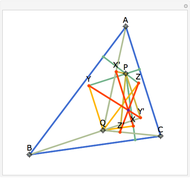
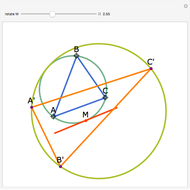
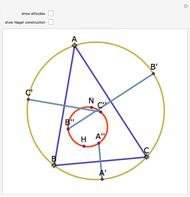
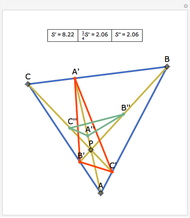
Let ABC be a triangle and let the angle bisectors at A, B, and C intersect the opposite sides at points P, Q, and R. Draw lines from P, Q, and R parallel to AB, BC, and AC that intersect AC, AB, and BC at P', Q', and R'. Then .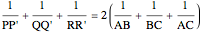

[more]

Drag the points A, B, or C to change the figure.

[less]

Contributed by: Jay Warendorff (March 2011)
After work by: Paul Yiu
Open content licensed under CC BY-NC-SA

Snapshots

Details

The statement of the theorem is in "Notes on Euclidean Geometry" by Paul Yiu, p. 90.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send