Wolfram Demonstrations Project

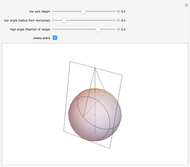
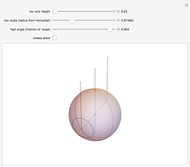
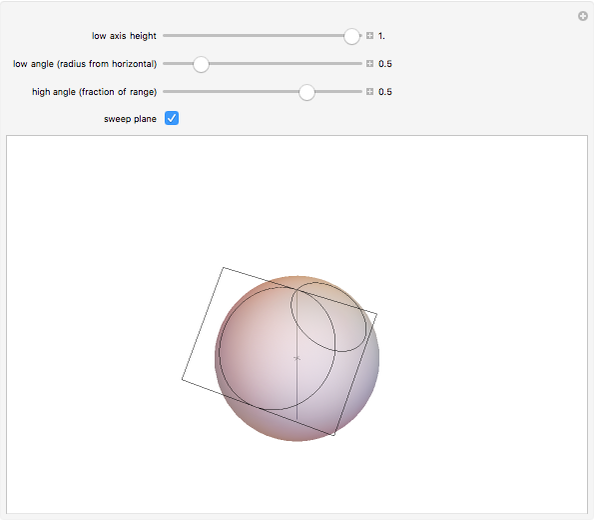
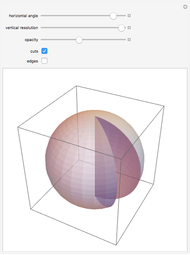
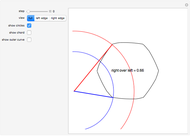
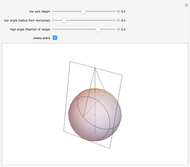
This Demonstration shows two families of circles on the sphere such that each circle in one family is orthogonal to each circle of the other family. In the plane these are called Apollonian circles.

As a special example, consider the circles of latitude (cut by planes perpendicular to the polar axis) and the great circles of longitude (cut by planes containing the polar axis). With the exception of the two poles, every point of the sphere is intersected by exactly one circle from each family and these two circles are orthogonal where they intersect (one circle is north-south at the point and the other is east-west). In this Demonstration, the circles are the intersection of the sphere with planes rotating about two axes.

Contributed by: Mark D. Meyerson

Rotate and Zoom in 3D
Slider Zoom
Gamepad Controls
Automatic Animation

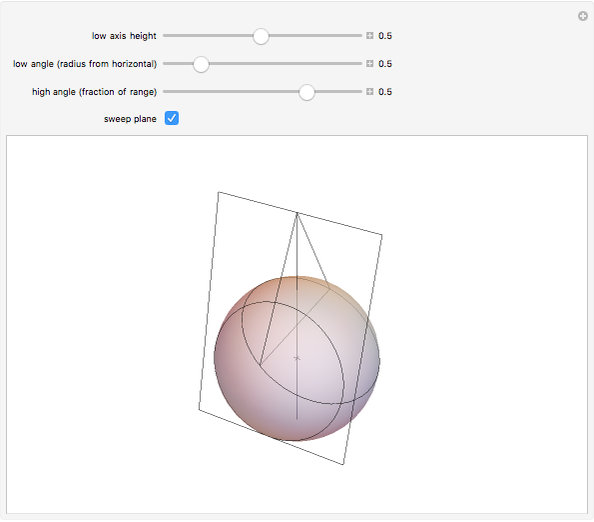
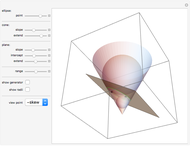
1. Assume that the sphere is centered at the origin and has unit radius. The low axis is a line parallel to the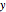

axis through the point

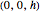with

between 0 and 1. The first family of circles consists of all intersections of the sphere with planes through this line. The high axis is a line parallel to the

axis at a distance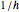

from the origin, cutting the

axis at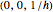

. (If

, then it is viewed as a line at infinity.) The second family of circles is given by the intersections of the sphere with planes through this line. Just as in the latitude/longitude case, with the exception of at most two points, every point of the sphere is intersected by exactly one circle from each family and the two circles are orthogonal at their intersection.

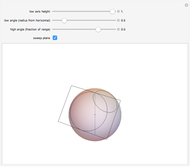
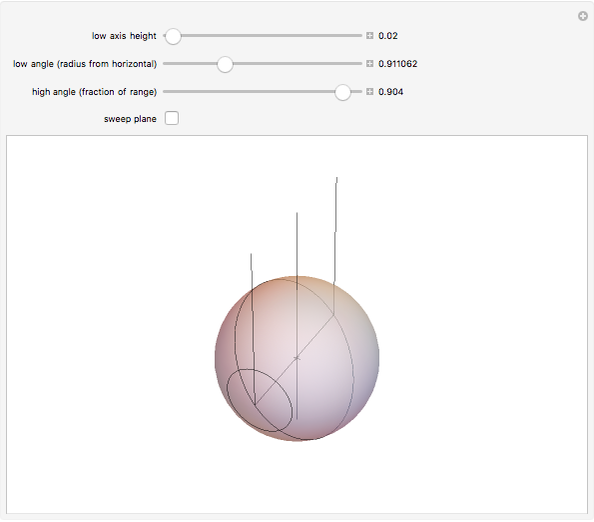
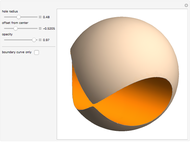
2. There are two special cases. If

, then the second axis is viewed as a line at infinity (snapshot 3). The planes through the axis are perpendicular to the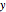

axis, and so this is our original motivating example of circles of latitude and longitude, with the

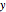axis as the polar axis. The other extreme is when

(snapshot 2). Then the two axes meet at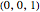

and that is the only point not on only one pair of circles.

3. To view the orthogonality of pairs of circles through a point, drag to rotate the sphere until the intersection point lies over the center of the sphere. Then you are looking straight down onto the plane of tangency at the point of intersection so the angle appears accurate. One can prove this orthogonality with a fairly straightforward use of vectors, cross products, and dot products.

4. In the special case of

, there is an easy way to see perpendicularity. For by symmetry, circles meeting at two points form the same angle at both intersections. At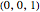

the tangents to the circles are in the direction of the

and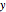

axes, and so are perpendicular. (See snapshot 2.)

5. Apollonian circles in the plane can be found by stereographically projecting these spherical systems. These are also known as coaxial (or coaxal) systems of circles and lines. In particular, the