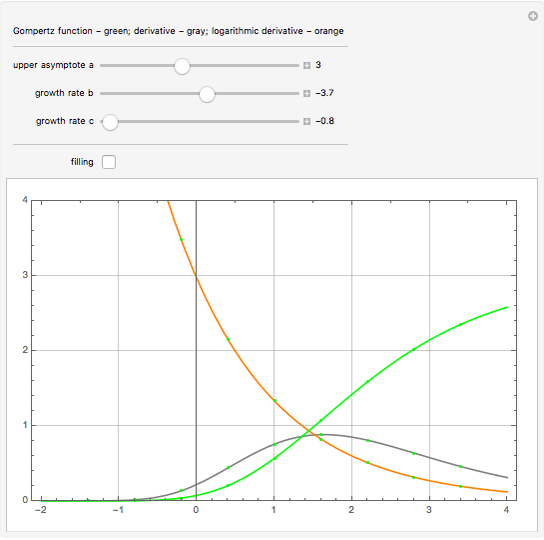
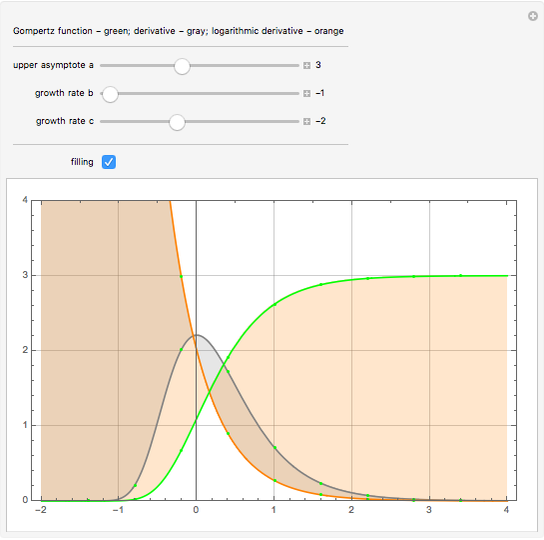
The Gompertz Sigmoid Function and Its Derivative

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
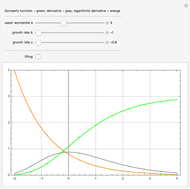
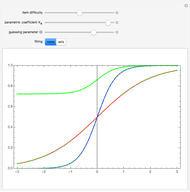
This Gompertz function is defined by 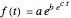 or
or 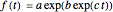 , where
, where  is the upper asymptote and
is the upper asymptote and  and
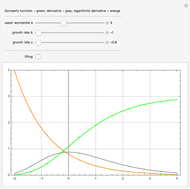
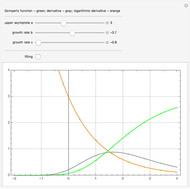
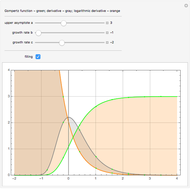
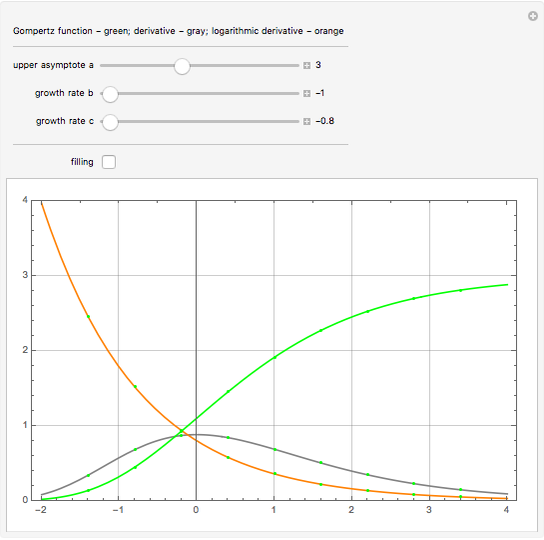
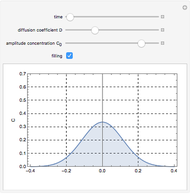
and  are the negative growth rates. The Gompertz function is a sigmoid function. It is a type of mathematical model for a time series, where growth is slowest at the start and end of a time period. This Demonstration plots the Gompertz function
are the negative growth rates. The Gompertz function is a sigmoid function. It is a type of mathematical model for a time series, where growth is slowest at the start and end of a time period. This Demonstration plots the Gompertz function 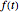 , its derivative,
, its derivative,  , and the ratio
, and the ratio  )=
)=  .
.
Contributed by: Olexandr Eugene Prokopchenko (Ukraine) (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
For more information, see the Wikipedia entry for Gompertz–Makeham Law of Mortality, as well as B. Gompertz, "On the Nature of the Function Expressive of the Law of Human Mortality, and on a New Mode of Determining the Value of Life Contingencies," Philosophical Transactions of the Royal Society of London, 115, 1825 pp. 513–585.
Permanent Citation
"The Gompertz Sigmoid Function and Its Derivative"
http://demonstrations.wolfram.com/TheGompertzSigmoidFunctionAndItsDerivative/
Wolfram Demonstrations Project
Published: March 7 2011