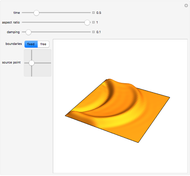
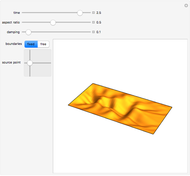
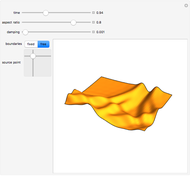
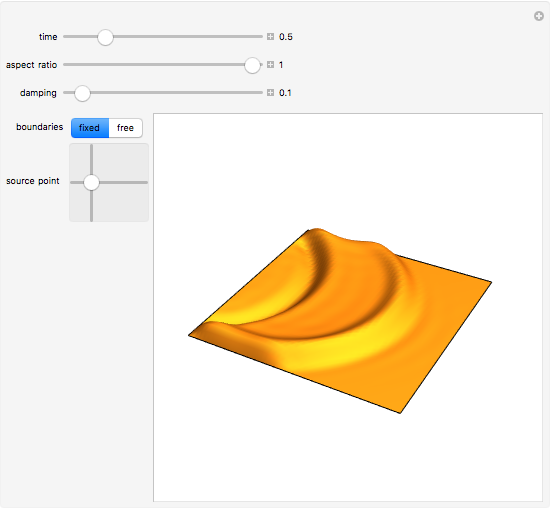
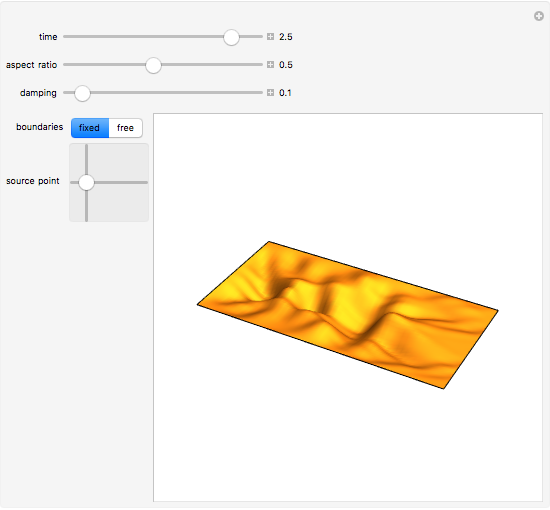
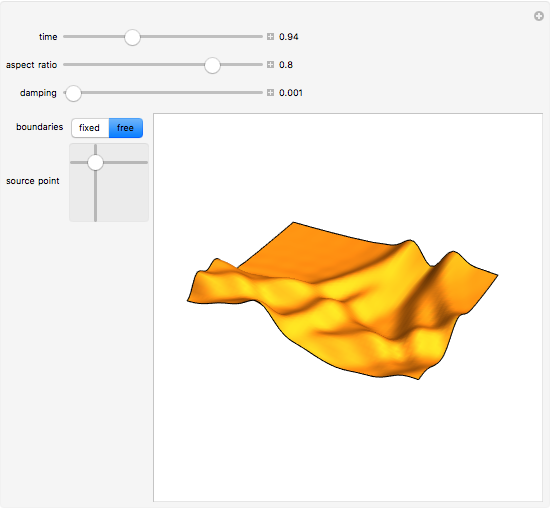
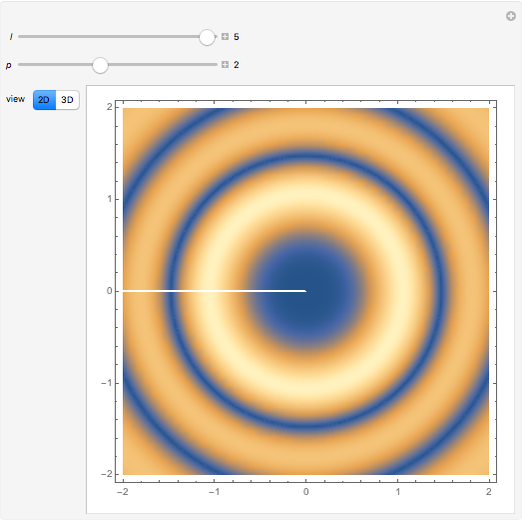
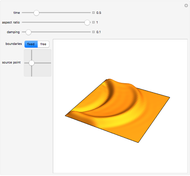
This Demonstration shows the solution of the two-dimensional wave equation subjected to an instantaneous hammer hit centered at the source point location with zero initial displacement and velocity. You can choose free or fixed boundary conditions. A fast and accurate solution was obtained by using the orthogonal function expansion method. By decreasing the aspect ratio, the solution approaches the one-dimensional approximation.