Vibration of a Rectangular Membrane

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
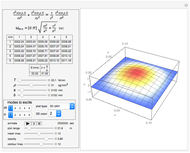
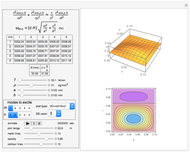
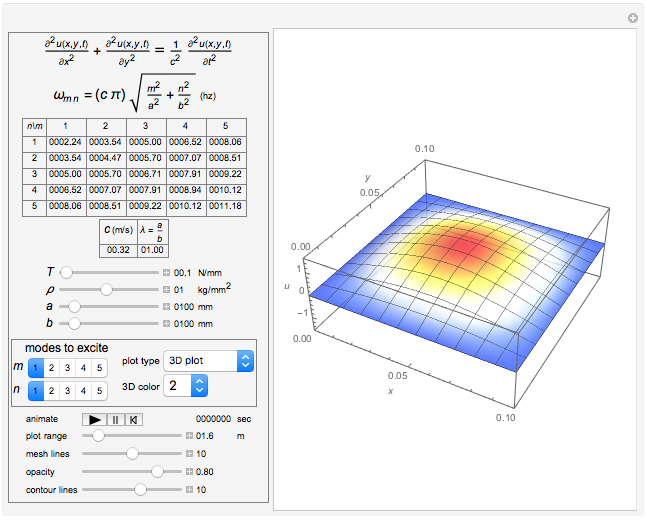
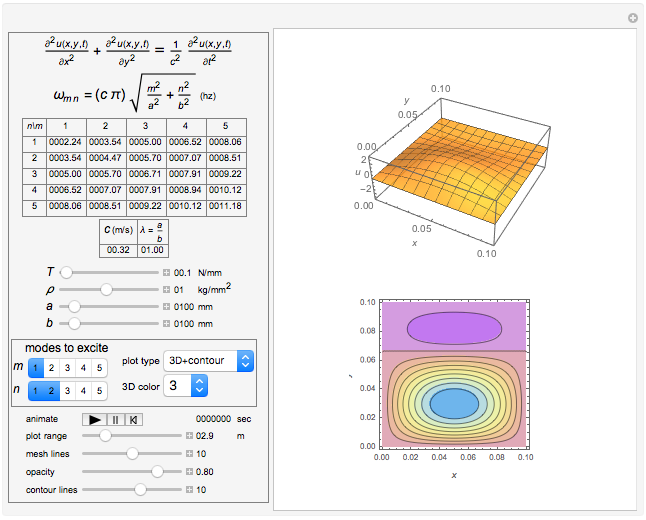
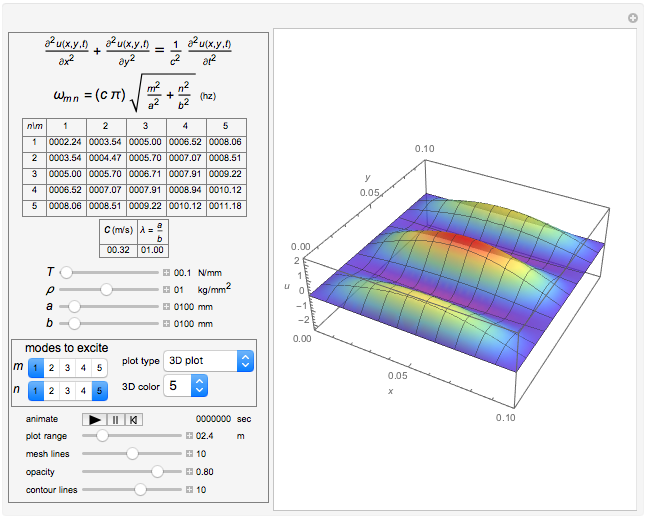
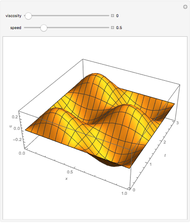
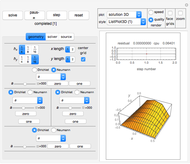
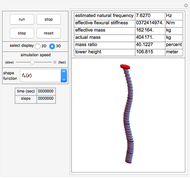
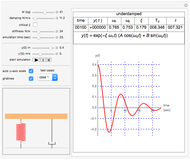
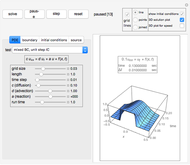
This Demonstration shows the vibration of a 2D membrane for a selected combination of modal vibration shapes. The membrane is fixed along all four edges. You can select any combination of the first five spatial modes  . The fundamental mode is given by
. The fundamental mode is given by  ,
,  . The system obeys the two-dimensional wave equation, given by
. The system obeys the two-dimensional wave equation, given by  , where
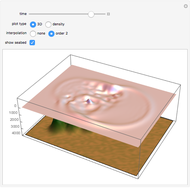
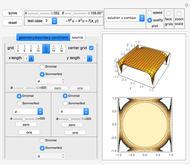
, where  is the amplitude of the membrane's vibration. You can vary the width and length of the membrane using the sliders, the tension, and the surface density, and see the new motion played in time. You can choose a 3D or a contour plot. The contour plot can be used to observe the vibrational modes as it is commonly found in textbook diagrams on this subject.
is the amplitude of the membrane's vibration. You can vary the width and length of the membrane using the sliders, the tension, and the surface density, and see the new motion played in time. You can choose a 3D or a contour plot. The contour plot can be used to observe the vibrational modes as it is commonly found in textbook diagrams on this subject.
Contributed by: Nasser M. Abbasi (September 2013)
Open content licensed under CC BY-NC-SA
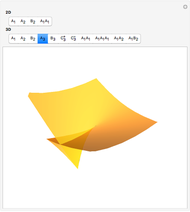
Snapshots
Details
The characteristic frequencies for 2D membrane motion are given by  , where
, where  is the length in the
is the length in the  dimension and
dimension and  is the length in the
is the length in the  direction. These characteristic frequencies or eigenvalues are the frequencies of the membrane's vibrations. The spatial frequencies are given by
direction. These characteristic frequencies or eigenvalues are the frequencies of the membrane's vibrations. The spatial frequencies are given by  and
and  along the
along the  and
and  directions, respectively.
directions, respectively.
The full solution of the PDE is a linear combination of the spatial and time components of the solution obtained by separation of variables and is given by  . The coefficients
. The coefficients  and
and  are found from initial conditions. It simplifies this Demonstration to assume that the initial conditions (position and velocity of the membrane) are such that
are found from initial conditions. It simplifies this Demonstration to assume that the initial conditions (position and velocity of the membrane) are such that  and
and  . Hence the solution becomes
. Hence the solution becomes  and this is the solution that is animated.
and this is the solution that is animated.
This Demonstration supports modes up to  and
and  . You select the parameter values of
. You select the parameter values of  ,
,  ,
,  , and
, and  from the sliders and see the resulting vibrations. The wave speed is
from the sliders and see the resulting vibrations. The wave speed is  , where
, where  is the tension the membrane bears per unit length of its boundary. Hence
is the tension the membrane bears per unit length of its boundary. Hence  has units of
has units of  and
and  is the membrane mass per unit of surface area; therefore
is the membrane mass per unit of surface area; therefore  has units of
has units of  per
per  . The parameter
. The parameter  represents the wave speed (in the transverse direction) in units of
represents the wave speed (in the transverse direction) in units of  per
per  .
.
Tension is assumed constant, gravity is ignored, and no damping is assumed.
The tension and density parameters are expressed in  and internally converted to the SI unit of meters.
and internally converted to the SI unit of meters.
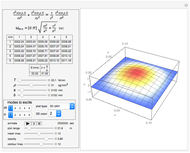
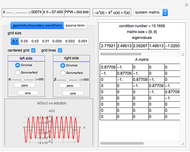
A table of the characteristic frequencies  is on the left in units of Hz. You select the modes to excite by using the dialog shown on the left. A mode is selected and unselected by pressing on the button specific for that mode. Mouseover the 3D plot to see the full analytical solution using the selected modes.
is on the left in units of Hz. You select the modes to excite by using the dialog shown on the left. A mode is selected and unselected by pressing on the button specific for that mode. Mouseover the 3D plot to see the full analytical solution using the selected modes.
The membrane is fixed on all four edges.
References
[1] R. D. Belvins, Formula for Natural Frequency and Mode Shape, New York: Van Nostrand, 1979.
[2] R. Engelstad. "ME 740, Advanced Vibration." Class lecture, University of Wisconsin-Madison, Madison, WI, April 2, 2013.
[3] H. Esoy, "Free Vibration Analysis of Rectangular Membranes with Variable Density Using the Discrete Singular Convolution Approach," Asian Journal of Civil Engineering (Building and Housing), 11(1), 2010 pp. 83–94.
Permanent Citation