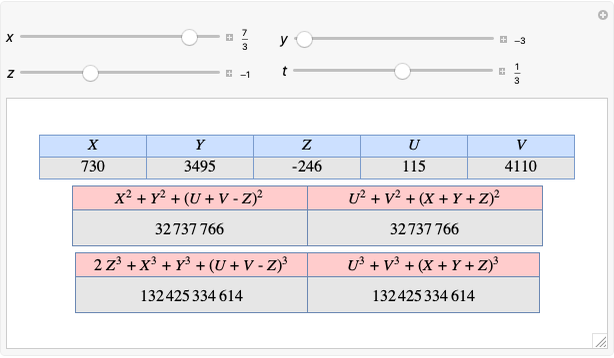
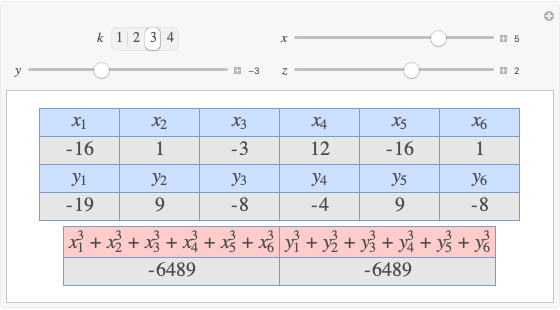
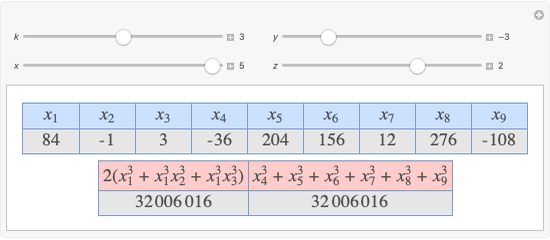
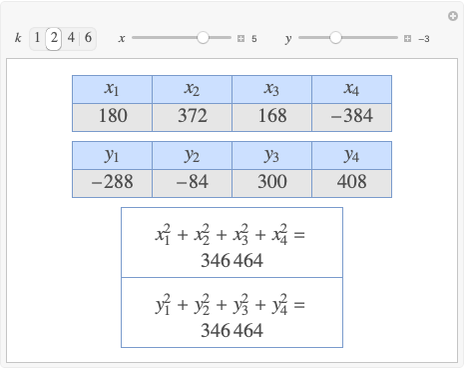
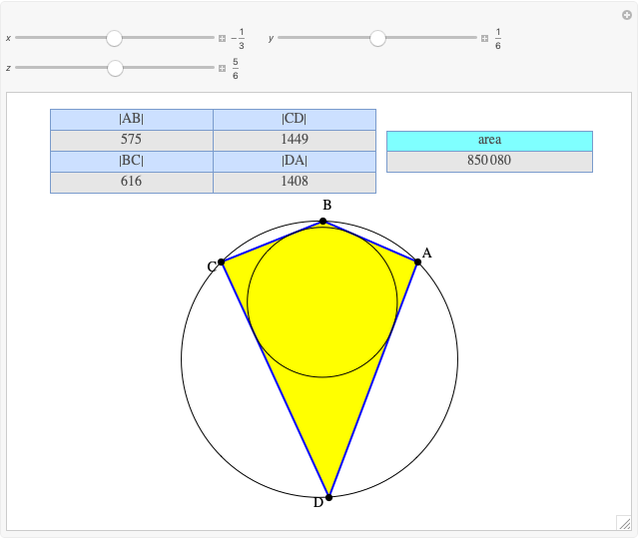
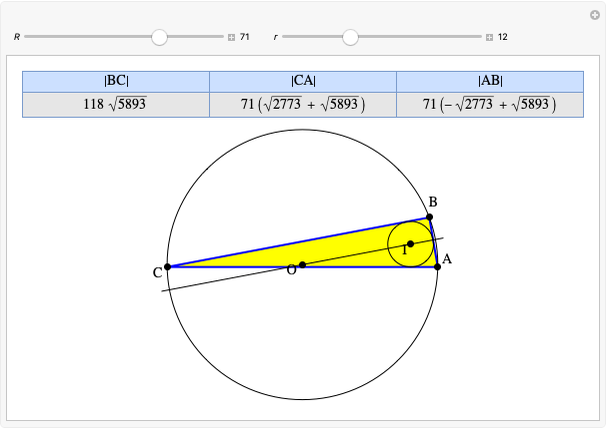
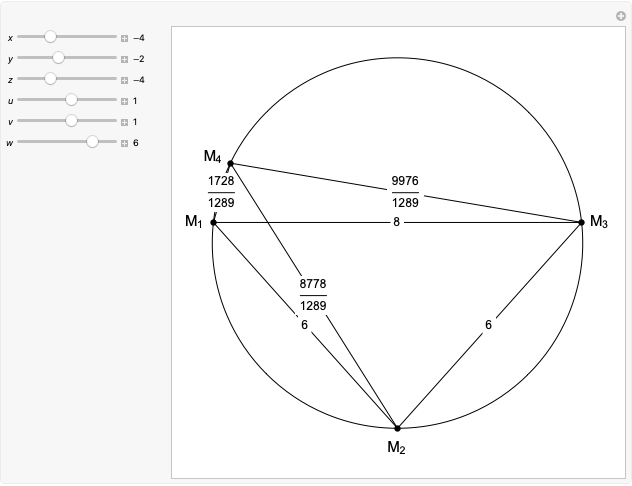
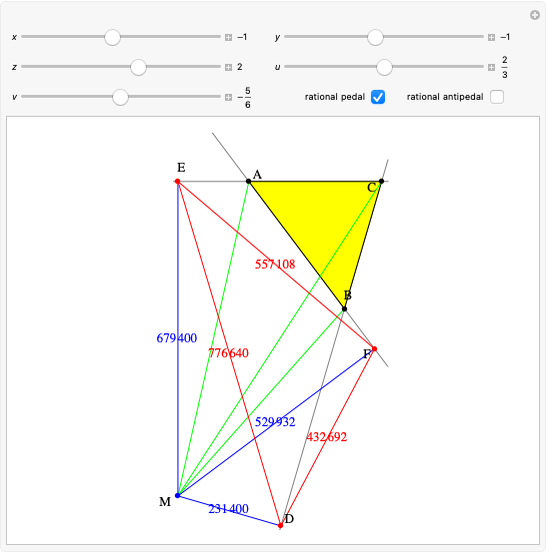
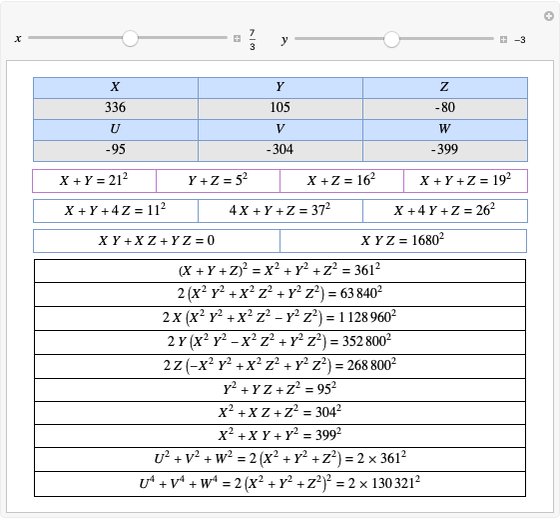
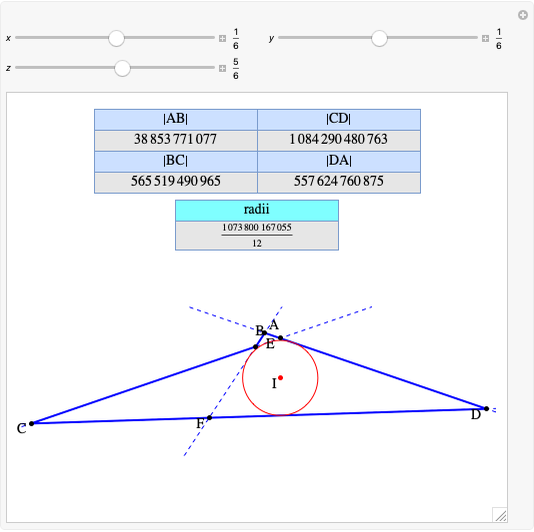
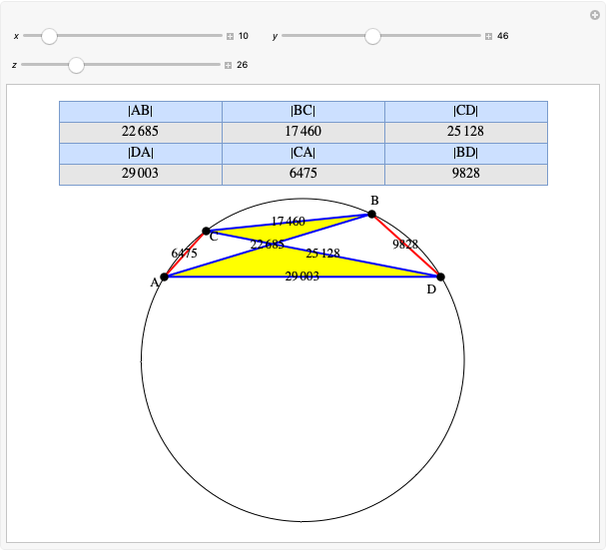
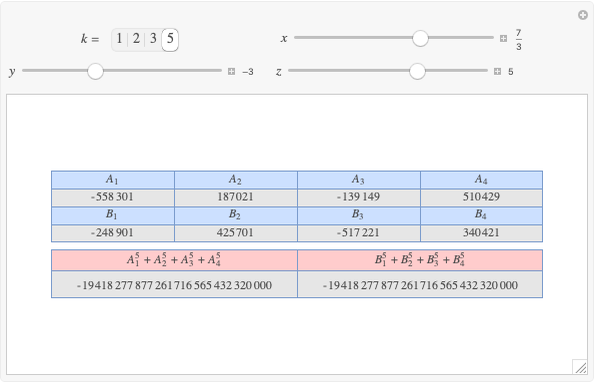
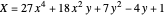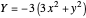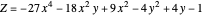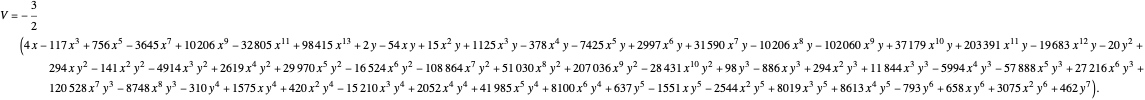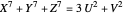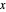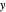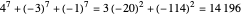Algebraic Identity with Squares and Seventh Powers

Initializing live version

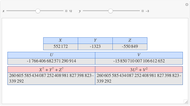
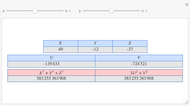
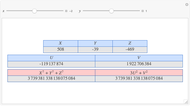
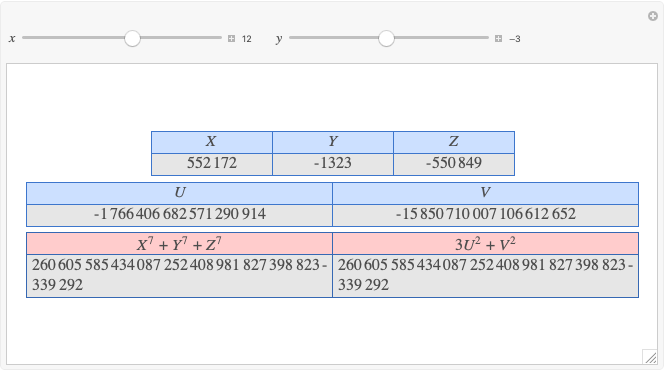
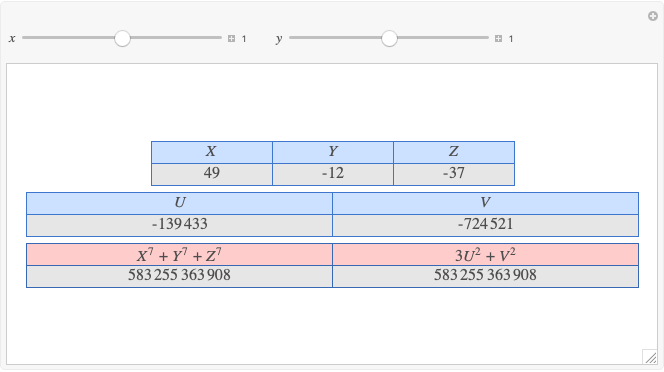
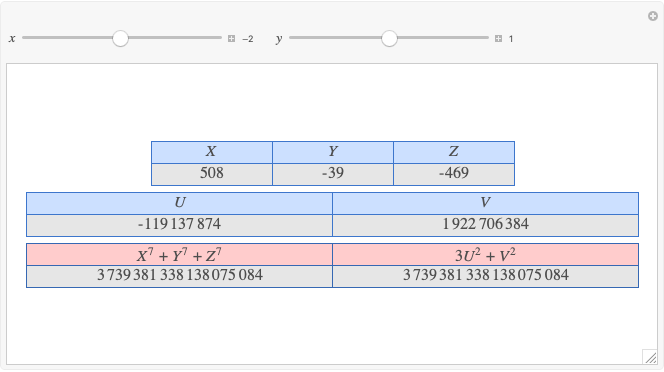
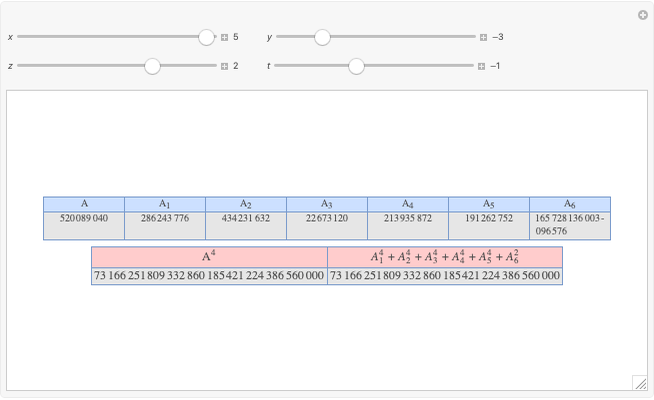
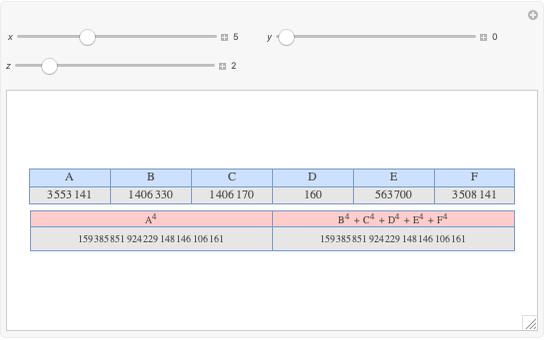
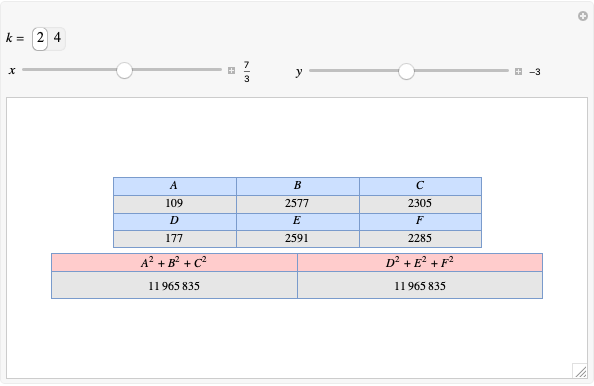
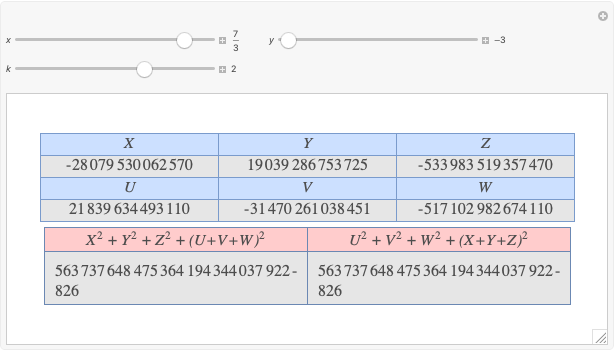
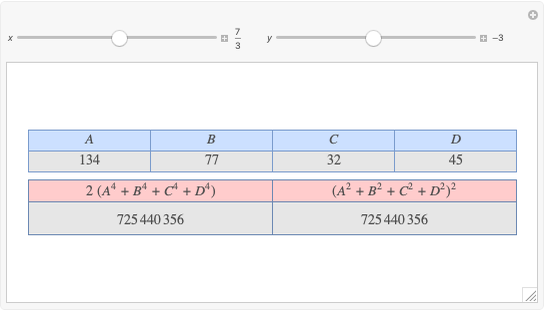
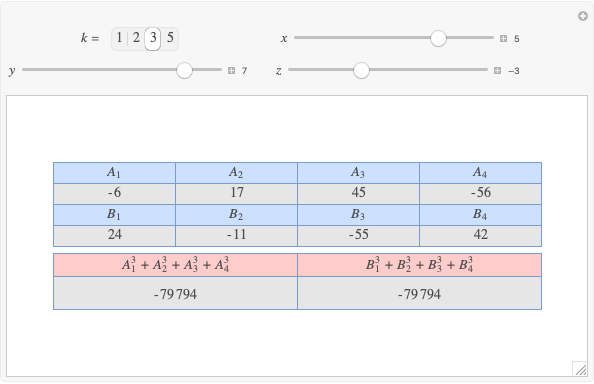
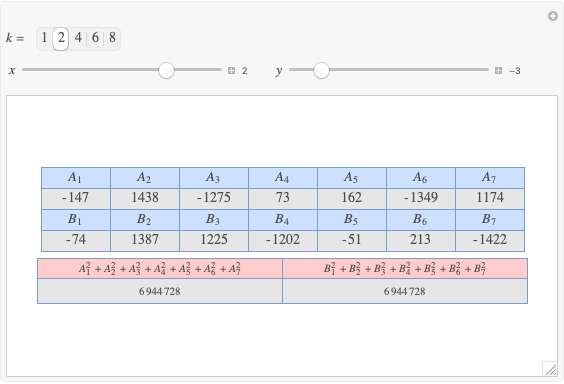
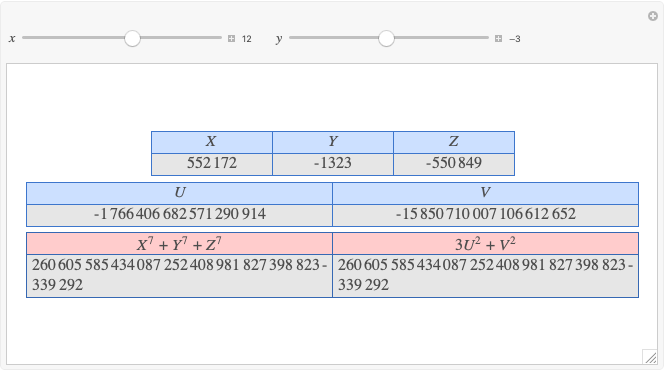
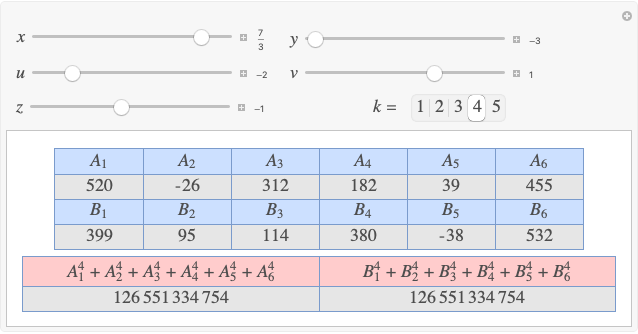
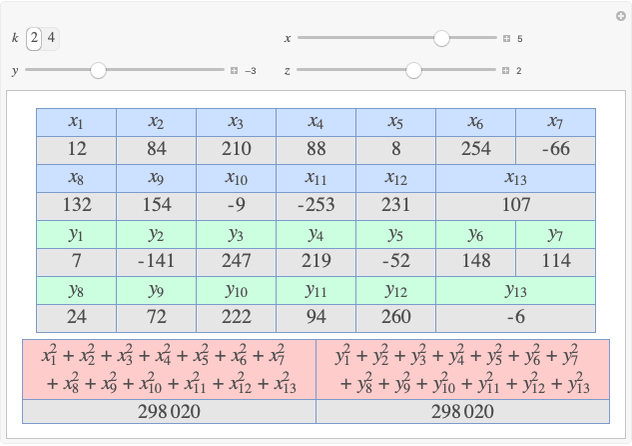
Let 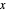, 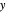be two arbitrary complex numbers. Then set

Contributed by: Minh Trinh Xuan (June 14)
Open content licensed under CC BY-NC-SA

Details


Feedback (field required)

Email (field required)	Name

Occupation	Organization

Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback. Send