An Oscillating Pendulum

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
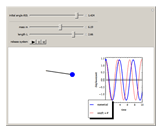
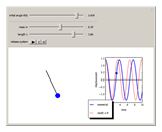
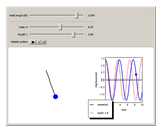
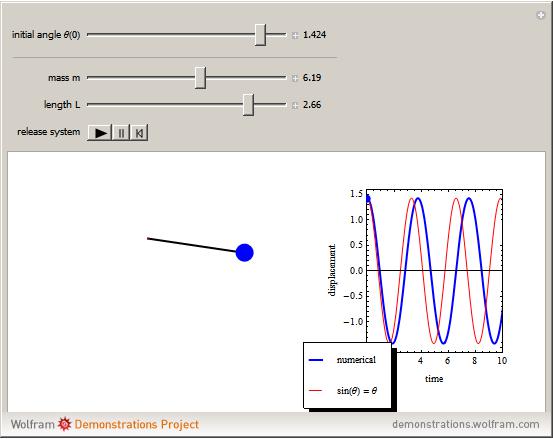
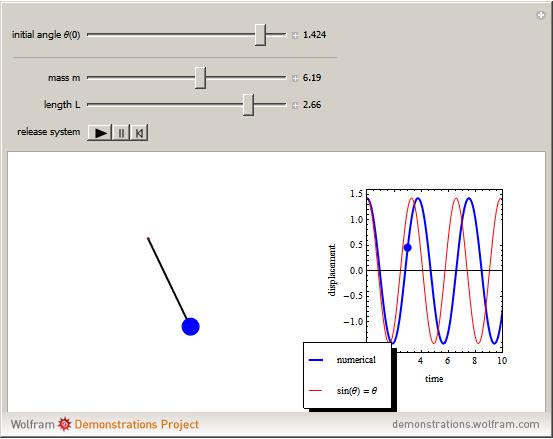
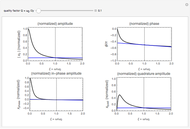
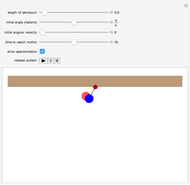
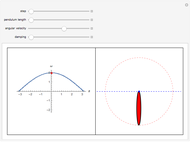
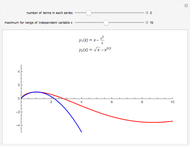
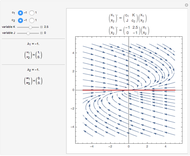
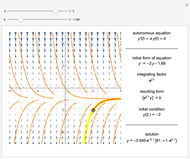
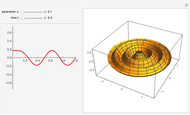
The equation for a swinging pendulum is  , where
, where  is the angle of the pendulum at time
is the angle of the pendulum at time  ,
,  is the acceleration due to gravity, and
is the acceleration due to gravity, and  is the length of the pendulum arm. The linearized approximation replaces
is the length of the pendulum arm. The linearized approximation replaces  by
by  , which is valid for small
, which is valid for small  .
.
Contributed by: Stephen Wilkerson (March 2011)
(United States Military Academy West Point, Department of Mathematics)
Open content licensed under CC BY-NC-SA
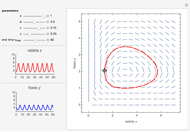
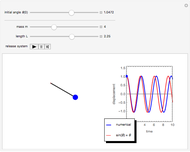
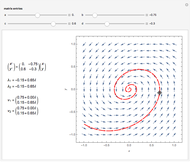
Snapshots
Details
This is the example An Oscillating Pendulum from [1], Section 1.4, Modeling with First Order Equations. The equation can be solved numerically using NDSolve in Mathematica.
Reference
[1] J. R. Brannan and W. E. Boyce, Differential Equations with Boundary Value Problems: An Introduction to Modern Methods and Applications, New York: John Wiley and Sons, 2010.
Permanent Citation