Predator-Prey Model

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
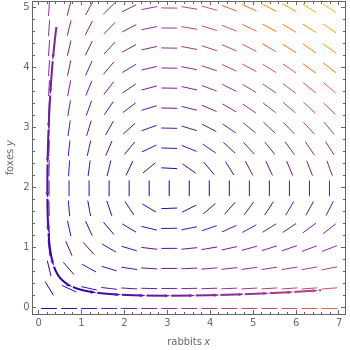
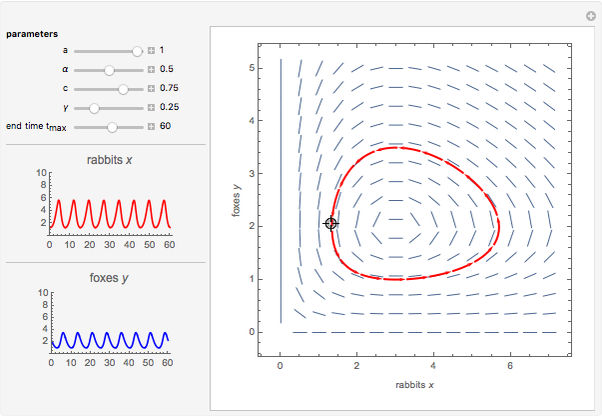
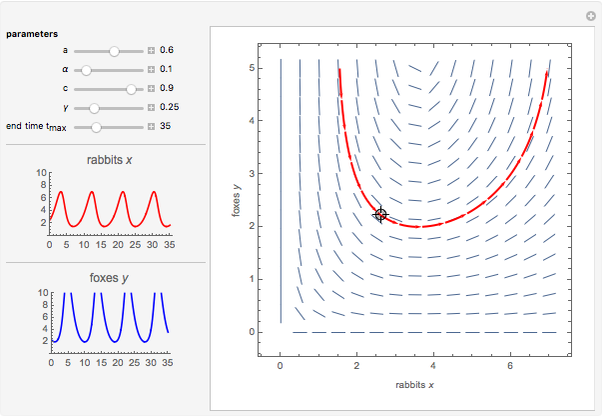
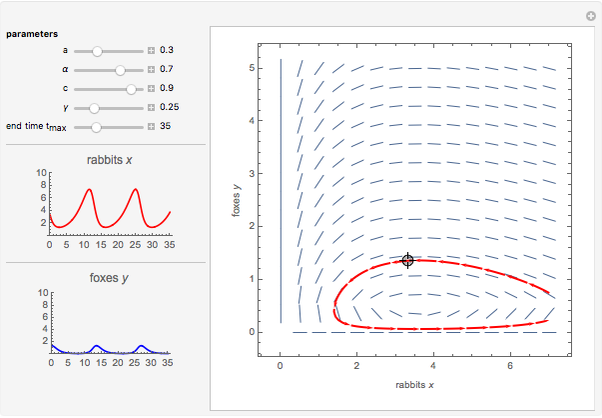
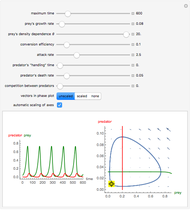
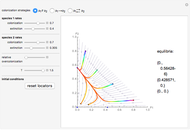
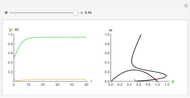
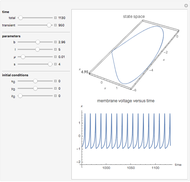
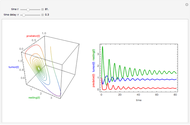
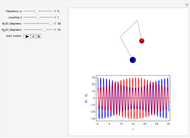
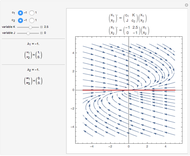
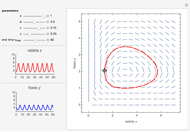
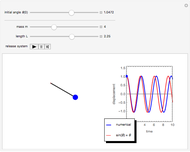
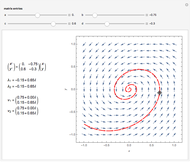
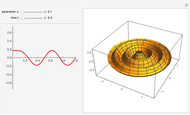
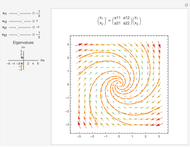
This Demonstration illustrates the predator-prey model with two species, foxes and rabbits. Foxes prey on rabbits that live on vegetation. The rabbit population is  and the fox population is
and the fox population is  ; both depend on time
; both depend on time  .
.
Contributed by: Stephen Wilkerson (March 2011)
(United States Military Academy West Point, Department of Mathematics)
Open content licensed under CC BY-NC-SA
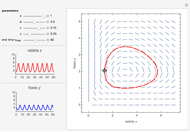
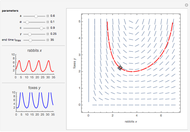
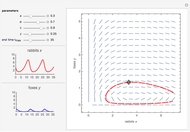
Snapshots
Details
This example comes from [1], Section 7.4, Predator Prey Equations.
Reference:
[1] J. R. Brannan and W. E. Boyce, Differential Equations with Boundary Value Problems: An Introduction to Modern Methods and Applications, New York: John Wiley and Sons, 2010.
Permanent Citation