Bound States of a Finite Potential Well

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
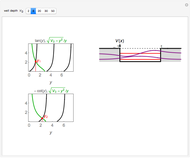
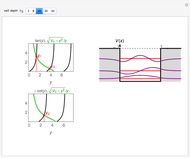
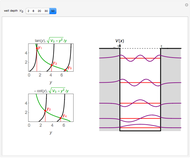
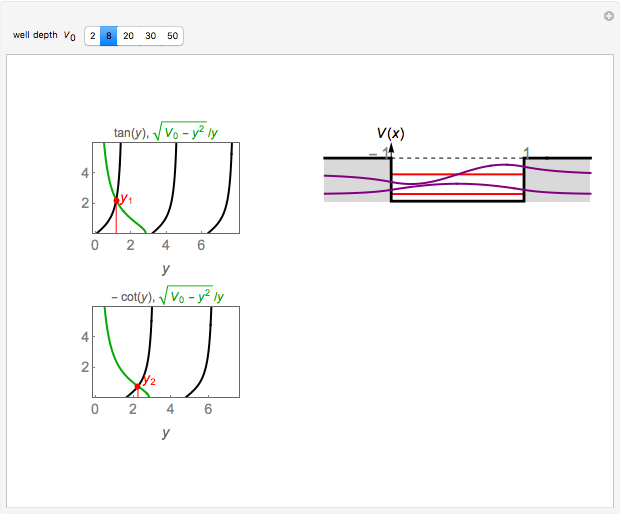
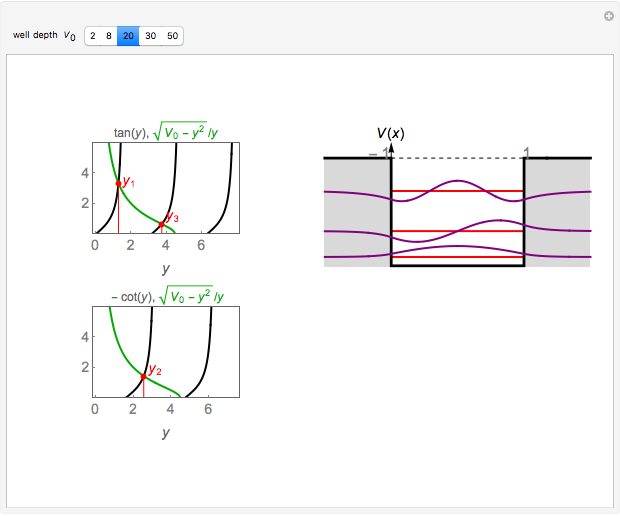
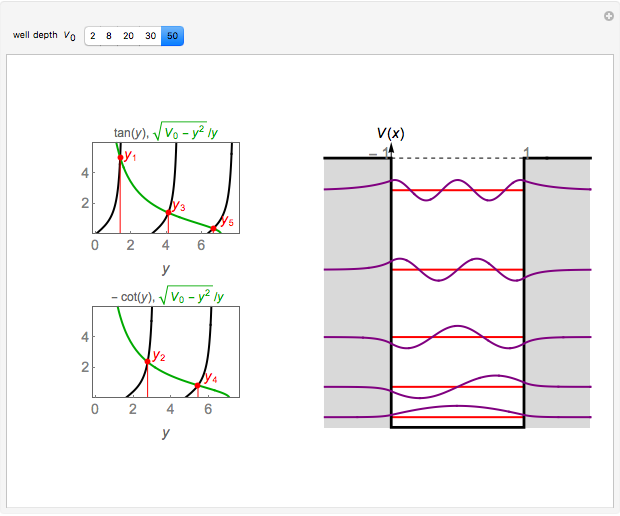
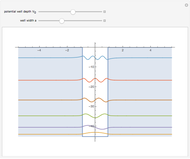
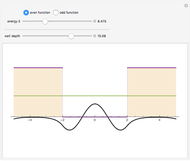
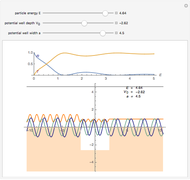
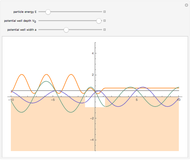
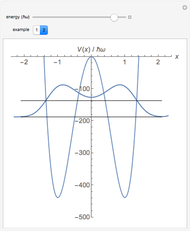
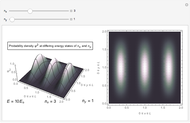
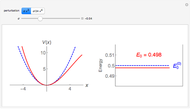
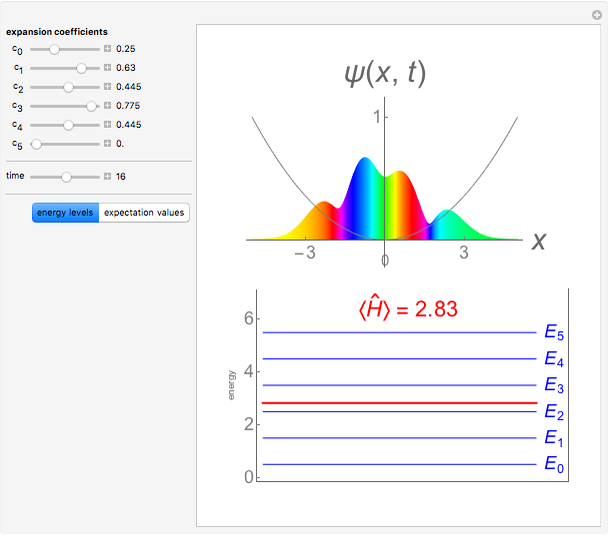
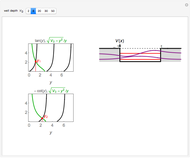
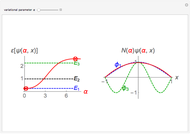
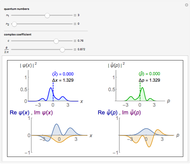
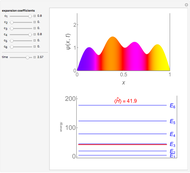
This Demonstration shows the bound state energy levels and eigenfunctions for a square finite potential well defined by  . The solutions are obtained by solving the time-independent Schrödinger equation in each region and requiring continuity of both the wavefunction and its first derivative. This leads to two transcendental equations,
. The solutions are obtained by solving the time-independent Schrödinger equation in each region and requiring continuity of both the wavefunction and its first derivative. This leads to two transcendental equations,  and
and  , where
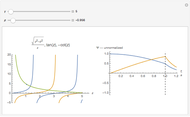
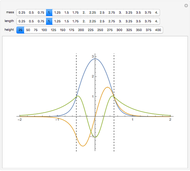
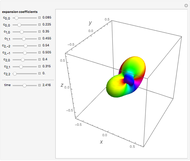
, where  . The graphical solutions to these equations give the bound state energy levels, shown on the left. The dependence of the energy levels and the corresponding eigenfunctions on well depth are shown on the right.
. The graphical solutions to these equations give the bound state energy levels, shown on the left. The dependence of the energy levels and the corresponding eigenfunctions on well depth are shown on the right.
Contributed by: Porscha McRobbie and Eitan Geva (March 2011)
Open content licensed under CC BY-NC-SA
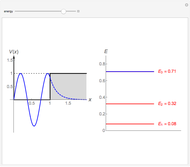
Snapshots
Details
P. T. Tam, A Physicist's Guide to Mathematica, 2nd ed., Burlington, MA: Academic Press, 2008.
Permanent Citation