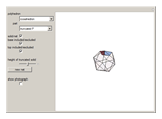
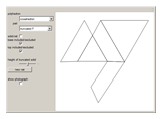
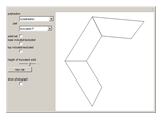
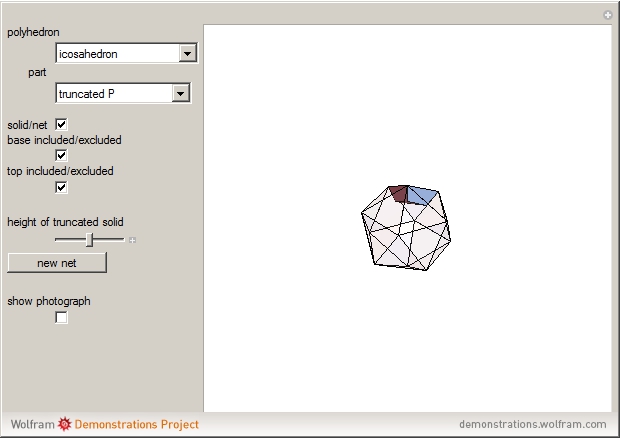
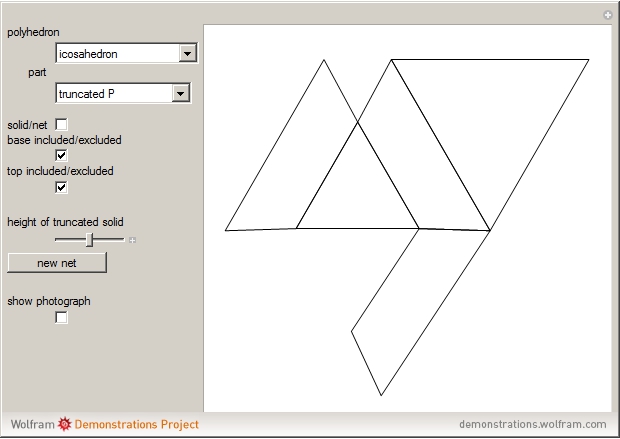
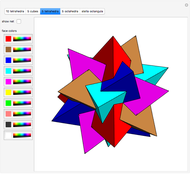
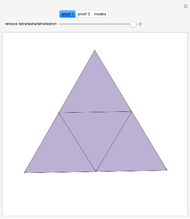
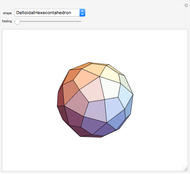
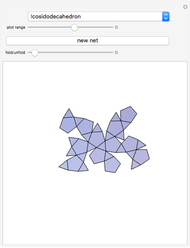
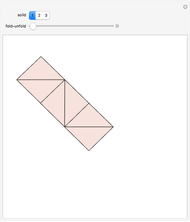
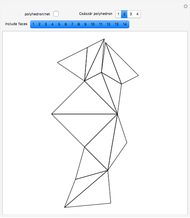
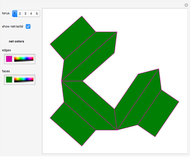
This Demonstration shows the five regular solids (or Platonic solids), a rhombic dodecahedron, and a rhombic triacontahedron. You can see a (possibly truncated) pyramid with vertex at the center of the polyhedron and base that is a face of the polyhedron. You can also see the fundamental region of a triangular pyramid. You can use a net to construct certain kaleidoscopes with three, four, or five mirrors.