Cournot and Stackelberg Equilibria and Best Responses

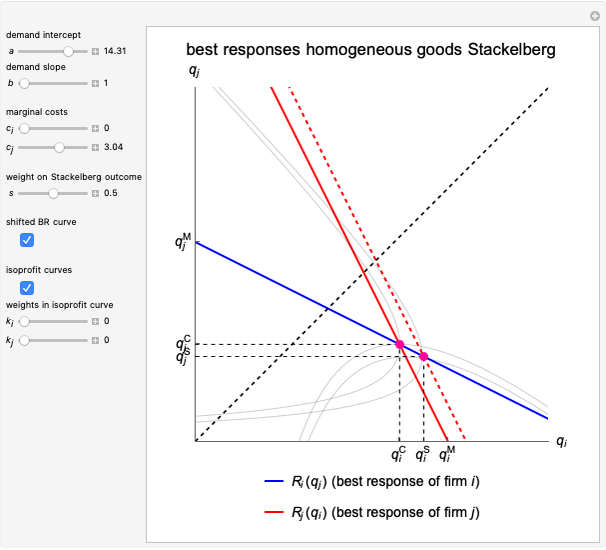
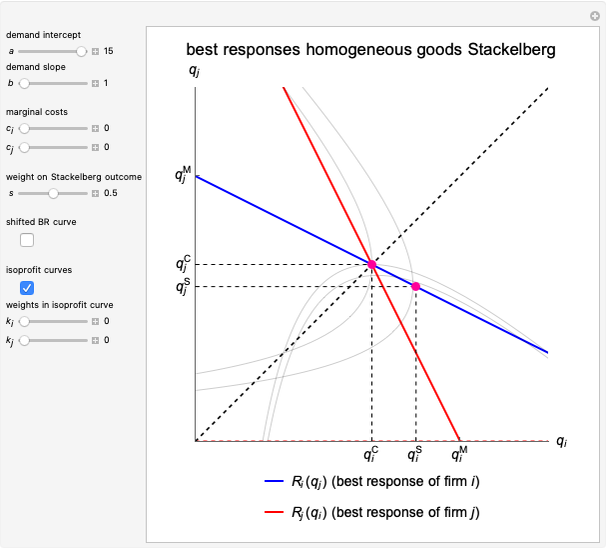
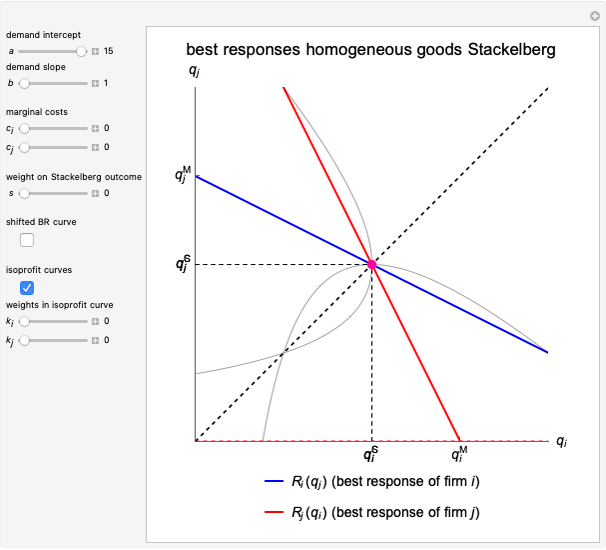
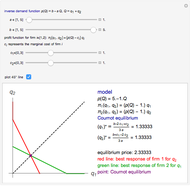
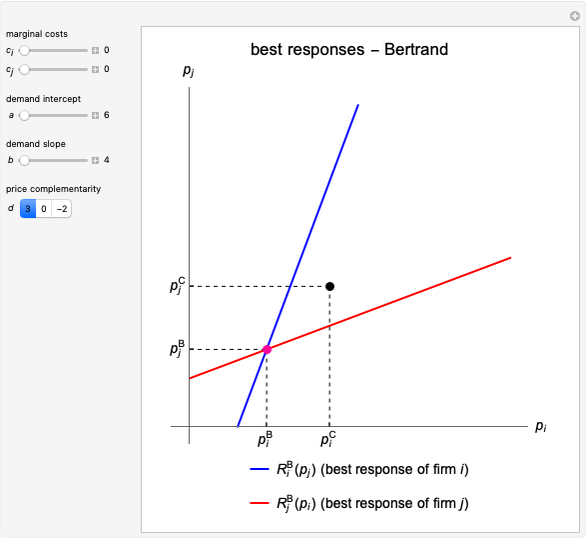
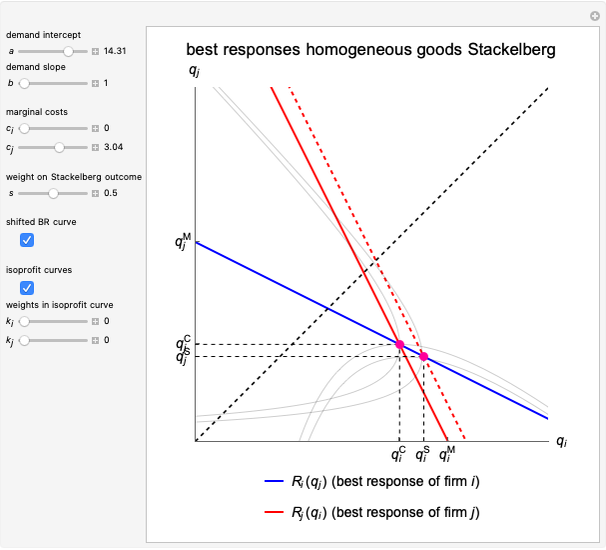
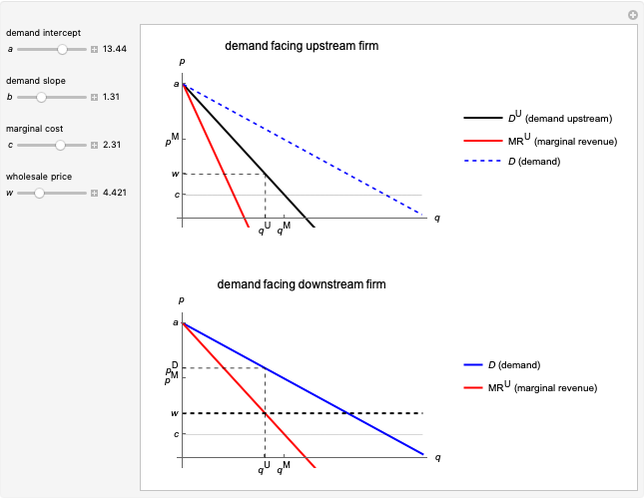
This Demonstration illustrates a number of features of the homogeneous goods Cournot and Stackelberg duopoly model [1, Chapter 4] as opposed to the Singh and Vives model of product differentiation under Bertrand competition [2], which is treated in the Duopoly Competition with Differentiated Products Demonstration. The market has inverse demand function 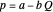and the firms produce at constant marginal costs 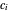and 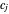, respectively. The graph illustrates the best response curves of firm (blue, Stackelberg dotted) and firm (red). The intersections are the Cournot equilibrium point and the Stackelberg equilibrium point. Note that the Stackelberg quantity equilibria are always below the Cournot equilibria and coincide when the weight on the Stackelberg outcome is zero. The demand parameters are 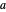(demand intercept) and (demand slope). The parameters 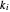and 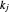are the weights on the monopoly points in the isoprofit curves. That is, for 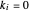, the graph shows the isoprofit curve reached by firm in the Cournot equilibrium. For 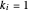, the graph shows the isoprofit curve for firm in the monopoly point, the point preferred by firm . The slider 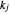does the same for firm .