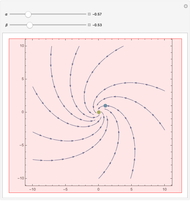
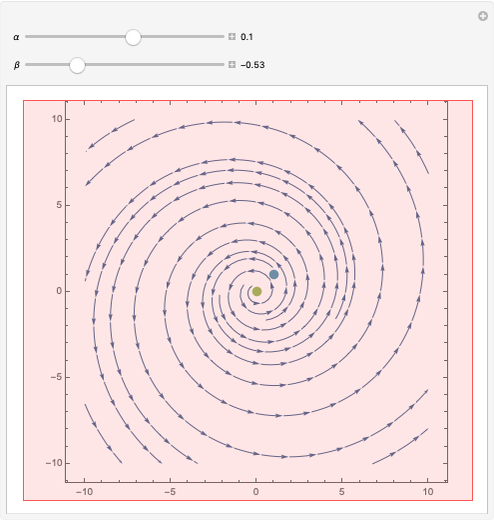
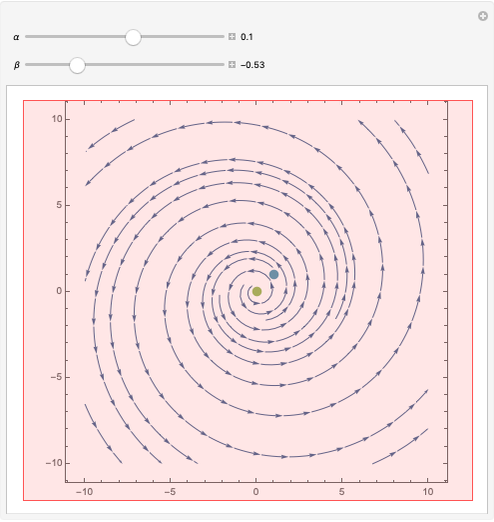
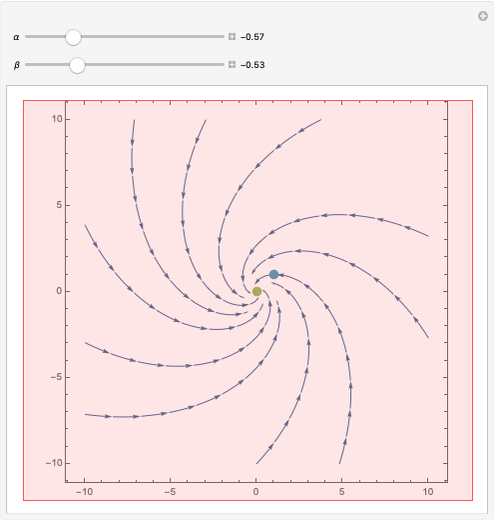
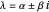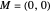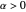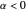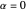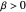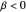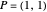Dynamic Behavior of a Simple Canonical System
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
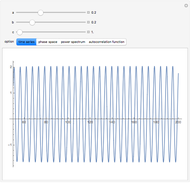
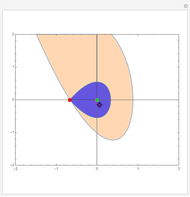
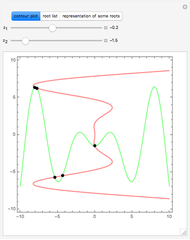
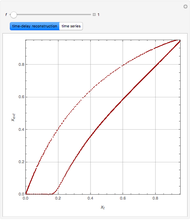
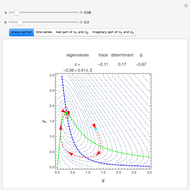
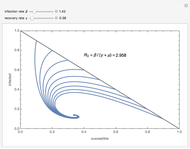
Consider the following system of ODEs:
[more]
Contributed by: Housam Binous (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
S. Lynch, Dynamical Systems with Applications using Mathematica, Boston: Birkhäuser, 2007.
Permanent Citation