GL(2,p) and GL(3,3) Acting on Points

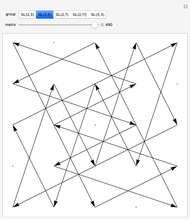
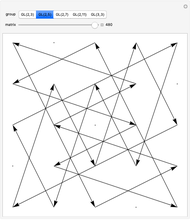
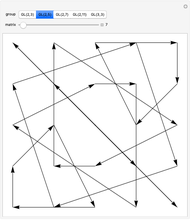
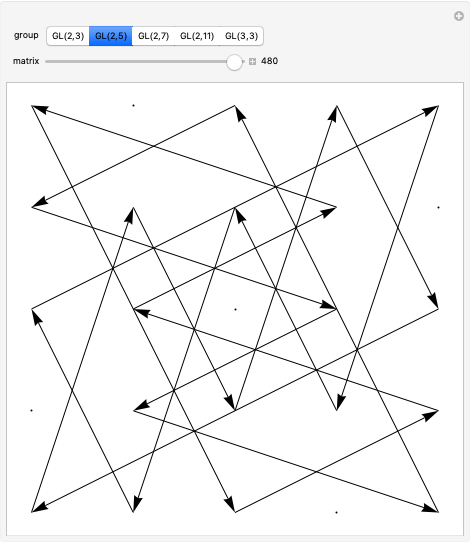
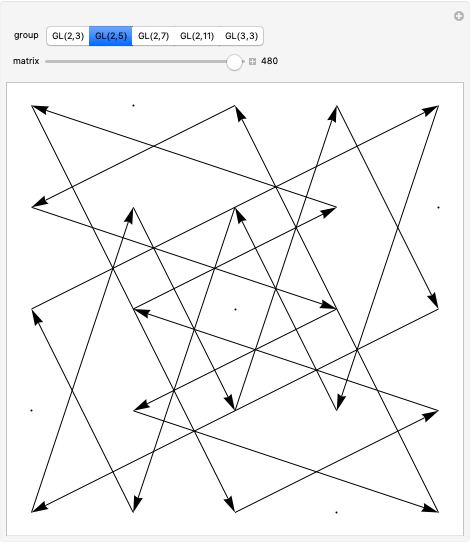
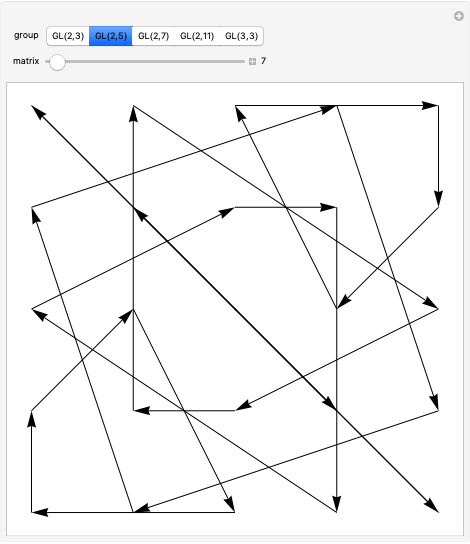
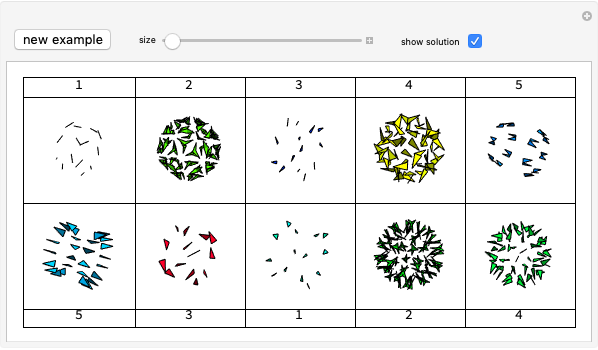
The two-dimensional space 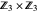contains nine points: (0,0), (0,1), (0,2), (1,0), (1,1), (1,2), (2,0), (2,1), and (2,2). The 48 invertible 2×2 matrices over 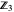form the general linear group known as 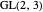. They act on 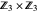by matrix multiplication modulo 3, permuting the nine points. More generally, 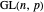is the set of invertible 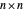matrices over the field 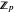, where 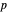is prime With (0,0) shifted to the center, the matrix actions on the nine points make symmetrical patterns.