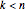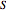Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

-Closure of a Directed -Cycle Graph

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

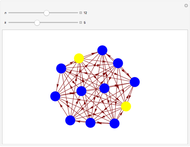
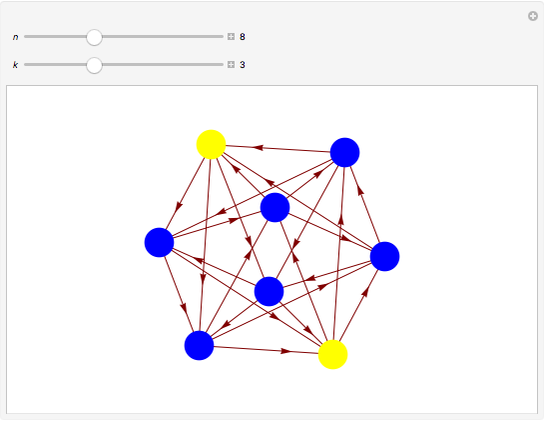
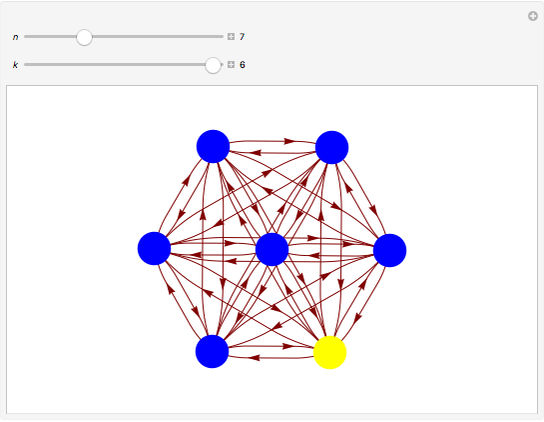
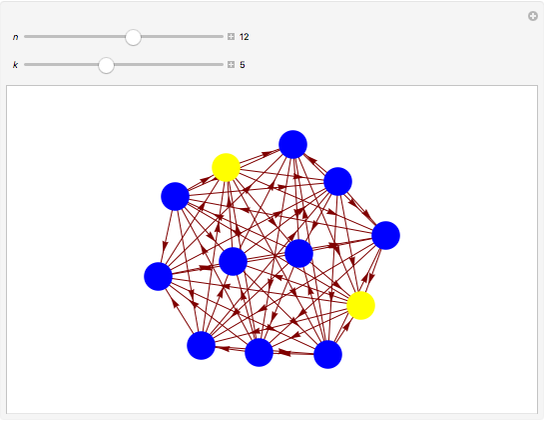
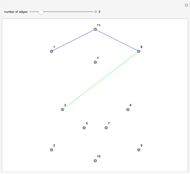
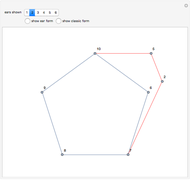
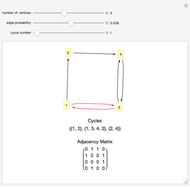
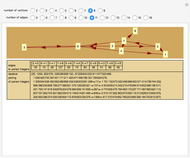
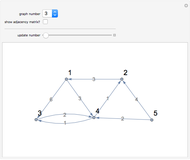
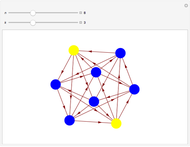
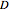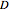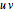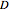The -closure of a digraph is a digraph with the same vertex set as and an arc set that is the set of arcs where and are vertices of such that there is a 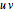-path in 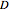of length less than or equal to .

[more]

This Demonstration draws a directed cycle with vertices. Then it draws its -closure for a given value of . When divides , there is a set that is kernel of the -closure, and therefore is a -kernel of the original cycle. When divides , one kernel of the closure is colored in yellow.

[less]

Contributed by: Sebastian Gonzalez Hermosillo de la Maza (December 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send