Knopp's Osgood Curve Construction

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
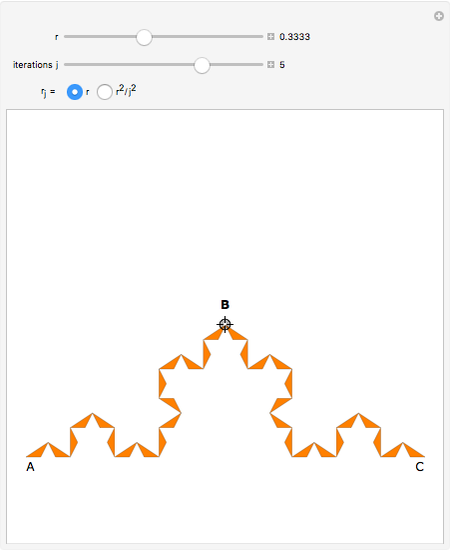
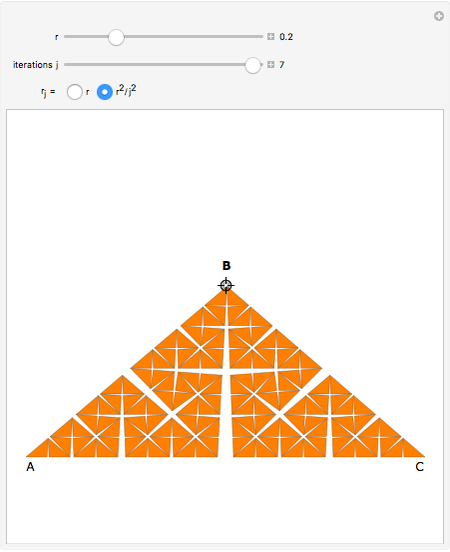
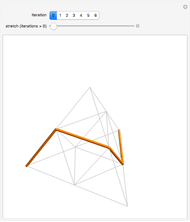
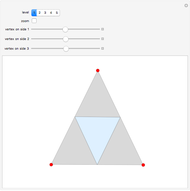
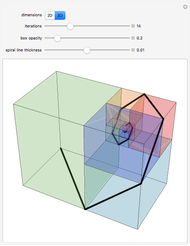
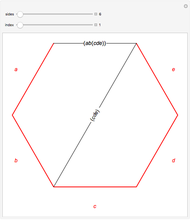
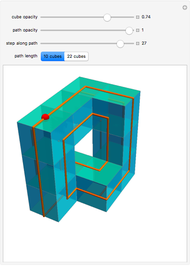
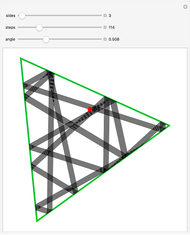
Starting with a triangle, remove a triangle-shaped region in such a way that two triangles remain, where the ratio of the removed triangle area to the original triangle area is  . Repeating the process on the two remaining triangles—removing a proportion of area
. Repeating the process on the two remaining triangles—removing a proportion of area  from each—creates four triangles, and further repetitions double the number of remaining triangles. By carefully choosing the proportions of areas removed
from each—creates four triangles, and further repetitions double the number of remaining triangles. By carefully choosing the proportions of areas removed  , you can generate a set of points with any desired Lebesgue measure between 0 and 1. The construction is due to Knopp, a refinement of previous attempts by Sierpinski and Osgood.
, you can generate a set of points with any desired Lebesgue measure between 0 and 1. The construction is due to Knopp, a refinement of previous attempts by Sierpinski and Osgood.
Contributed by: Robert Dickau (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
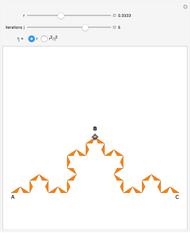
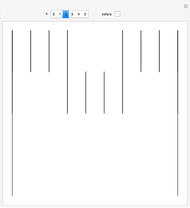
Snapshot 1: when  is the constant
is the constant  , the limit of the iteration is the Koch curve
, the limit of the iteration is the Koch curve
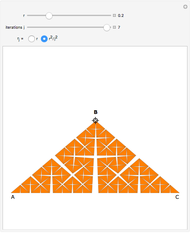
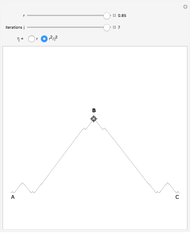
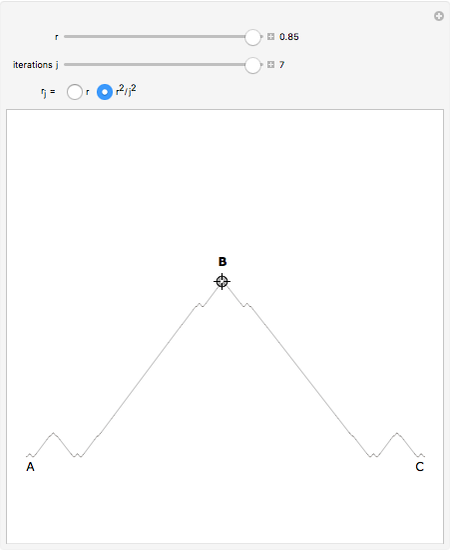
Snapshots 2, 3: when  converges, the Lebesgue measure of the resulting point set is greater than 0; with
converges, the Lebesgue measure of the resulting point set is greater than 0; with  , by selecting a value of
, by selecting a value of  one can achieve any desired Lebesgue measure in
one can achieve any desired Lebesgue measure in 
H. Sagan, Space-Filling Curves, New York: Springer-Verlag, 1994 pp. 136–140.
Permanent Citation