Lyapunov Exponents of Elementary Cellular Automata

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
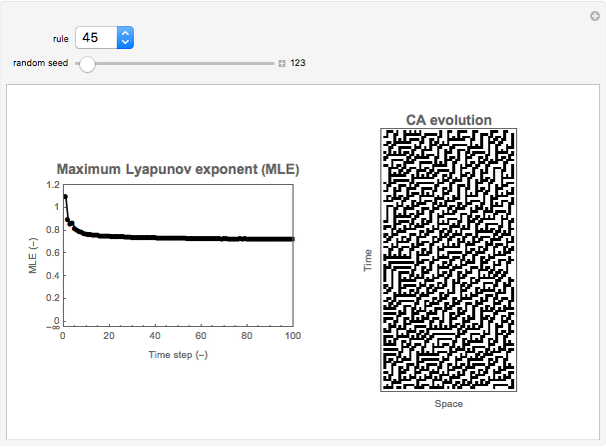
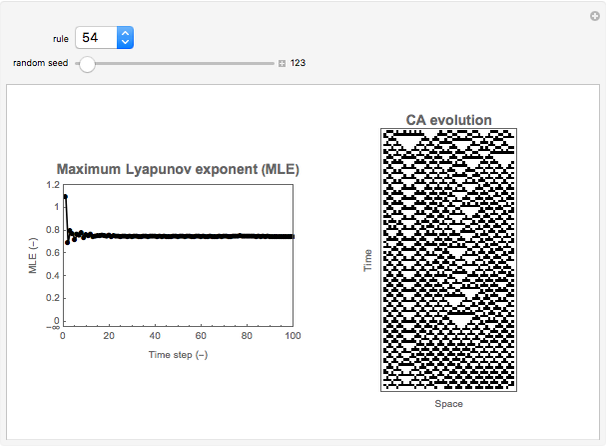
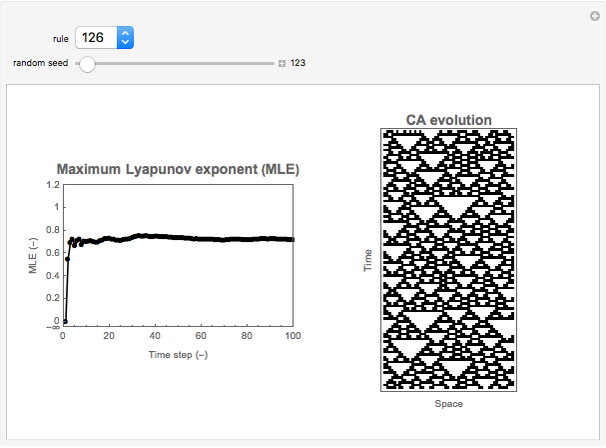
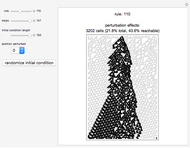
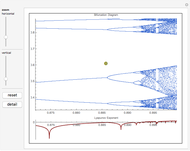
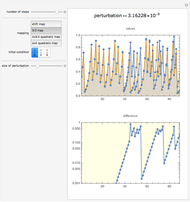
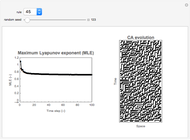
The nondirectional maximum Lyapunov exponent (MLE) of an elementary cellular automaton (CA) may be interpreted as the natural logarithm of the time averaged number of cells  in a cell's neighborhood
in a cell's neighborhood  ) that is affected during each consecutive time step if the state of
) that is affected during each consecutive time step if the state of  is perturbed. Clearly, this number is bounded above by |
is perturbed. Clearly, this number is bounded above by | )|, so that the MLE of an elementary CA can at most attain
)|, so that the MLE of an elementary CA can at most attain  . This indicates strongly diverging phase-space trajectories, whereas a CA that evolves to a stable (homogeneous) configuration after a certain transient gives rise to
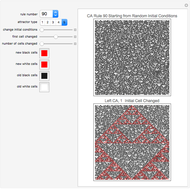
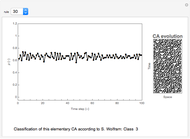
. This indicates strongly diverging phase-space trajectories, whereas a CA that evolves to a stable (homogeneous) configuration after a certain transient gives rise to  . Actually, the MLE can be employed to quantitatively assess the stability of a CA, and may serve as a valuable alternative for a typification that is based upon a visual inspection of the evolved space-time diagrams. Mostly, the limit value of the MLE for a large number of time steps does not change much if the simulation is started from another randomly chosen initial condition, though for some rules it has been found that they can give rise to either
. Actually, the MLE can be employed to quantitatively assess the stability of a CA, and may serve as a valuable alternative for a typification that is based upon a visual inspection of the evolved space-time diagrams. Mostly, the limit value of the MLE for a large number of time steps does not change much if the simulation is started from another randomly chosen initial condition, though for some rules it has been found that they can give rise to either  or
or  , depending on the initial condition on which the CA's evolution is built. Nondirectional Lyapunov exponents can be used to grasp the dynamics of
, depending on the initial condition on which the CA's evolution is built. Nondirectional Lyapunov exponents can be used to grasp the dynamics of  -dimensional CA, graph CA, and so on. This Demonstration lets you evaluate the MLE for the 256 elementary CA based upon a randomly chosen initial condition.
-dimensional CA, graph CA, and so on. This Demonstration lets you evaluate the MLE for the 256 elementary CA based upon a randomly chosen initial condition.
Contributed by: Jan Baetens (April 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
More information on nondirectional Lyapunov exponents and the algorithm to compute them can be found in [1].
References
[1] J. M. Baetens and B. De Baets, "Phenomenological Study of Irregular Cellular Automata Based on Lyapunov Exponents and Jacobians," Chaos, 20, 2010, 033112.doi:10.1063/1.3460362.
[2] J. M. Baetens and B. De Baets, "On the Topological Sensitivity of Cellular Automata," Chaos, preliminary, 2011.
[3] F. Bagnoli, R. Rechtman, and S. Ruffo, "Damage Spreading and Lyapunov Exponents in Cellular Automata," Physics Letters A, 172, 1992, pp. 34–38. doi:10.1016/0375-9601(92)90185-O.
Permanent Citation
"Lyapunov Exponents of Elementary Cellular Automata"
http://demonstrations.wolfram.com/LyapunovExponentsOfElementaryCellularAutomata/
Wolfram Demonstrations Project
Published: April 13 2011