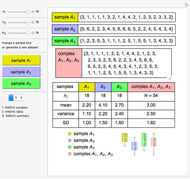
Numerical Example of One-Way ANOVA

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
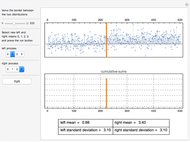
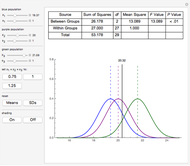
This Demonstration illustrates some basic principles of one-way ANOVA (factor analysis of variance) and shows how it works so you can analyze the statistical variability of a statistical complex.
[more]
Contributed by: Olexandr Eugene Prokopchenko and Pylyp Prokopchenko (September 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
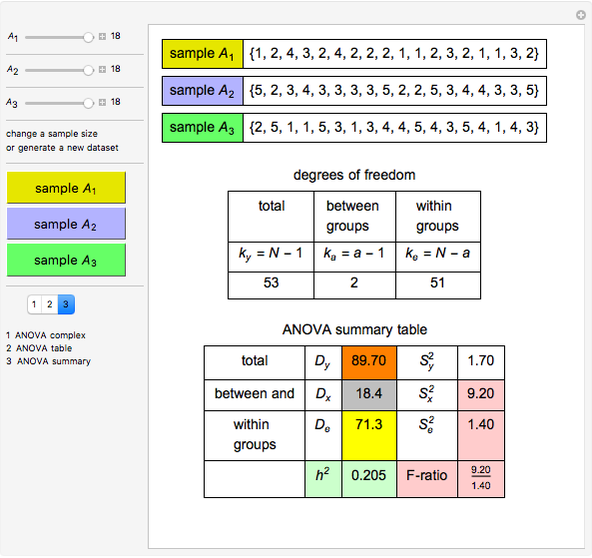
In ANOVA logic, partitioning the sources of variance and hypothesis testing can be done individually. According to the ANOVA method, the total variation is decomposed into two parts: a source of variation due to the group or factor effect (expressed with 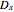 ) and a source of variation due to the measurement error (expressed with
) and a source of variation due to the measurement error (expressed with 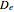 ).
).
This Demonstration uses the ANOVA table algorithm based on deviations.
By definition, the variance 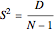 , where
, where 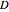 is the sum of squared deviations;
is the sum of squared deviations; 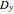 is the total deviation, based on the differences between variants
is the total deviation, based on the differences between variants 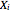 and the mean of statistical complex;
and the mean of statistical complex; 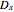 is the intergroup or between-group deviation, based on differences between each group (or sample) and the complex means; and
is the intergroup or between-group deviation, based on differences between each group (or sample) and the complex means; and 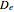 is the intragroup or within-group deviation, based on differences between the variants
is the intragroup or within-group deviation, based on differences between the variants 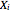 and the sample (group) mean.
and the sample (group) mean.
This Demonstration illustrates some basic principles of one-way ANOVA only. We know that the Fisher 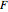 -test is used for comparisons of the components of the total deviation. The
-test is used for comparisons of the components of the total deviation. The 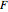 -value is the ratio of variance between and variance within samples (groups).
-value is the ratio of variance between and variance within samples (groups).
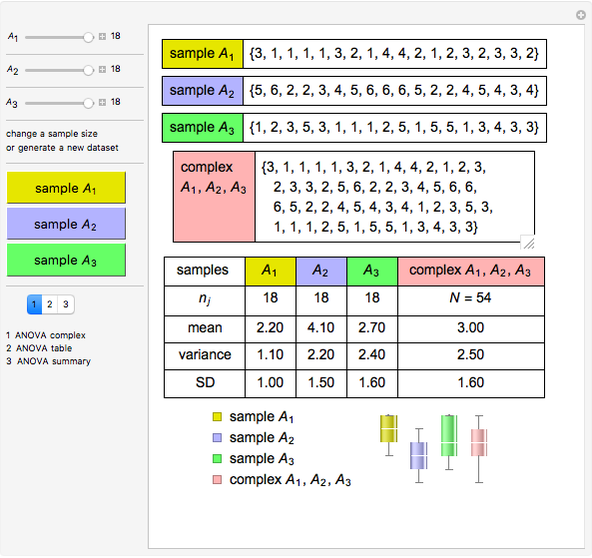
Consider an experiment to study the effect of three different levels of a factor on a response 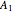 ,
, 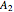 ,
, 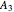 . With
. With 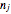 (here we use
(here we use  but say there are
but say there are  groups) observations for each level, we write the outcome of the experiment in a work table.
groups) observations for each level, we write the outcome of the experiment in a work table.
To calculate the 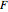 -ratio:
-ratio:
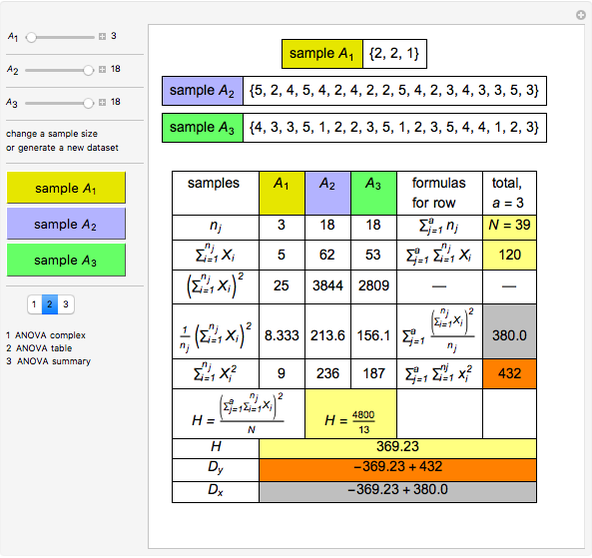
Calculate the size of the statistical complex (first row in the work table) 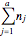 .
.
Calculate the sum 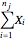 within each sample (group), and the complex total sum
within each sample (group), and the complex total sum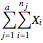 , adding values in the row.
, adding values in the row.
Calculate 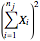 , the square of the sum.
, the square of the sum.
Find the value 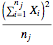 and the total sum in the row:
and the total sum in the row:
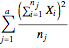 .
.
Calculate the 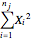 (sum of square).
(sum of square).
Find the total sum in the row 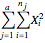 .
.
Find the value 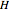 :
:
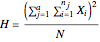
and the deviations:
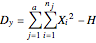 ,
,
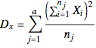 ,
,
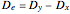 .
.
Calculate total variance 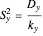 , between-group variance
, between-group variance 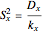 , and within-group variance
, and within-group variance 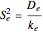 .
.
The statistical ANOVA complex degrees of freedom is  .
.
The between-group degrees of freedom is one less than the number of groups (samples):  .
.
The within-group degrees of freedom is 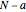 .
.
And finally we find the 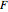 -ratio:
-ratio:
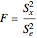
and the relative factor effect:
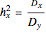 .
.
It is well known that the 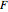 -test is used to compare the components of the total deviation. In this Demonstration, we are using the textbook method of concluding the hypothesis test: compare the observed
-test is used to compare the components of the total deviation. In this Demonstration, we are using the textbook method of concluding the hypothesis test: compare the observed 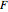 -value with the critical value of
-value with the critical value of 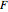 determined from tables. The critical value of
determined from tables. The critical value of 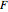 is a function of the numerator degrees of freedom, the denominator degrees of freedom, and the significance level alpha. If the experimental
is a function of the numerator degrees of freedom, the denominator degrees of freedom, and the significance level alpha. If the experimental 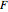 -value is more than critical
-value is more than critical 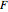 -value, then reject the null hypothesis.
-value, then reject the null hypothesis.
The Demonstration has thus illustrated how we can get and apply the experimental 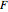 -value.
-value.
References
[1] A. Gelman, "Analysis of Variance—Why It Is More Important Than Ever," Annals of Statistics, 33(1), 2005. pp. 1–53. doi:10.1214/009053604000001048.
[2] D. C. Montgomery, Design and Analysis of Experiments, 5th ed., New York: Wiley, 2001.
Permanent Citation