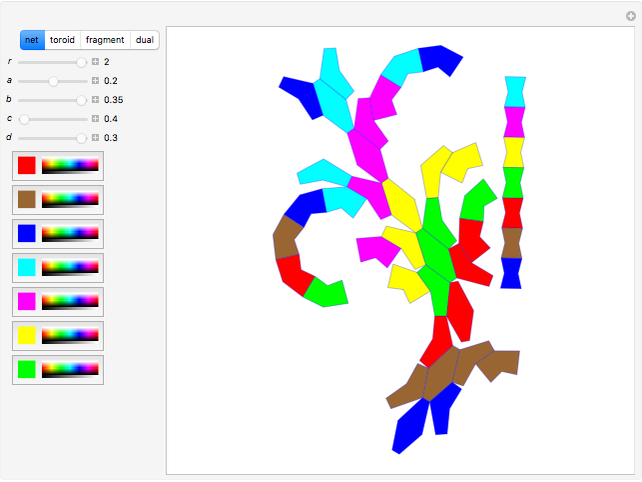
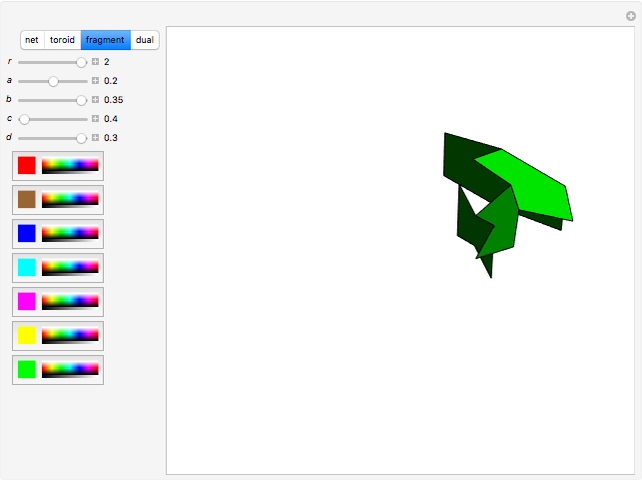
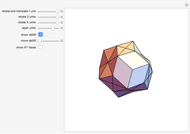
Parametrized Toroid with 42 Faces

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
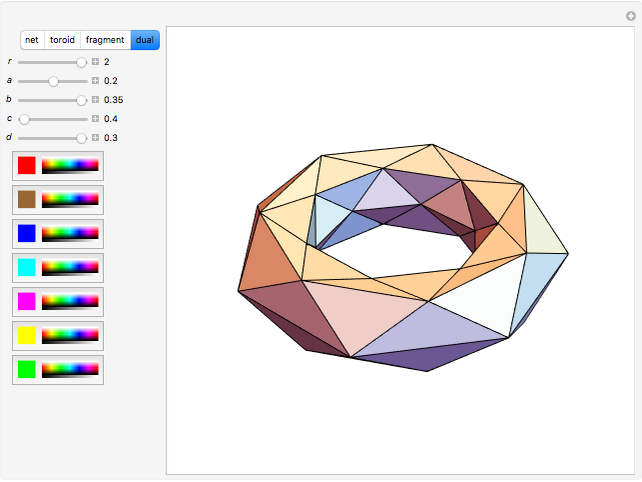
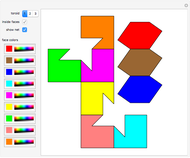
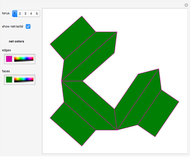
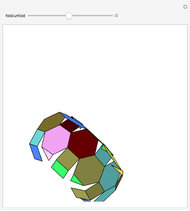
This Demonstration shows a parametrized toroid with 42 faces. Looking down along the axis of symmetry, the larger and smaller concave heptagons have circumscribing circles of radius  and 1, respectively. The vertical faces are double trapezoids with bases of length
and 1, respectively. The vertical faces are double trapezoids with bases of length 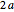 ,
, 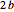 (inner) and
(inner) and 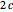 ,
, 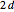 (outer). This polyhedral toroid is colored so that it realizes the Heawood map.
(outer). This polyhedral toroid is colored so that it realizes the Heawood map.
Contributed by: Lajos Szilassi (University of Szeged, Hungary) and Izidor Hafner (May 2016)
Additional contribution by: Sándor Kabai
Open content licensed under CC BY-NC-SA
Snapshots
Details
When constructing this toroid, care must be taken to make sure that the six points defining a face region are in the same plane [2, p. 325]. This toroid belongs to the class 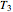 , which means that it is a regular toroid with hexagonal faces, with three edges meeting at each vertex [2, p. 318]. The dual has triangular faces, with six edges meeting at each vertex, thus it belongs to the class
, which means that it is a regular toroid with hexagonal faces, with three edges meeting at each vertex [2, p. 318]. The dual has triangular faces, with six edges meeting at each vertex, thus it belongs to the class 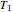 .
.
References
[1] L. Szilassi, "Regular Toroids," Structural Topology, Université de Montréal, 13, 1986 pp. 69–80. www-iri.upc.es/people/ros/StructuralTopology/ST13/st13-06-a3-ocr.pdf.
[2] L. Szilassi, "On Three Classes of Regular Toroids," Symmetry: Culture and Science, 11(1–4), 2000 pp. 317–335.
[3] "Shelf of Lajos Szilassi." www.kabai.hu/elte-mathematical-museum.
[4] B. M. Stewart, Adventures among the Toroids, Okemos, Michigan: B. M. Stewart, rev. 2nd ed., 1980 p. 199.
Permanent Citation