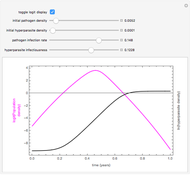
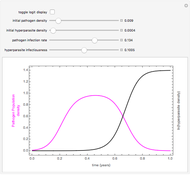
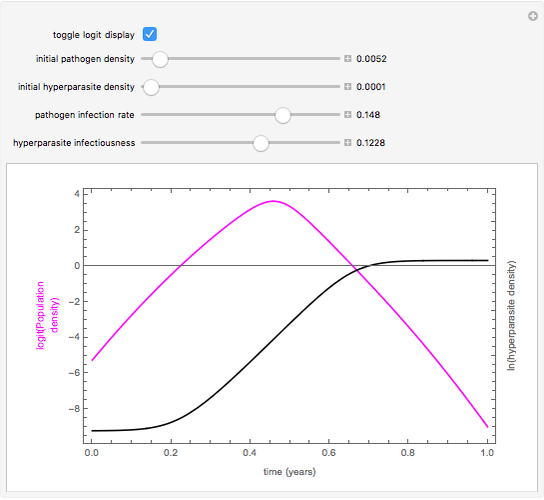
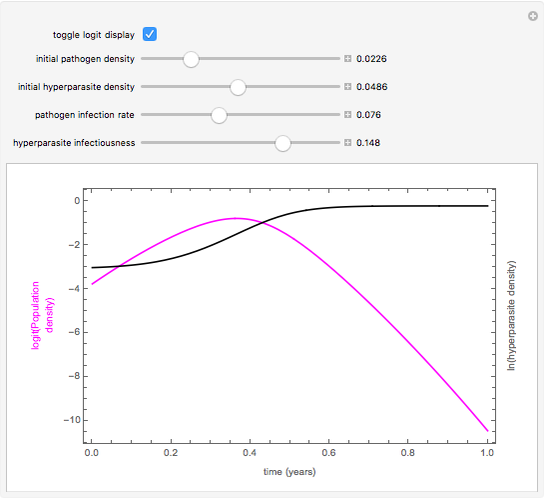
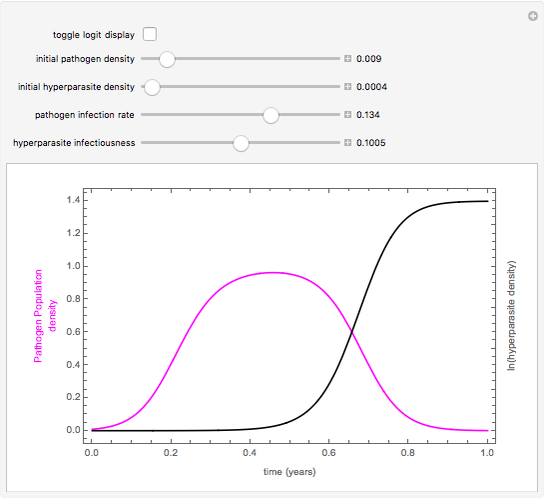
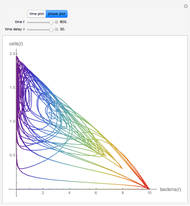
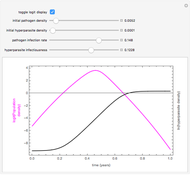
This Demonstration shows the annual cycle of a population of a plant pathogen that is itself attacked by a hyperparasite. The pathogen increases at a per capita rate that is reduced in proportion to the population size and decreases during the season as conditions become progressively unfavorable. It is destroyed by a hyperparasite at a rate that is proportional to both populations. The growing season is assumed to be 180 days. The hyperparasite harms the pathogen and multiplies by destroying it, but has no in-season death rate. This models something with long-lived structures but only one food source.