Probing Macroscopic Quantum States with Gravitational-Wave Detectors

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
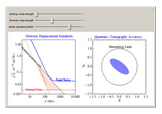
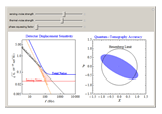
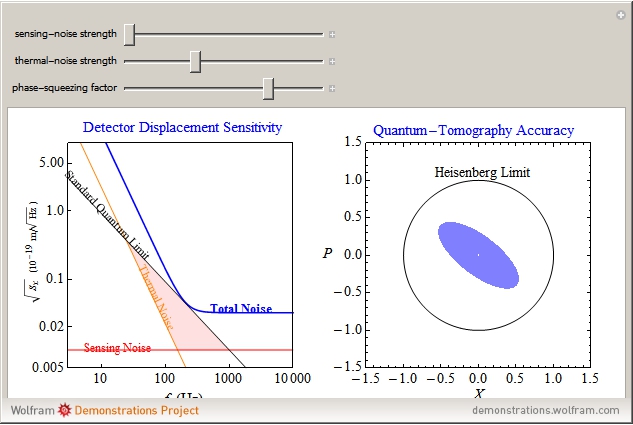
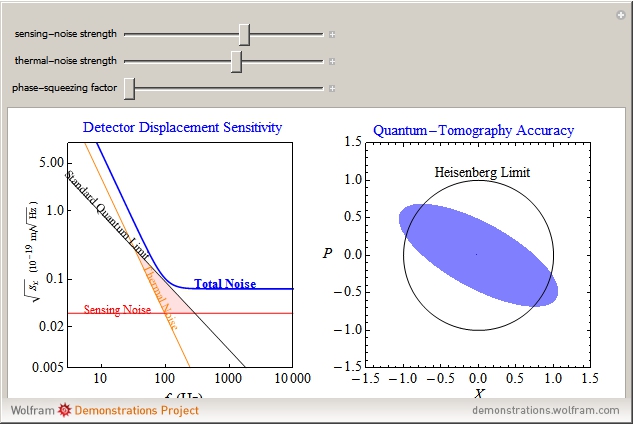
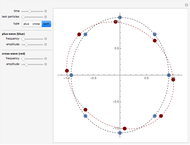
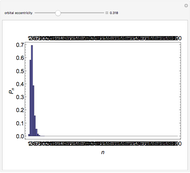
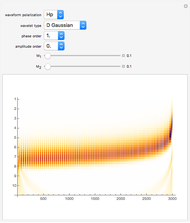
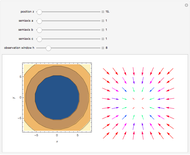
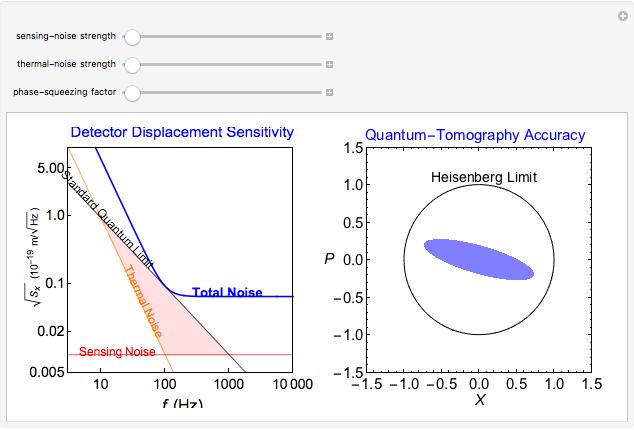
With state-of-the-art technologies, various types of sensors and detectors have achieved such high sensitivities that we will soon be able to probe the quantum jiggling of macroscopic objects. One notable example is the gravitational-wave (GW) detectors that sense the extremely tiny motion of the kilogram-scale test masses caused by gravitational waves. At present, a global network of GW detectors is now operating at their design sensitivities. In addition, tremendous efforts have been made in the GW community to further increase detector sensitivity. For the planned advanced detectors, the anticipated noise levels should be below the standard quantum limit. This will not only allow us to detect GWs from distant astronomical sources with high fidelity but also to shed light on investigations of quantum behavior of really macroscopic objects (~ 40 kg test masses). In previous work (see reference [1] below), we showed that the classical noise budget of advanced GW detectors allows for the creation of a nearly Heisenberg-limited quantum state of the test mass. More recently (see reference [2]), we showed that by applying an optimal measurement strategy, the same detector can also be used to probe this quantum state with accuracy below the Heisenberg limit.
[more]
Contributed by: Haixing Miao, Stefan Danilishin, Helge Muller-Ebhardt, Henning Rehbein, Kentaro Somiya, and Yanbei Chen (July 2009)
Open content licensed under CC BY-NC-SA
Snapshots
Details
For more technical details, please refer to
[1] H. Mueller-Ebhardt, H. Rehbein, C. Li, Y. Mino, K. Somiya, R. Schnabel, K. Danzmann, and Y. Chen, "Quantum State Preparation and Macroscopic Entanglement in Gravitational-Wave Detectors," arXiv:0903.0079, 2009.
[2] H. Miao, S. Danilishin, H. Muller-Ebhardt, H. Rehbein, K. Somiya, and Y. Chen, "Probing Macroscopic Quantum States with a Sub-Heisenberg Accuracy," arXiv:0905.3729, 2009.