Real Roots of Sparse Polynomials

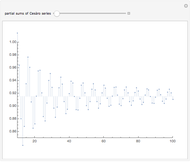
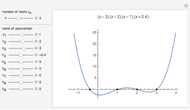
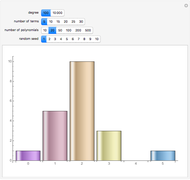
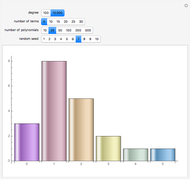
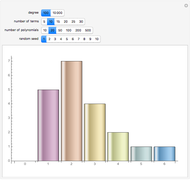
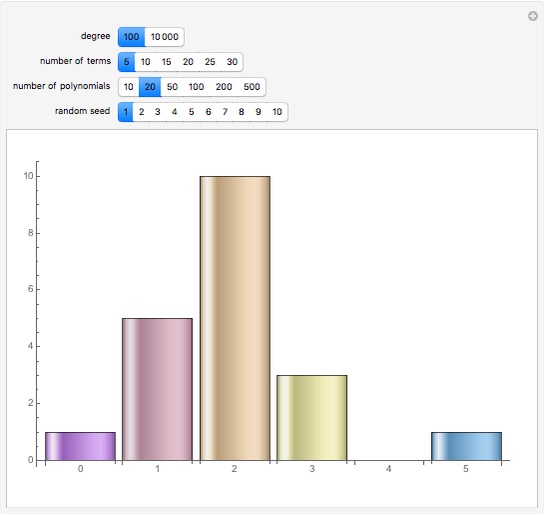
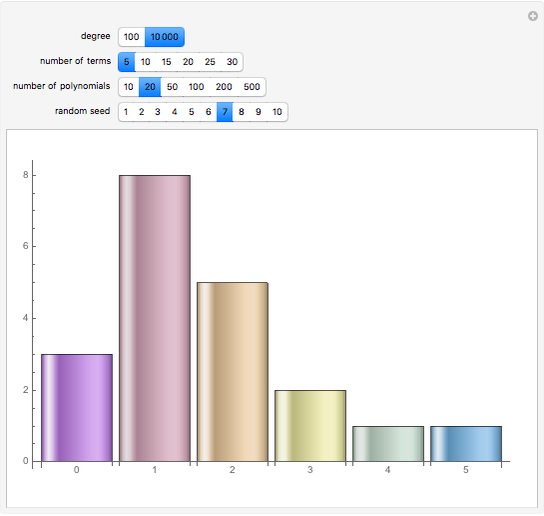
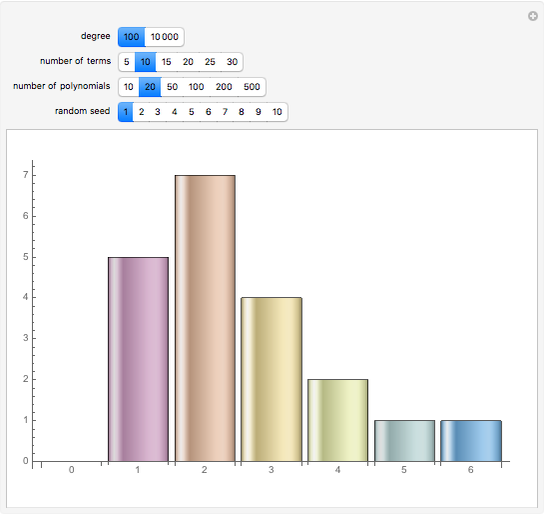
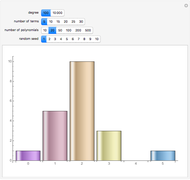
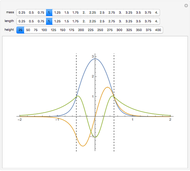
The number of real roots of a polynomial with terms is bounded by and does not depend on the degree of the polynomial. This chart shows the distribution of the number of real roots for 20 randomly generated polynomials of degree 100 with 10 terms each. If you keep the number of terms fixed and increase the degree to 10,000, the distribution of the number of real roots will be similar. With Mathematica 7 you can find real roots of polynomials with much higher degrees; however for polynomials of degree 1,000,000 generating the root distribution charts would take from a few seconds to about a minute per polynomial.