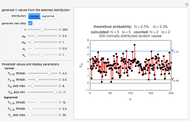
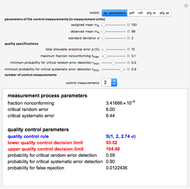
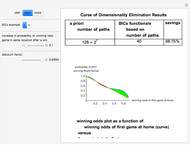
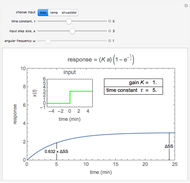
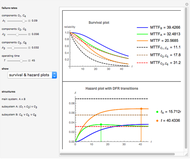
The main goal of structural reliability analysis is to compute the probability of failure of an event, which may be described as an individual component or a larger system. In the former case, it is usual to define the component in terms of a limit-state function, LSF. LSFs are functions of random variables and separate the failure domain from the safe domain. Thus, the probability of failure results from the integration of the joint multivariate probability density function (PDF) over the failure domain. Defining the LSF and the multivariate PDF and performing the integrations are challenging tasks.
Under the first-order reliability method (FORM) scheme, the joint multivariate PDF is transformed into a joint standard multinormal uncorrelated PDF. The LSF is also transformed and approximated by a hyperplane crossing the closest point from the LSF to the system origin lying at the mean values (all are zero). This point is known as the most probable point (MPP) or the design point. The distance between the MPP and the origin is called the reliability index,  . Thus, the probability of failure approximates to the multifold integral of a standard multinormal uncorrelated PDF beyond a hyperplane located at the distance
. Thus, the probability of failure approximates to the multifold integral of a standard multinormal uncorrelated PDF beyond a hyperplane located at the distance  from the origin.
from the origin.
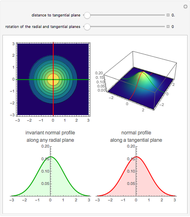
Under these conditions, the multifold integral always reduces to an integral of a standard normal variate. The FORM probability of failure is computed using the cumulative probability distribution of a standard normal with negative argument,  . The formulation takes advantage of the rotational symmetry property found in a multinormal uncorrelated PDF.
. The formulation takes advantage of the rotational symmetry property found in a multinormal uncorrelated PDF.
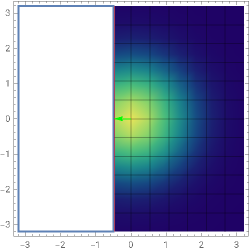
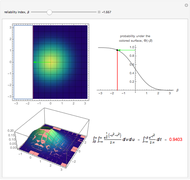
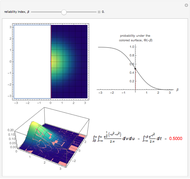
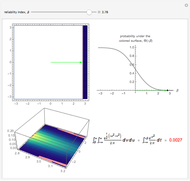
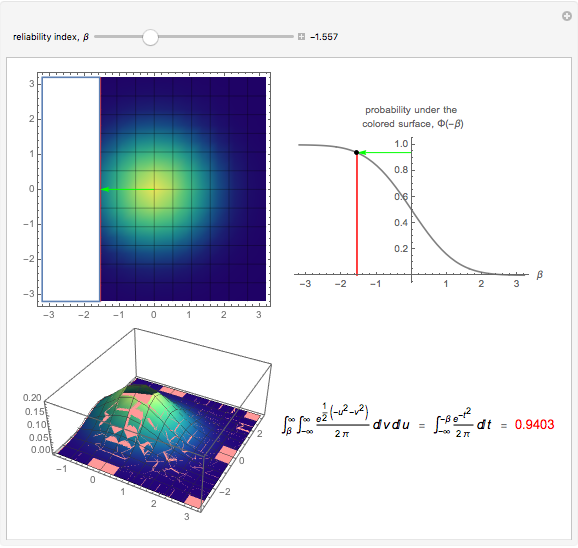
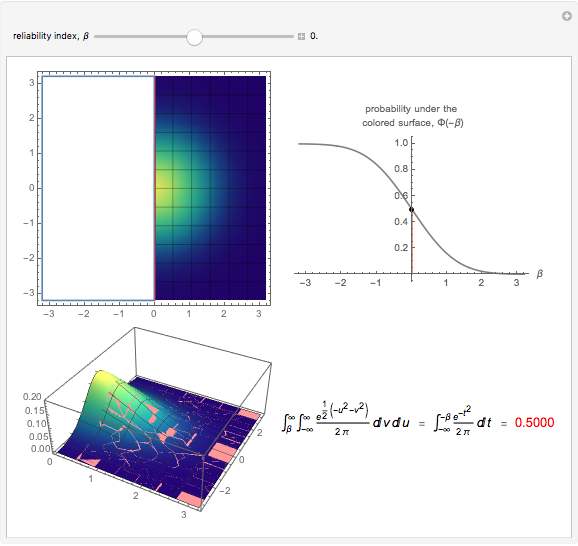
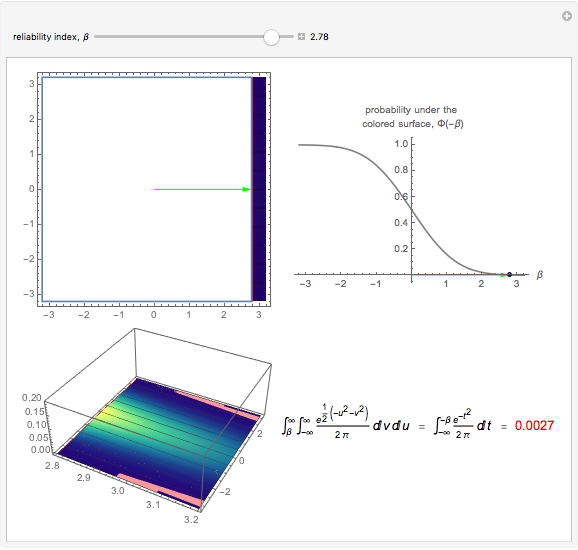
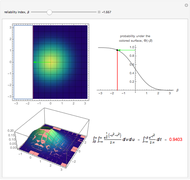
For the bivariate case, this Demonstration depicts the region of a density plot for a bivariate standard normal uncorrelated beyond the approximated LSF at a distance  , shown as a green arrow. The Demonstration also renders a 3D plot with similar features and plots
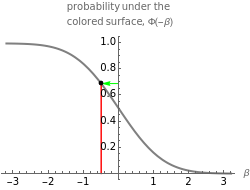
, shown as a green arrow. The Demonstration also renders a 3D plot with similar features and plots  versus
versus  with
with  again as a green arrow and the numerical value of the probability as a red ordinate. Finally, the lower right shows the two-fold integral for the bivariate function, the single integral, and the numerical value of either integral. Note the limits of the two integrals.
again as a green arrow and the numerical value of the probability as a red ordinate. Finally, the lower right shows the two-fold integral for the bivariate function, the single integral, and the numerical value of either integral. Note the limits of the two integrals.
[less]