Details

A significant number of topological spaces are (or can be modeled by) simplicial complexes—spaces that are "glued" out of simplexes, attaching them face to face. Given a subcomplex of a complex , one would often like to consider a neighborhood of in that approximates fairly well. It is not always possible to build such a out of simplexes of ; For example, if , the only simplicial neighborhood of is the whole segment , which fails to capture the disconnectedness of .

However, there are such neighborhoods that are simplicial in a double barycentric subdivision of (i.e. each simplex is subdivided barycentrically twice). Given any subcomplex of , such a neighborhood is given by in , where is defined as the union of all open simplexes with a vertex in .

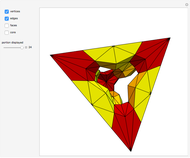
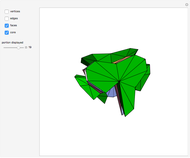
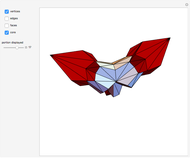
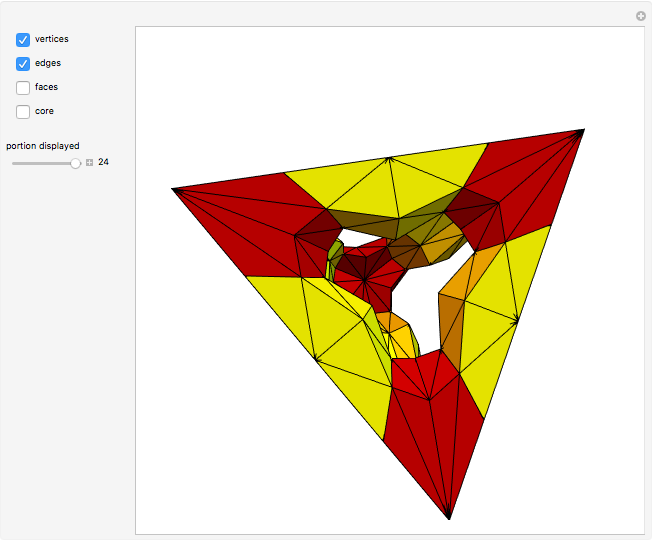
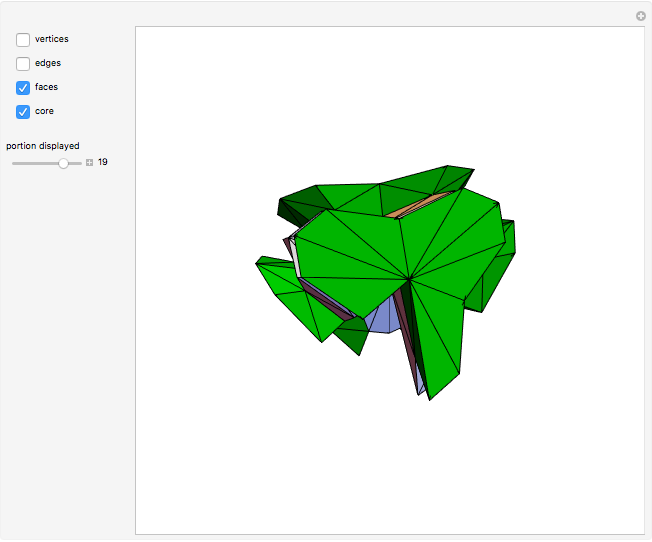
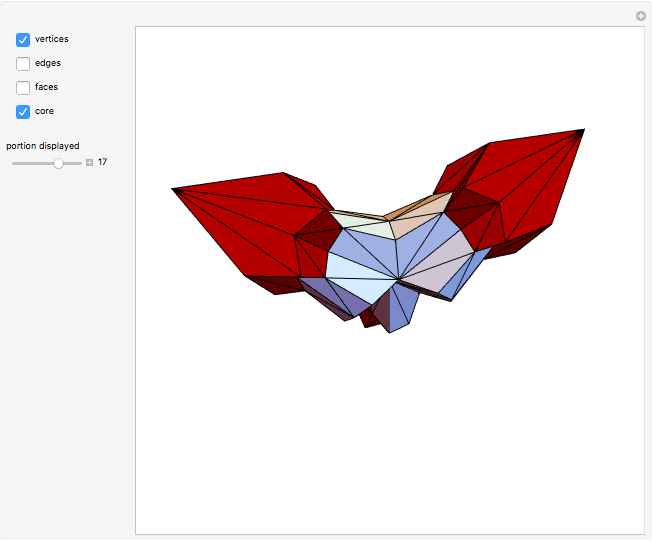
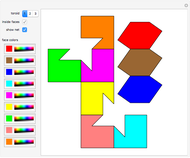
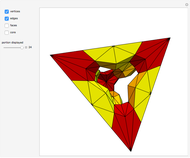
The Demonstration illustrates this concept with the 3-simplex as an example of . Each controller toggles the display of stars (in the double subdivision) of centers of corresponding elements. For example, selecting "vertices" and "edges" gives a neighborhood of a 1-skeleton of (i.e. of the collection of its 1-simplexes).

Barycentric subdivision is defined inductively. Subdivision of a point is the point itself. To define a subdivision of a simplex , take the subdivision of its boundary and take as the collection of simplexes with base the simplex in and vertex the barycenter of .