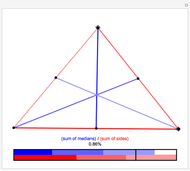
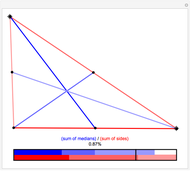
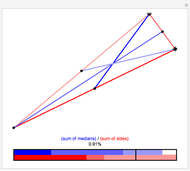
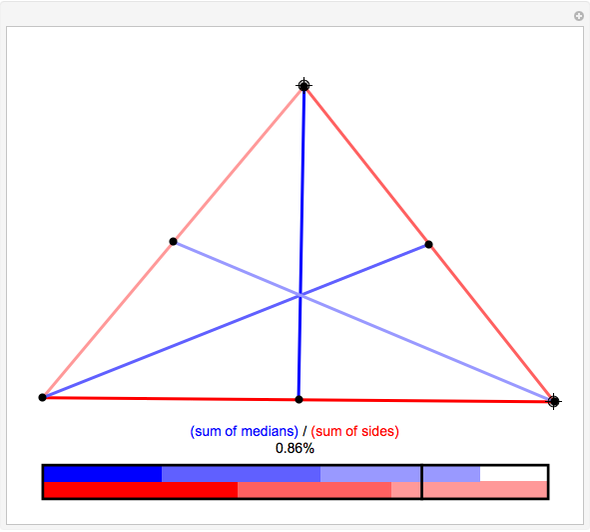
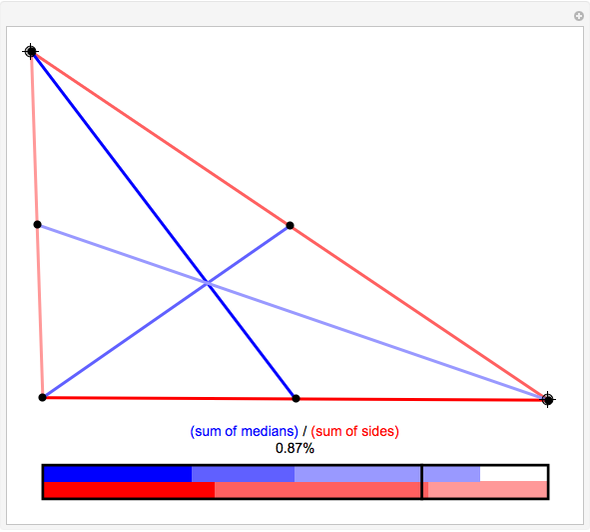
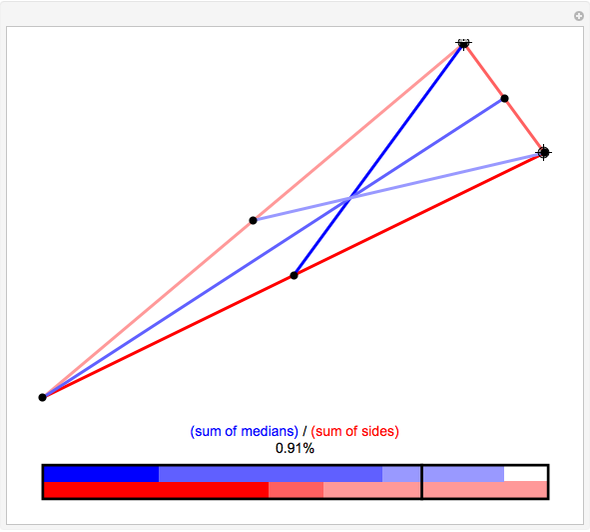
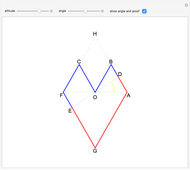
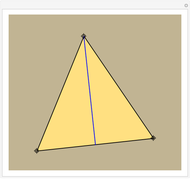
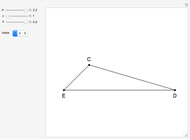
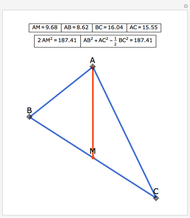
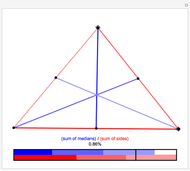
It is a simple theorem that the sum of the lengths of the medians in a triangle must be between and , where is the perimeter of the triangle. By dragging the vertices of the triangle, you can confirm the theorem and discover which kinds of triangles come close to achieving the lower and upper bounds of 75% and 100% of the perimeter, respectively.