Synthesis of Rugate Optical Filter

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
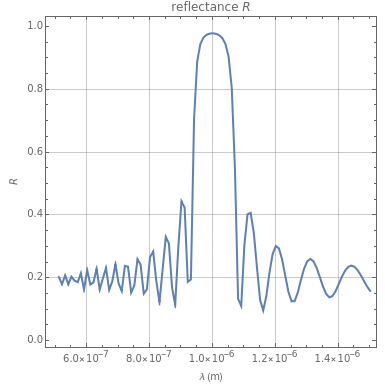
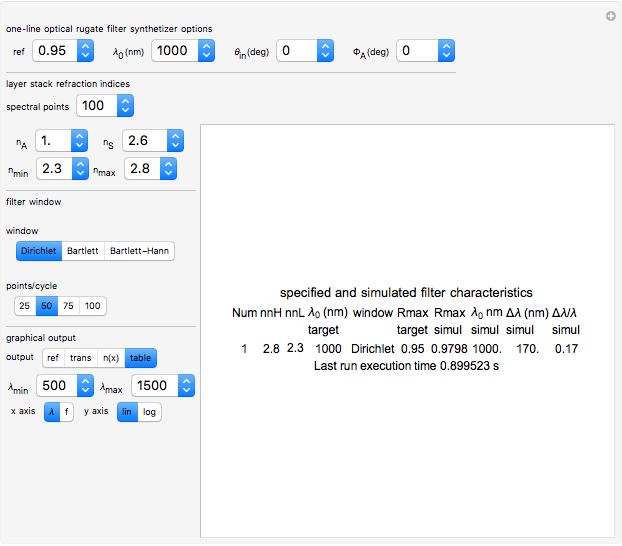
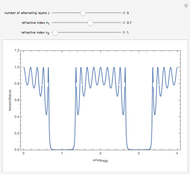
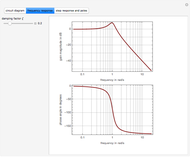
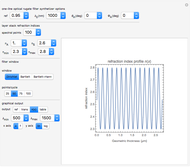
A rugate filter exploits dielectric interference produced by a continuous variation of the refractive index. This Demonstration simulates a one-line rugate optical filter. You can select the central wavelength, maximum and minimum refraction indices, and the desired filter reflectance. The program calculates the corresponding reflectance profile 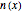 and filter geometrical length. A material with these properties could be fabricated from crystalline silicon by anodic etching.
and filter geometrical length. A material with these properties could be fabricated from crystalline silicon by anodic etching.
Contributed by: Rodolfo Quintero (March 2015)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Single-line filter characteristics
The peak filter response 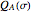 is approximated by
is approximated by
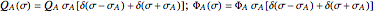 , where
, where 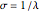 .
.
The following Fourier transform pair determines 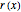 :
:
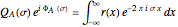 ,
,
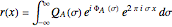 ,
,

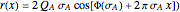 ,
,
and with
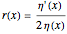 .
.
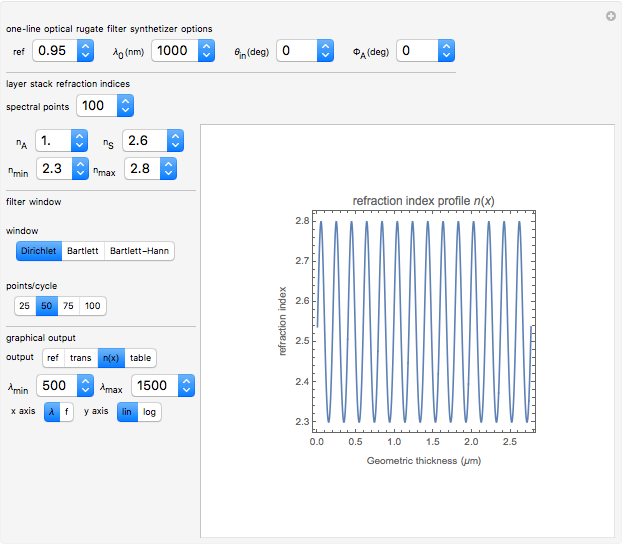
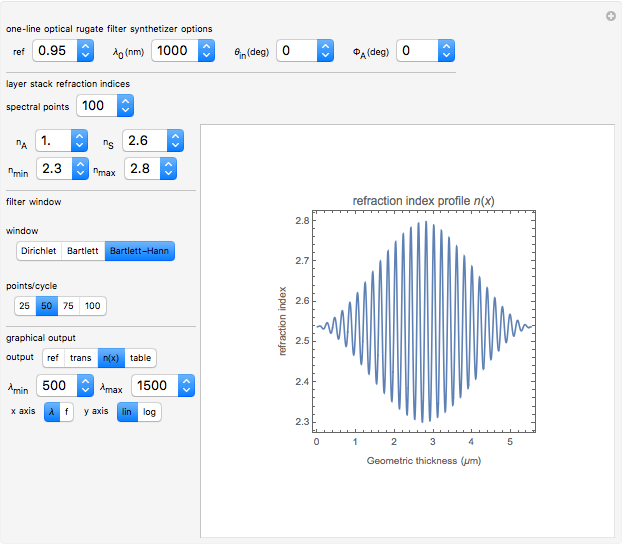
Filter truncation by means of a Dirichlet, Bartlett, or Bartlett–Hann window
The infinite 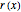 is truncated to a finite length, and the frequency response is calculated by convoluting it with a window, shown here with a simple Dirichlet window:
is truncated to a finite length, and the frequency response is calculated by convoluting it with a window, shown here with a simple Dirichlet window:
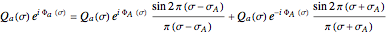 .
.
The filter length 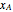 is calculated numerically from the equation
is calculated numerically from the equation
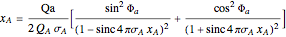 ,
,
where 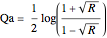 and
and 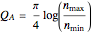 .
.
Here 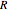 is the reflectance and
is the reflectance and 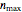 and
and 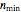 are the minimum and maximum refraction index values. Similar nonlinear equations are solved numerically for the Bartlett and the Bartlett–Hann windows.
are the minimum and maximum refraction index values. Similar nonlinear equations are solved numerically for the Bartlett and the Bartlett–Hann windows.
The optical admittance 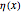 for a Dirichlet window
for a Dirichlet window 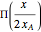 is given by
is given by
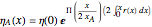 ,
, 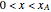 .
.
Two more truncated 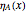 were obtained by substituting for
were obtained by substituting for 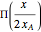 with a Bartlett or a Bartlett–Hann window.
with a Bartlett or a Bartlett–Hann window.
Numerical calculation of the filter response by means of the matrix method, for a transverse electrical field (TE) or s wave [1]
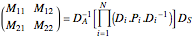 ,
,
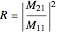 ,
,
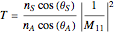 .
.
Here 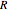 and
and 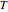 are the reflectance and transmittance of the dielectric stack.
are the reflectance and transmittance of the dielectric stack.
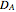 ,
, 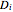 , and
, and 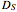 are the dynamical matrices of air, layer
are the dynamical matrices of air, layer 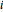 (
(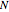 of them), and the substrate, respectively. They deal with boundary conditions between dielectric layers.
of them), and the substrate, respectively. They deal with boundary conditions between dielectric layers. 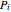 calculates the phase change introduced by layer
calculates the phase change introduced by layer 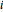 .
.
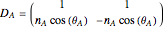 ,
,
 ,
,
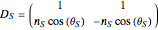 ,
,
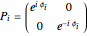 ,
,
where 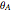 ,
, 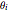 , and
, and 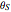 are the incidence angles of the impinging radiation of the air, of layer
are the incidence angles of the impinging radiation of the air, of layer 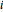 , and of the substrate, respectively;
, and of the substrate, respectively; 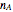 ,
, 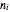 , and
, and 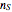 are the dielectric constants of the air, layer
are the dielectric constants of the air, layer 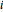 , and the substrate.
, and the substrate. 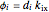 is the phase shift introduced by layer
is the phase shift introduced by layer 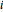 , whose thickness is
, whose thickness is 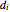 , and
, and 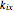 is the wave vector component in the direction of propagation
is the wave vector component in the direction of propagation  .
.
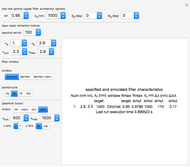
One-line optical filter synthesizer options
ref: Desired maximum reflection 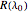
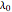 : Central wavelength
: Central wavelength 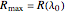
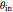 : Incidence angle, 0 deg when impinging radiation is perpendicular to the surface
: Incidence angle, 0 deg when impinging radiation is perpendicular to the surface
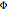 : An adjustment angle, usually 0 deg
: An adjustment angle, usually 0 deg
Layer stack refraction indices
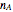 : Refraction index of input medium, normally air
: Refraction index of input medium, normally air
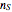 : Refraction index of substrate, for example, silicon
: Refraction index of substrate, for example, silicon
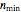 : Minimum refraction index
: Minimum refraction index 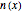 . If made from silicon, it depends on the etching process.
. If made from silicon, it depends on the etching process.
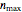 : Maximum refraction index of
: Maximum refraction index of 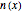 . If made from silicon, it depends on the etching process.
. If made from silicon, it depends on the etching process.
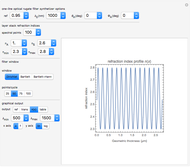
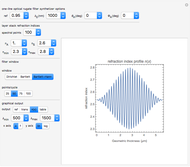
Filter window
Dirichlet: The originally infinite sinusoidal 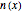 is cut to a finite length.
is cut to a finite length.
Bartlett: The sinusoid is smoothly modulated and vanished; it has reduced sidelobes.
Bartlett–Hann: The sinusoid is smoothly modulated and vanished; it has reduced sidelobes.
Graphical output
ref: Reflectance 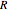
trans: Transmission 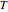
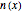 : Refraction index profile
: Refraction index profile
table: Design parameters and simulated results
References
[1] P. Yeh, Optical Waves in Layered Media, New York: John Wiley & Sons, 1988.
[2] L. Sossi, "On the Theory of the Synthesis of Multilayer Dielectric Light Filters," Eesti NSV Teaduste Akadeemia Toimetised Fuusika Matemaatika, 25, 1976 pp. 171–176.
[3] L. Sossi, "A Method for the Design of Multilayer Dielectric Interference Coatings," Eesti NSV Teaduste Akadeemia Toimetised Fuusika Matemaatika, 23, 1974 pp. 229-237.
[4] B. G. Bovard, "Rugate Filter Theory: An Overview," Applied Optics, 32(28), 1993 pp. 5427–5442. doi:10.1364/AO.32.005427.
[5] A. Kovacs, P. Jonnalagadda, and U. Mescheder, "Optoelectrical Detection System Using Porous Silicon-Based Optical Multilayers," IEEE Sensors Journal, 11(10), 2011. doi:10.1109/JSEN.2011.2119310.
Permanent Citation