The 29 Tetrachords and Other Set Classes

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
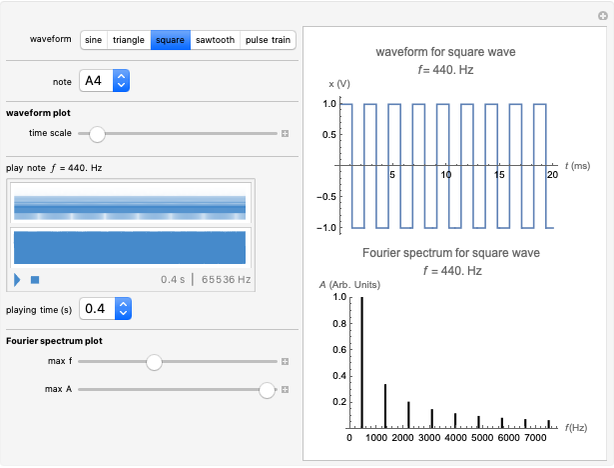
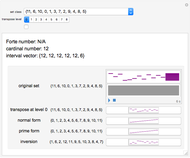
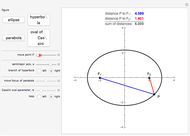
In the study of 12-tone atonal music, a "set class" is a collection of subsets of the 12 notes (or more formally, pitch-classes) of the chromatic scale. This Demonstration uses a common specific definition of a set class as an equivalence class under the relations of both (musical) transposition and inversion. A well-known fact is that there are exactly 29 tetrachords—set classes consisting of four tones. Mathematically, a set class can be viewed as a 12-bead necklace with beads of exactly two colors. This Demonstration allows you to visualize and listen to all set classes consisting of 3, 4, 5, or 6 notes. You can also find and listen to the inverted set class for each of these classes.
Contributed by: Marc Brodie (Wheeling Jesuit University) (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
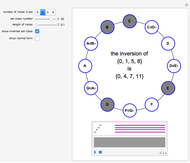
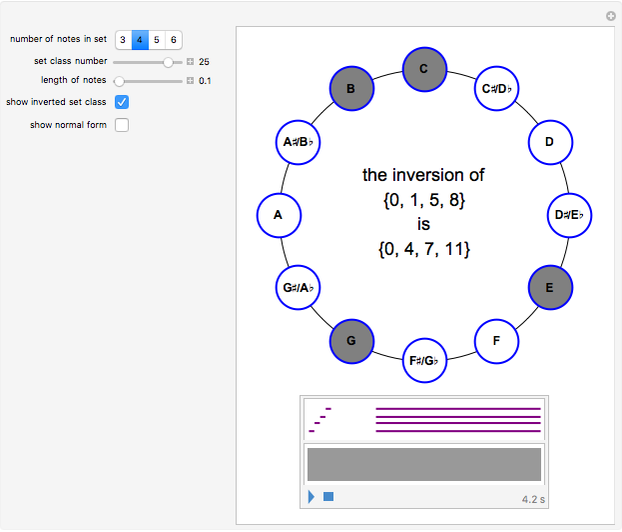
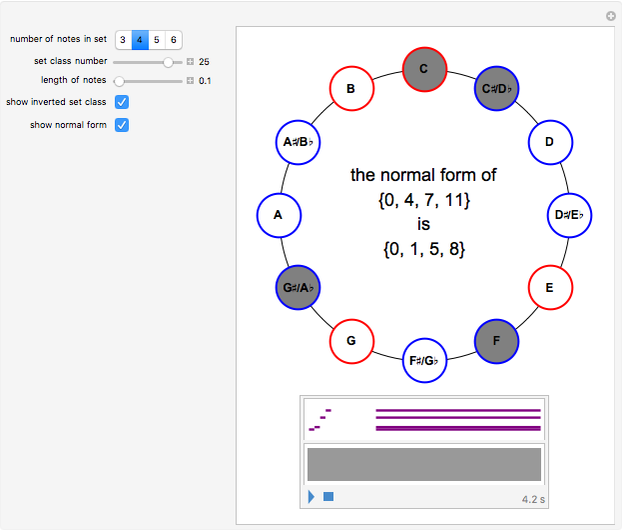
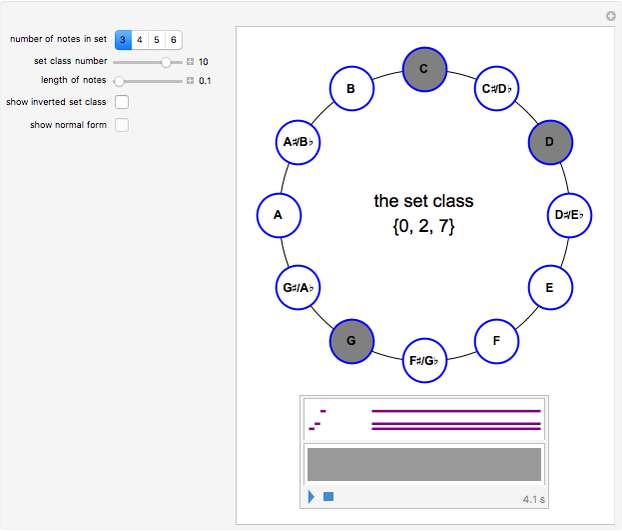
Since transpositions do not alter set classes, a set class is typically given as a subset of {0, 1, 2, …, 11}, where each number represents the note that many half-steps above a fixed note labeled 0. This Demonstration labels Middle C with 0.
The inversion of a set class is obtained by starting at 0 and going down the same number of half-steps that the original set class went up. Thus the inversion of {0, 1, 5, 8} is {0, -1, -5, -8}. Since any two notes that differ by an octave are in the same pitch-class, we are working mod 12. Therefore the inversion of {0, 1, 5, 8} is {0, 11, 7, 4}, which is displayed in the standard increasing order {0, 4, 7, 11}. The inversion can be visualized as the reflection of the original set class over the vertical axis through C and F♯/G♭.
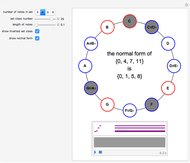
The normal form of a set class is obtained by choosing as 0 the note (pitch-class) in the set that results in the smallest distance from 0 to the highest numbered pitch-class in the set. In other words, the largest ascending interval from 0 in the set class is as small as possible. In the event of a tie, the normal form is the set class that comes first in the usual ordering of sets. Visually, to find the normal form of a set class, the necklace is rotated until with one note at C, the highest note is closest to C in the clockwise direction. The given set classes are in normal form, so normal form is only relevant here when exploring the inverted pitch classes. In this case, a "shadow" of the original inverted set class is displayed by red edge color while the normal form is shown in the usual way.
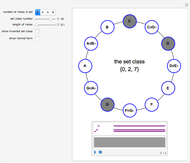
Note that the set class {0, 1, 5, 8} is the same when inverted (snapshots 1–3), but set class {0, 3, 7} is not (snapshot 4). It is interesting to observe that set class {0, 3, 7} is a minor triad and its inversion {0, 4, 7} is a major triad. Thus in this approach to studying atonal music, major and minor triads are "the same".
Permanent Citation