The Ballot Problem
Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
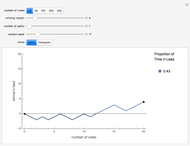
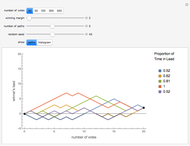
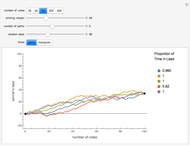
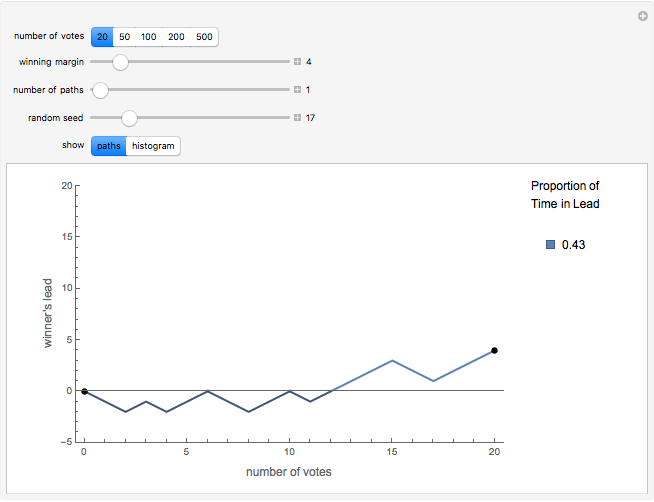
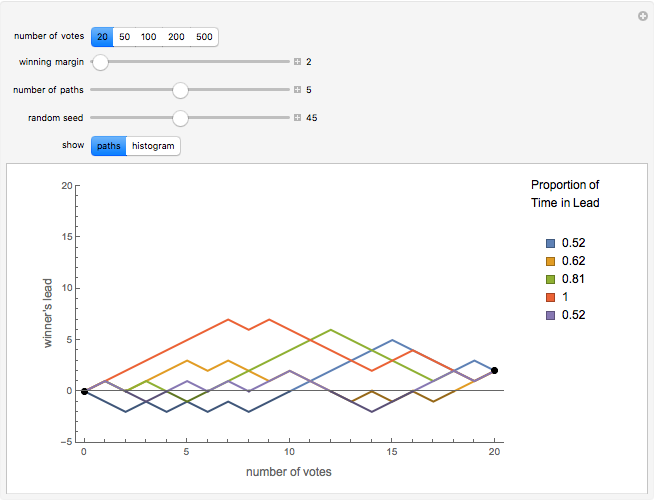
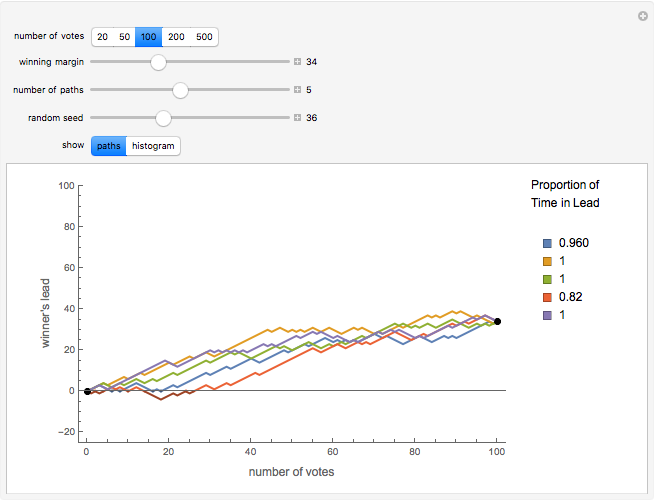
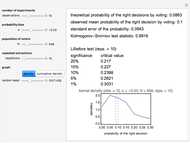
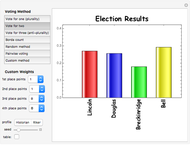
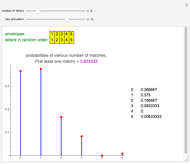
Consider an election with two candidates A and B resulting in 
votes for A and 
votes for B. If candidate A wins the election, the ballot problem is concerned with the probability that A was in the lead throughout the entire voting process. The ballot-counting process may be interpreted as a simple one-dimensional random walk with a step of 
for each vote for A and 
for each vote for B. The ballot problem asks for the probability that a simple random walk from 
to 
is strictly positive except at the origin. This probability turns out to be 
.
Contributed by: Jose Sanchez and Madina Bolat (September 2016)
(Illinois Mathematics Summer REU Program 2016)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation
Three-Candidate Elections Using Saari Triangles
Matthew Romney, Yukun Tan, and Mengzhou Tang
Comparing Voting Systems for a Normal Distribution of Voters
Adam Rumpf
Kernel Density Estimations: Condorcet's Jury Theorem, Part 5
Tetsuya Saito
The 1860 US Presidential Election under Different Voting Methods
Zelin Li and A. J. Hildebrand
Click a Country
Michael Schreiber
Montmort's Problem
Heikki Ruskeepää
Urn Problem
Ed Pegg Jr
The Birthday Problem
Chris Boucher
The Secretary Problem
Heikki Ruskeepää
Buffon's Needle Problem
Ed Pegg Jr and Eric W. Weisstein
