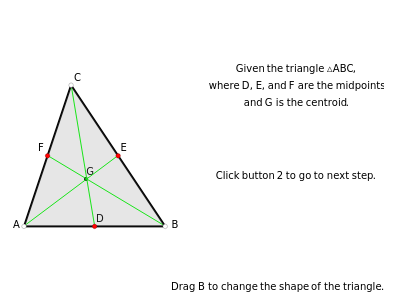
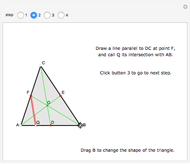
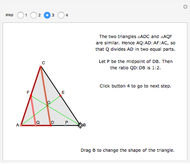
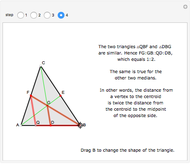
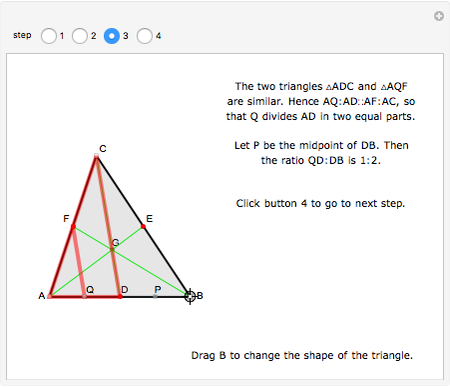
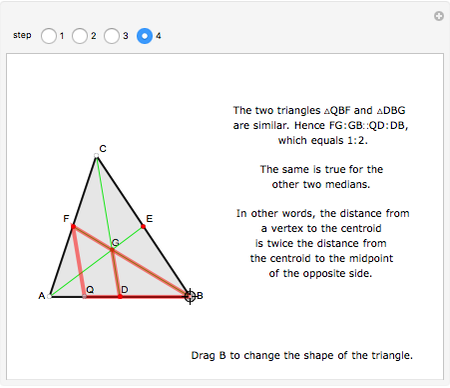
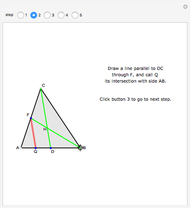
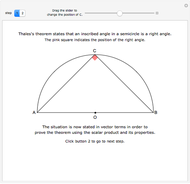
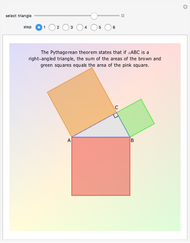
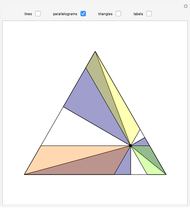
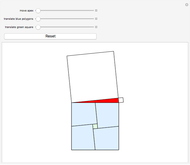
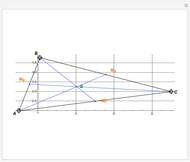
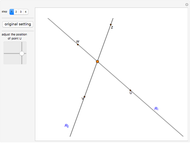
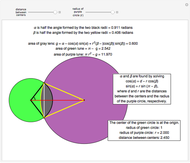
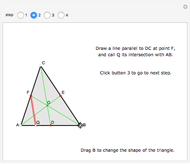
A median is the line from a midpoint of a side to the opposite vertex. The medians meet in the centroid, which is the center of mass of the triangle. A visual proof is given for the fact that the centroid of a triangle splits each of the medians in two segments, the one closer to the vertex being twice as long as the other one.