The Harmonic Oscillator in Extended Relativistic Dynamics

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
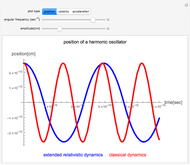
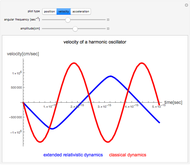
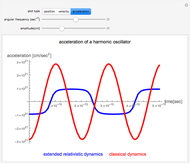
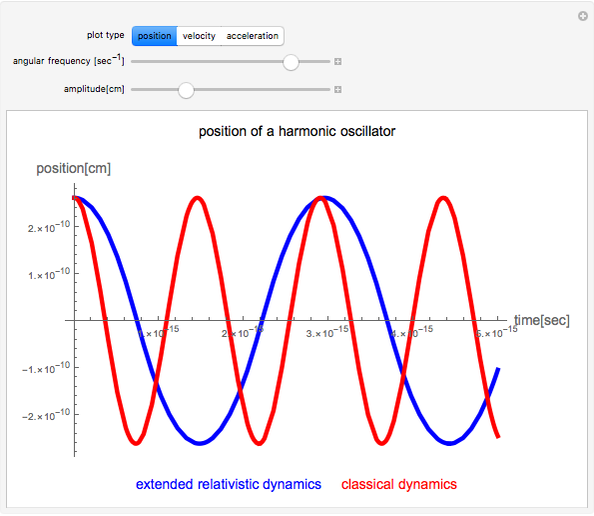
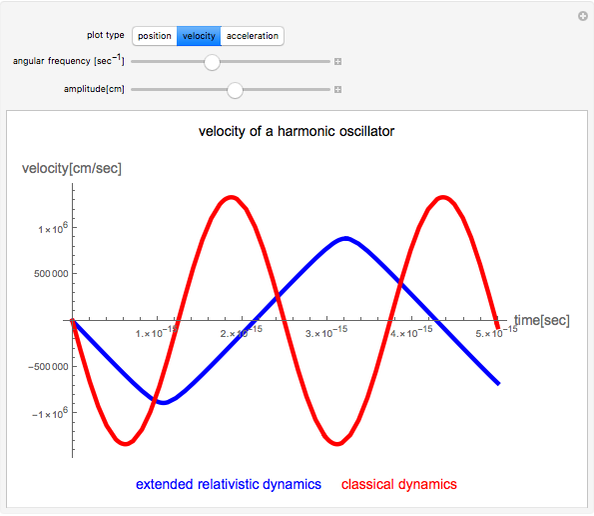
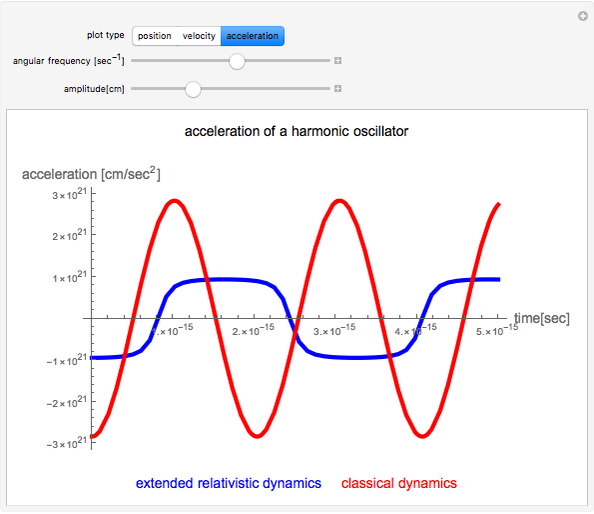
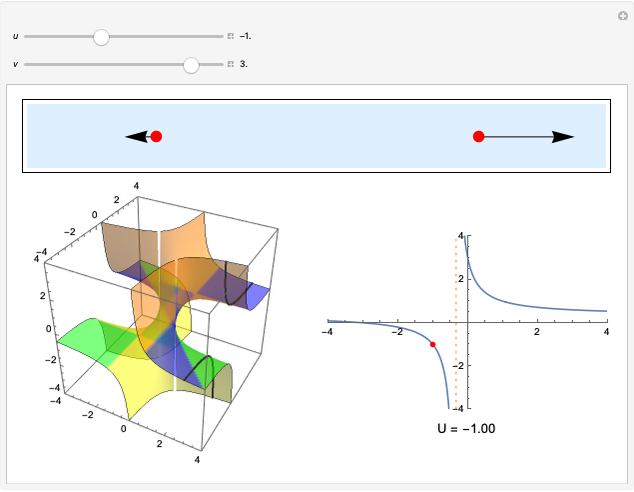
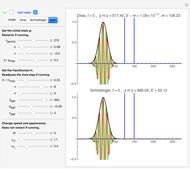
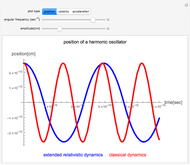
Extended relativistic dynamics is a speculative modification of special relativity that proposes a maximum possible value of the acceleration. This Demonstration shows the velocity and acceleration of the extended relativistic harmonic oscillator compared to the classical harmonic oscillator as functions of the natural frequency  and amplitude
and amplitude 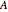 .
.
Contributed by: Esra Yudkin (July 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
This Demonstration shows how the motion of a harmonic oscillator behaves according to "extended relativity" [1, 2], as compared to the classical model. In extended relativity, it is assumed that both the velocity and acceleration are bounded: the velocity by  , and the acceleration by
, and the acceleration by 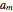 , a value which, by some indications, approximates
, a value which, by some indications, approximates 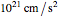 .
.
For the classical model of the harmonic oscillator, the Hamiltonian is
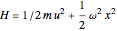 .
.
For the extended relativistic model of the harmonic oscillator, the Hamiltonian is generalized to [1, 2, 3]
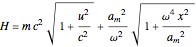 .
.
By solving the Hamiltonian system
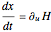 ,
,
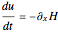 ,
,
we find the motion of the harmonic oscillator.
In the classical model, the effective frequency (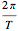 , where
, where 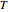 is the period of the motion) and the natural frequency are equal. In extended relativity, however, the effective frequency is considerably smaller. It can be shown [2, 3] that as the natural frequency goes to infinity, the effective frequency approaches
is the period of the motion) and the natural frequency are equal. In extended relativity, however, the effective frequency is considerably smaller. It can be shown [2, 3] that as the natural frequency goes to infinity, the effective frequency approaches
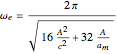 .
.
References
[1] Y. Friedman, "The Maximal Acceleration, Extended Relativistic Dynamics and Doppler Type Shift for an Accelerated Source," Annalen der Physik (Berlin), 523(5), 2011 pp. 408–416. arxiv.org/abs/0910.5629.
[2] Y. Friedman, "Extending the Relativity of Time," Proceedings of IARD 2012, 8th Biennial Conference on Classical and Quantum Relativistic Dynamics of Particles and Fields, Florence, Italy. Journal of Physics: Conference Series, 437, 2013 #012017. doi:10.1088/1742-6596/437/1/012017.
[3] Y. Friedman, "Digitization of the Harmonic Oscillator in Extended Relativity," Physica Scripta 87(6), 2013 #065702. doi:10.1088/0031-8949/87/06/065702.
Permanent Citation