Call Graphs of Nestedly Recursive Functions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
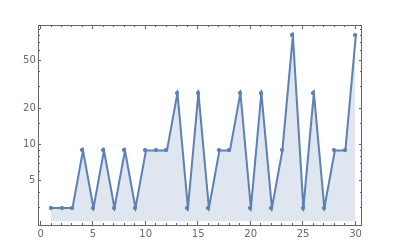
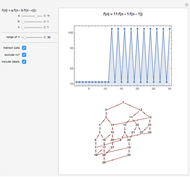
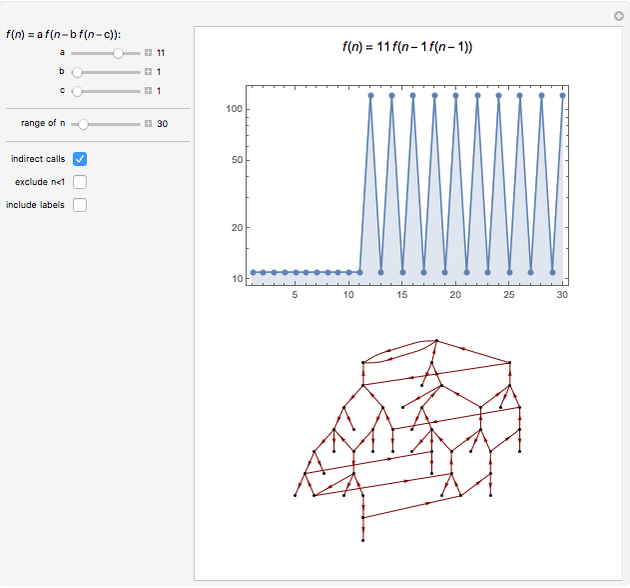
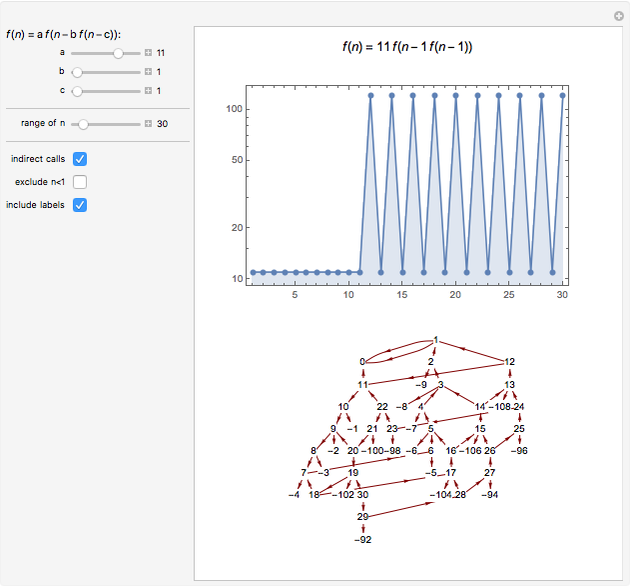
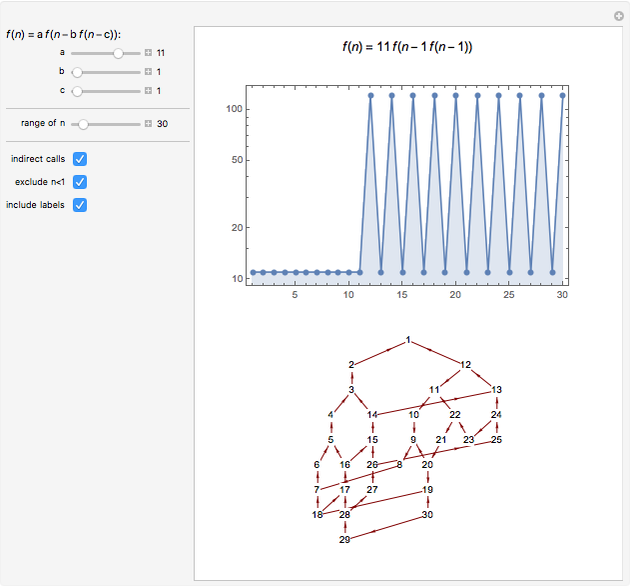
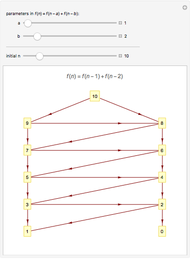
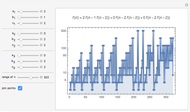
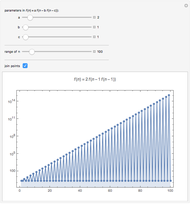
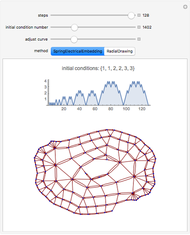
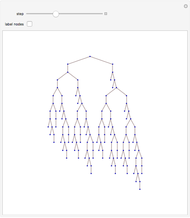
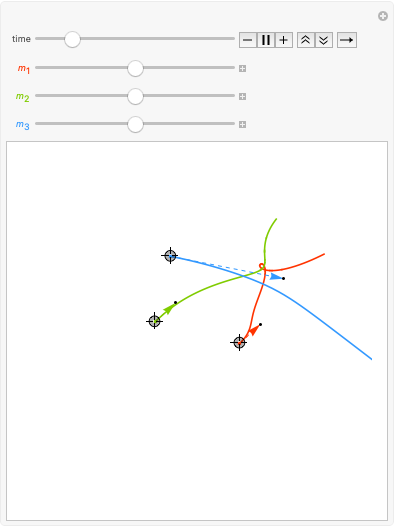
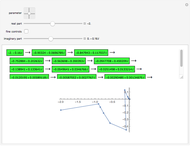
Nestedly recursive functions nestedly "call" previous instances of themselves. Even very simple recursion relations can lead to a complex sequence of values for nestedly recursive functions.
Contributed by: Stephen Wolfram (March 2011)
Based on: A live experiment at the 2003 NKS Summer School
Open content licensed under CC BY-NC-SA
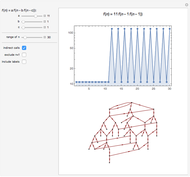
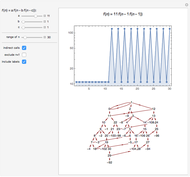
Snapshots
Details
The recursion relations are set up so that whenever they sample below n=1, the f[n] is taken to have value 1.
f[n]=3 f[n - f[n - 1]] is the simplest example that seems to yield complex behavior.
Functions like these were mentioned in A New Kind of Science, but first studied in detail in Stephen Wolfram's Live Experiment at the opening of the first NKS Summer School, in June 2003.
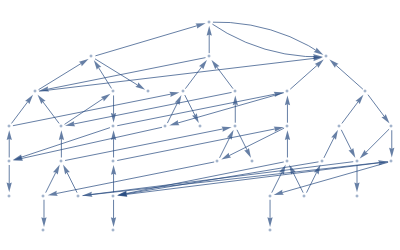
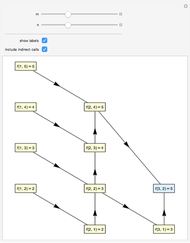
"Indirect calls" are instances of the "inner f" in the recursion relation.
Permanent Citation