Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

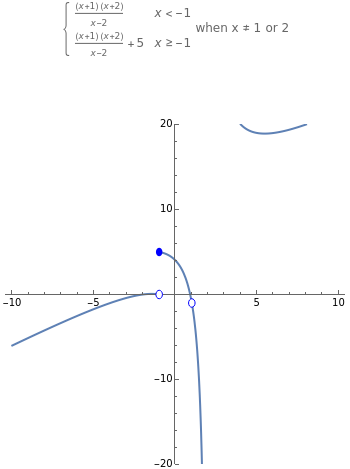
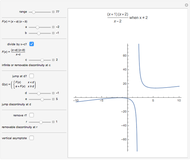
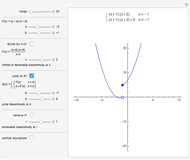
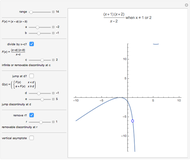
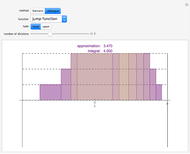
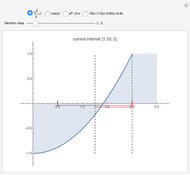
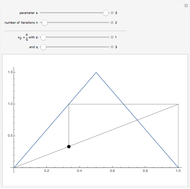
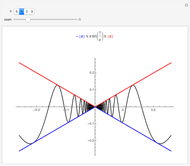
Discontinuity

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

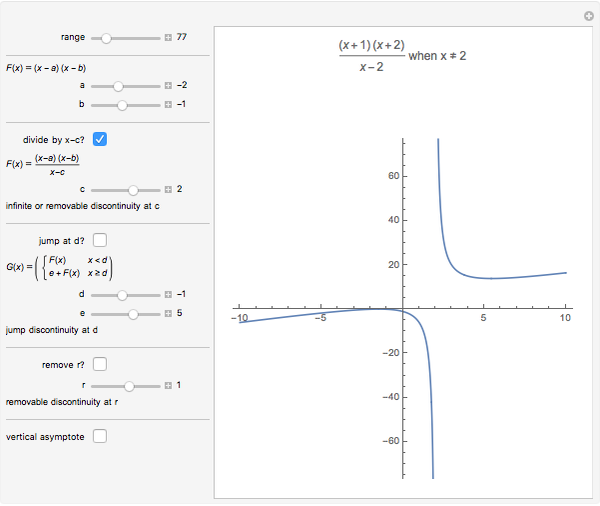
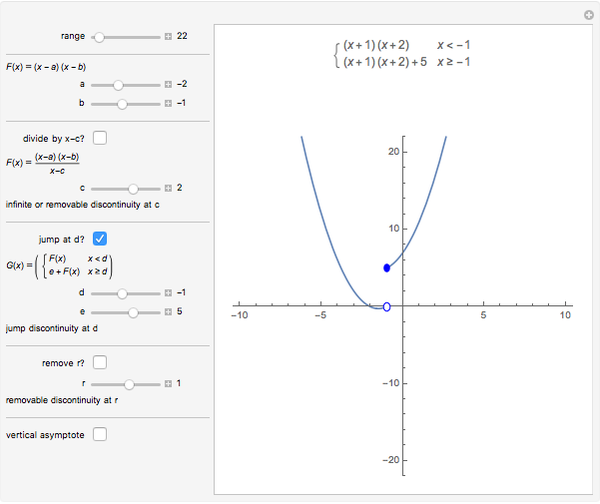
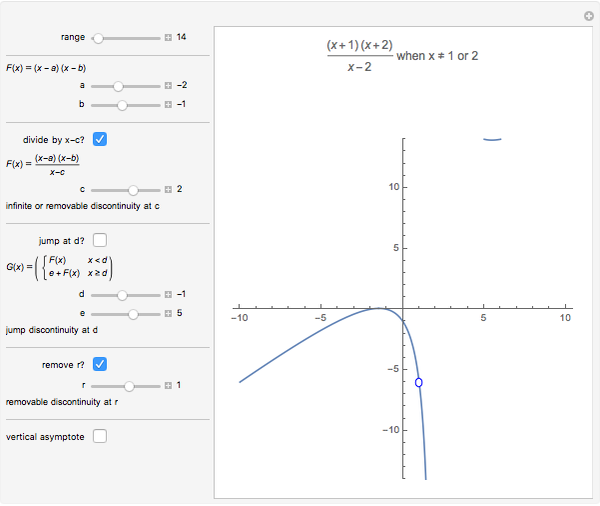
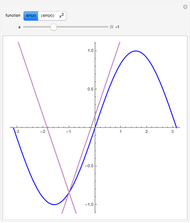
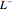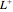
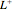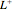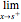
For a value , let = (the limit from the left) and = 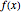(the limit from the right).

[more]

If = = , the function is continuous at .

If = ≠ , the function has a removable discontinuity at .

If ≠ , and both values are finite, the function has a jump discontinuity at .

If ≠ , and one or both values is infinite, the function has an infinite discontinuity at . This is also called an essential discontinuity.

[less]

Contributed by: Ed Pegg Jr (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

detailSectionParagraph
Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send